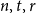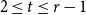In 1975 Bollobás, Erdős, and Szemerédi asked the following question: given positive integers $n, t, r$ with $2\le t\le r-1$ , what is the largest minimum degree $\delta (G)$ among all $r$ -partite graphs $G$ with parts of size $n$ and which do not contain a copy of 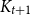$K_{t+1}$ ? The 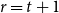$r=t+1$ case has attracted a lot of attention and was fully resolved by Haxell and Szabó, and Szabó and Tardos in 2006. In this article, we investigate the 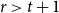$r\gt t+1$ case of the problem, which has remained dormant for over 40 years. We resolve the problem exactly in the case when 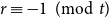$r \equiv -1 \pmod{t}$ , and up to an additive constant for many other cases, including when 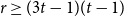$r \geq (3t-1)(t-1)$ . 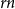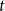Our approach utilizes a connection to the related problem of determining the maximum of the minimum degrees among the family of balanced $r$ -partite $rn$ -vertex graphs of chromatic number at most $t$ .