Let 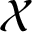H be a complex separable Hilbert space with $\dim H \geq 2$. Let $\mathcal {N}$ be a nest on H such that $E_+ \neq E$ for any $E \neq H, E \in \mathcal {N}$. We prove that every 2-local isometry of $\operatorname {Alg}\mathcal {N}$ is a surjective linear isometry.

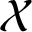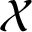Let $\mathcal {X}$ be a Banach space over the complex field $\mathbb {C}$ and $\mathcal {B(X)}$ be the algebra of all bounded linear operators on $\mathcal {X}$ . Let $\mathcal {N}$ be a nontrivial nest on $\mathcal {X}$ , 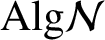$\text {Alg}\mathcal {N}$ be the nest algebra associated with $\mathcal {N}$ , and 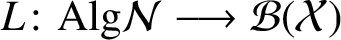$L\colon \text {Alg}\mathcal {N}\longrightarrow \mathcal {B(X)}$ be a linear mapping. Suppose that 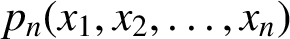$p_n(x_1,x_2,\ldots ,x_n)$ is an 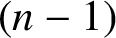$(n-1)\,$ th commutator defined by n indeterminates $x_1, x_2, \ldots , x_n$ . It is shown that L satisfies the rule

$$ \begin{align*}L(p_n(A_1, A_2, \ldots, A_n))=\sum_{k=1}^{n}p_n(A_1, \ldots, A_{k-1}, L(A_k), A_{k+1}, \ldots, A_n) \end{align*} $$

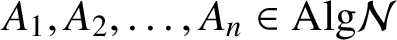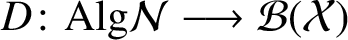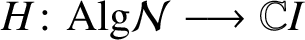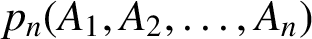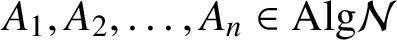for all $A_1, A_2, \ldots , A_n\in \text {Alg}\mathcal {N}$ if and only if there exist a linear derivation $D\colon \text {Alg}\mathcal {N}\longrightarrow \mathcal {B(X)}$ and a linear mapping $H\colon \text {Alg}\mathcal {N}\longrightarrow \mathbb {C}I$ vanishing on each $(n-1)\,$ th commutator $p_n(A_1,A_2,\ldots , A_n)$ for all $A_1, A_2, \ldots , A_n\in \text {Alg}\mathcal {N}$ such that 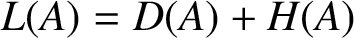$L(A)=D(A)+H(A)$ for all 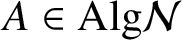$A\in \text {Alg}\mathcal {N}$ . We also propose some related topics for future research.

We show that Ringrose's diagonal ideals are primitive ideals in a nest algebra (subject to the continuum hypothesis). This answers an old question of Lance and provides for the first time concrete descriptions of enough primitive ideals to obtain the Jacobson radical as their intersection. Separately, we provide a standard form for all left ideals of a nest algebra, which leads to insights into the maximal left ideals. In the case of atomic nest algebras, we show how primitive ideals can be categorized by their behaviour on the diagonal and provide concrete examples of all types.

Triangular algebras, and maximal triangular algebras in particular, have been objects of interest for over 50 years. Rich families of examples have been studied in the context of many w*- and C*-algebras, but there remains a dearth of concrete examples in $B({\cal H})$. In previous work, we described a family of maximal triangular algebras of finite multiplicity. Here, we investigate a related family of maximal triangular algebras with infinite multiplicity, and unearth a new asymptotic structure exhibited by these algebras.