We study the large-time behaviour of the
nonlinear oscillator
\[
\hskip-20mm m\,x'' + f(x') + k\,x=0\,,
\] where m, k>0 and f is a monotone real function representing
nonlinear friction. We are interested in understanding the
long-time effect of a nonlinear damping term, with special
attention to the model case $f(x')= A\,|x'|^{\alpha-1}x'$
where m, k>0 and f is a monotone real function representing
nonlinear friction. We are interested in understanding the
long-time effect of a nonlinear damping term, with special
attention to the model case $f(x')= A\,|x'|^{\alpha-1}x'$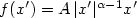 with
α real, A>0. We characterize the existence and behaviour
of fast orbits, i.e., orbits that stop in finite time.
with
α real, A>0. We characterize the existence and behaviour
of fast orbits, i.e., orbits that stop in finite time.