Zhou and Feng [‘On symmetric graphs of valency five’, Discrete Math. 310 (2010), 1725–1732] proved that all connected pentavalent 1-transitive Cayley graphs of finite nonabelian simple groups are normal. We construct an example of a nonnormal 2-arc transitive pentavalent symmetric Cayley graph on the alternating group 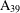 $\text{A}_{39}$. Furthermore, we show that the full automorphism group of this graph is isomorphic to the alternating group
$\text{A}_{39}$. Furthermore, we show that the full automorphism group of this graph is isomorphic to the alternating group  $\text{A}_{40}$.
$\text{A}_{40}$.
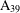 $\text{A}_{39}$
$\text{A}_{39}$