We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Let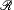 
be an o-minimal structure over a real closed field R. Given a simplicial complex K and some definable subsets S 1, …, Sl of its realization ∣K∣ in R we prove that there exist a subdivision K′ of K and a definable triangulation φ′: ∣K′∣ → ∣K∣ of ∣K∣ partitioning S 1, …, Sl with φ′ definably homotopic to id ∣K∣. As an application of this result we obtain the semialgebraic Hauptvermutung.
