In this paper, we study the continuity of rational functions realized by Büchi finite state transducers. It has been shown by Prieur that it can be decided whether such a function is continuous. We prove here that surprisingly, it cannot be decided whether such a function f has at least one point of continuity and that its continuity set C(f) cannot be computed. In the case of a synchronous rational function, we show that its continuity set is rational and that it can be computed. Furthermore we prove that any rational ${\bf \Pi}^0_2$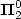-subset of Σω for some alphabet Σ is the continuity set C(f) of an ω-rational synchronous function f defined on Σω.