In this paper, by using the theory of reproducing kernel Hilbert spaces and the pair correlation formula constructed by Chandee et al. [‘Simple zeros of primitive Dirichlet 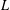 $L$-functions and the asymptotic large sieve’, Q. J. Math.65(1) (2014), 63–87], we prove that at least 93.22% of low-lying zeros of primitive Dirichlet
$L$-functions and the asymptotic large sieve’, Q. J. Math.65(1) (2014), 63–87], we prove that at least 93.22% of low-lying zeros of primitive Dirichlet 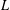 $L$-functions are simple in a proper sense, under the assumption of the generalised Riemann hypothesis.
$L$-functions are simple in a proper sense, under the assumption of the generalised Riemann hypothesis.
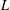 $L$-FUNCTIONS
$L$-FUNCTIONS