We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We prove a new energy or Caccioppoli type estimate for minimisers of the model functional ∫Ω|Du|2 + (det Du)2, where Ω ⊂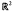 
2 and u: Ω → 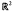2. We apply this to establish C∞ regularity for minimisers except on a closed set of measure zero. We also prove a maximum principle and use this to establish everywhere continuity of minimisers.
