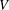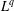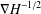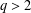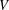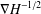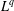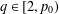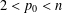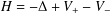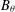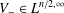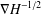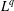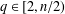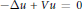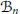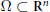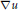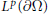Let $H=-\unicode[STIX]{x1D6E5}+V$ be a Schrödinger operator with some general signed potential $V$. This paper is mainly devoted to establishing the $L^{q}$-boundedness of the Riesz transform $\unicode[STIX]{x1D6FB}H^{-1/2}$ for $q>2$. We mainly prove that under certain conditions on $V$, the Riesz transform $\unicode[STIX]{x1D6FB}H^{-1/2}$ is bounded on $L^{q}$ for all $q\in [2,p_{0})$ with a given $2<p_{0}<n$. As an application, the main result can be applied to the operator $H=-\unicode[STIX]{x1D6E5}+V_{+}-V_{-}$, where $V_{+}$ belongs to the reverse Hölder class $B_{\unicode[STIX]{x1D703}}$ and $V_{-}\in L^{n/2,\infty }$ with a small norm. In particular, if $V_{-}=-\unicode[STIX]{x1D6FE}|x|^{-2}$ for some positive number $\unicode[STIX]{x1D6FE}$, $\unicode[STIX]{x1D6FB}H^{-1/2}$ is bounded on $L^{q}$ for all $q\in [2,n/2)$ and $n>4$.

We consider the Neumann problem for the Schrödinger equations 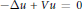$-\Delta u\,+\,Vu\,=\,0$, with singular nonnegative potentials $V$ belonging to the reverse Hölder class 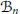${{\mathcal{B}}_{n}}$ , in a connected Lipschitz domain 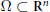$\Omega \,\subset \,{{\text{R}}^{n}}$ . Given boundary data $g$ in ${{H}^{p}}\text{or}\,{{L}^{p}}\,\text{for}\,\text{1}-\in \,<\,p\,\le \,2,\text{where}\,\text{0}<\in <\frac{1}{n}$ , it is shown that there is a unique solution, $u$, that solves the Neumann problem for the given data and such that the nontangential maximal function of 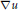$\nabla u$ is in 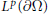${{L}^{p}}(\partial \Omega )$. Moreover, the uniform estimates are found.