We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Let {X
n
, n = 0, 1,…} be the sequence of the lower records for an arbitrary underlying distribution μ on [0, ∞). We show that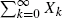 
is equal in distribution to 
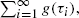where {τ
i
, i = 1, 2,…} is a Poisson flow of unit intensity and g is a right-continuous and nonincreasing function defined by μ. This observation allows us to extend results of Bose et al. and simplify their proofs.
