1 results
Numerical Semigroups That Are Not Intersections of d-Squashed Semigroups
-
- Journal:
- Canadian Mathematical Bulletin / Volume 52 / Issue 4 / 01 December 2009
- Published online by Cambridge University Press:
- 20 November 2018, pp. 598-612
- Print publication:
- 01 December 2009
-
- Article
-
- You have access
- Export citation
-
We say that a numerical semigroup is
$d$-squashed if it can be written in the form
$$S\,=\,\frac{1}{N}\langle {{a}_{1}},\,.\,.\,.\,,\,{{a}_{d}}\rangle \,\cap \,\mathbb{Z}$$for
$N$, 
${{a}_{1}}\,,\,.\,.\,.\,,\,{{a}_{d}}$ positive integers with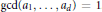 
$\gcd \left( {{a}_{1}},\,.\,.\,.\,,{{a}_{d}} \right)\,=\,1$. Rosales and Urbano have shown that a numerical semigroup is 2-squashed if and only if it is proportionally modular.Recent works by Rosales et al. give a concrete example of a numerical semigroup that cannot be written as an intersection of 2-squashed semigroups. We will show the existence of infinitely many numerical semigroups that cannot be written as an intersection of 2-squashed semigroups. We also will prove the same result for 3-squashed semigroups. We conjecture that there are numerical semigroups that cannot be written as the intersection of
$d$-squashed semigroups for any fixed $d$, and we prove some partial results towards this conjecture.
