We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We extend Goldie's (1991) implicit renewal theorem to enable the analysis of recursions on weighted branching trees. We illustrate the developed method by deriving the power-tail asymptotics of the distributions of the solutions R to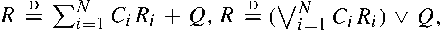 
and similar recursions, where (Q, N, C1, C2,…) is a nonnegative random vector with N ∈ {0, 1, 2, 3,…} ∪ {∞}, and 
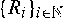are independent and identically distributed copies of R, independent of (Q, N, C1, C2,…); here ‘∨’ denotes the maximum operator.
