We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We prove the consistency of
with 
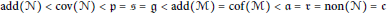$$~~\text{add}\left( \mathcal{N} \right)<\operatorname{cov}\left( \mathcal{N} \right)<\mathfrak{p}\text{=}\mathfrak{s}\text{=}\mathfrak{g}< \text{add}\left( \mathcal{M} \right)=\text{cof}\left( \mathcal{M} \right)<\mathfrak{a}=\mathfrak{r}=\text{non}\left( N \right)=\mathfrak{c}$$
$\text{ZFC}$, where each of these cardinal invariants assume arbitrary uncountable regular values.
