Every topological group G has, up to isomorphism, a unique minimal G-flow that maps onto every minimal G-flow, the universal minimal flow
 $M(G).$
We show that if G has a compact normal subgroup K that acts freely on
$M(G).$
We show that if G has a compact normal subgroup K that acts freely on
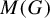 $M(G)$
and there exists a uniformly continuous cross-section from
$M(G)$
and there exists a uniformly continuous cross-section from
 $G/K$
to
$G/K$
to
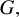 $G,$
then the phase space of
$G,$
then the phase space of
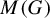 $M(G)$
is homeomorphic to the product of the phase space of
$M(G)$
is homeomorphic to the product of the phase space of
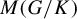 $M(G/K)$
with K. Moreover, if either the left and right uniformities on G coincide or G is isomorphic to a semidirect product
$M(G/K)$
with K. Moreover, if either the left and right uniformities on G coincide or G is isomorphic to a semidirect product
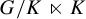 $G/K\ltimes K$
, we also recover the action, in the latter case extending a result of Kechris and Sokić. As an application, we show that the phase space of
$G/K\ltimes K$
, we also recover the action, in the latter case extending a result of Kechris and Sokić. As an application, we show that the phase space of
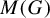 $M(G)$
for any totally disconnected locally compact Polish group G with a normal open compact subgroup is homeomorphic to a finite set, the Cantor set
$M(G)$
for any totally disconnected locally compact Polish group G with a normal open compact subgroup is homeomorphic to a finite set, the Cantor set
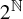 $2^{\mathbb {N}}$
,
$2^{\mathbb {N}}$
,
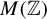 $M(\mathbb {Z})$
, or
$M(\mathbb {Z})$
, or
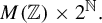 $M(\mathbb {Z})\times 2^{\mathbb {N}}.$
$M(\mathbb {Z})\times 2^{\mathbb {N}}.$