3 results
VANISHING COEFFICIENTS IN QUOTIENTS OF THETA FUNCTIONS OF MODULUS FIVE
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 102 / Issue 3 / December 2020
- Published online by Cambridge University Press:
- 27 March 2020, pp. 387-398
- Print publication:
- December 2020
-
- Article
- Export citation
VANISHING COEFFICIENTS IN FOUR QUOTIENTS OF INFINITE PRODUCT EXPANSIONS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 100 / Issue 2 / October 2019
- Published online by Cambridge University Press:
- 20 March 2019, pp. 216-224
- Print publication:
- October 2019
-
- Article
-
- You have access
- Export citation
-
Motivated by Ramanujan’s continued fraction and the work of Richmond and Szekeres [‘The Taylor coefficients of certain infinite products’, Acta Sci. Math. (Szeged)40(3–4) (1978), 347–369], we investigate vanishing coefficients along arithmetic progressions in four quotients of infinite product expansions and obtain similar results. For example,
$a_{1}(5n+4)=0$, where 
$a_{1}(n)$ is defined by 
$$\begin{eqnarray}\displaystyle {\displaystyle \frac{(q,q^{4};q^{5})_{\infty }^{3}}{(q^{2},q^{3};q^{5})_{\infty }^{2}}}=\mathop{\sum }_{n=0}^{\infty }a_{1}(n)q^{n}. & & \displaystyle \nonumber\end{eqnarray}$$
FURTHER RESULTS ON VANISHING COEFFICIENTS IN INFINITE PRODUCT EXPANSIONS
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society / Volume 98 / Issue 1 / February 2015
- Published online by Cambridge University Press:
- 11 November 2014, pp. 69-77
- Print publication:
- February 2015
-
- Article
-
- You have access
- Export citation
-
We extend results of Andrews and Bressoud [‘Vanishing coefficients in infinite product expansions’, J. Aust. Math. Soc. Ser. A27(2) (1979), 199–202] on the vanishing of coefficients in the series expansions of certain infinite products. These results have the form that if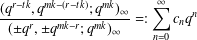
for certain integers
$$\begin{eqnarray}\frac{(q^{r-tk},q^{mk-(r-tk)};q^{mk})_{\infty }}{(\pm q^{r},\pm q^{mk-r};q^{mk})_{\infty }}=:\mathop{\sum }_{n=0}^{\infty }c_{n}q^{n}\end{eqnarray}$$
$k$, 
$m$, 
$s$ and 
$t$, where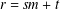 
$r=sm+t$, then 
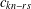$c_{kn-rs}$ is always zero. Our theorems also partly give a simpler reformulation of results of Alladi and Gordon [‘Vanishing coefficients in the expansion of products of Rogers–Ramanujan type’, in: The Rademacher Legacy to Mathematics (University Park, PA, 1992), Contemporary Mathematics, 166 (American Mathematical Society, Providence, RI, 1994), 129–139], but also give results for cases not covered by the theorems of Alladi and Gordon. We also give some interpretations of the analytic results in terms of integer partitions.
