Published online by Cambridge University Press: 12 September 2023
Turbulent wakes are often characterized by dominant coherent structures over disparate scales. Dynamics of their behaviour can be attributed to interscale energy dynamics and triadic interactions. We develop a methodology to quantify the dynamics of kinetic energy of specific scales. Coherent motions are characterized by the triple decomposition and used to define mean, coherent and random velocity. Specific scales of coherent structures are identified through dynamic mode decomposition, whereby the total coherent velocity is separated into a set of velocities classified by frequency. The coherent kinetic energy of a specific scale is defined by a frequency triad of scale-specific velocities. Equations for the balance of scale-specific coherent kinetic energy are derived to interpret interscale dynamics. The methodology is demonstrated on three wake flows: (i)  ${Re}=175$ flow over a cylinder; (ii) a direct numerical simulation of
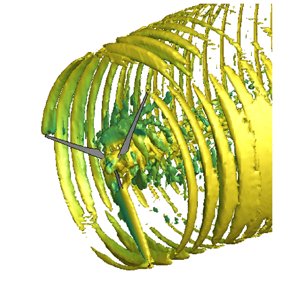
${Re}=175$ flow over a cylinder; (ii) a direct numerical simulation of  ${Re}=3900$ flow over a cylinder; and (iii) a large-eddy simulation of a utility-scale wind turbine. The cylinder wake cases show that energy transfer starts with vortex shedding and redistributes energy through resonance of higher harmonics. The scale-specific coherent kinetic energy balance quantifies the distribution of transport and transfer among coherent, mean and random scales. The coherent kinetic energy in the rotor scales and the hub vortex scale in the wind turbine interact to produce new scales. The analysis reveals that vortices at the blade root interact with the hub vortex formed behind the nacelle, which has implications for the proliferation of scales in the downwind near wake.
${Re}=3900$ flow over a cylinder; and (iii) a large-eddy simulation of a utility-scale wind turbine. The cylinder wake cases show that energy transfer starts with vortex shedding and redistributes energy through resonance of higher harmonics. The scale-specific coherent kinetic energy balance quantifies the distribution of transport and transfer among coherent, mean and random scales. The coherent kinetic energy in the rotor scales and the hub vortex scale in the wind turbine interact to produce new scales. The analysis reveals that vortices at the blade root interact with the hub vortex formed behind the nacelle, which has implications for the proliferation of scales in the downwind near wake.