Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Yang, Di
Shen, Lian
and
Meneveau, Charles
2013.
An Assessment of Dynamic Subgrid-Scale Sea-Surface Roughness Models.
Flow, Turbulence and Combustion,
Vol. 91,
Issue. 3,
p.
541.
Yang, Di
Meneveau, Charles
and
Shen, Lian
2014.
Large-eddy simulation of offshore wind farm.
Physics of Fluids,
Vol. 26,
Issue. 2,
Yang, Di
Meneveau, Charles
and
Shen, Lian
2014.
Effect of downwind swells on offshore wind energy harvesting – A large-eddy simulation study.
Renewable Energy,
Vol. 70,
Issue. ,
p.
11.
Sullivan, Peter P.
McWilliams, James C.
and
Patton, Edward G.
2014.
Large-Eddy Simulation of Marine Atmospheric Boundary Layers above a Spectrum of Moving Waves.
Journal of the Atmospheric Sciences,
Vol. 71,
Issue. 11,
p.
4001.
Chan, L.
MacDonald, M.
Chung, D.
Hutchins, N.
and
Ooi, A.
2015.
A systematic investigation of roughness height and wavelength in turbulent pipe flow in the transitionally rough regime.
Journal of Fluid Mechanics,
Vol. 771,
Issue. ,
p.
743.
Melville, W. Kendall
and
Fedorov, Alexey V.
2015.
The equilibrium dynamics and statistics of gravity–capillary waves.
Journal of Fluid Mechanics,
Vol. 767,
Issue. ,
p.
449.
Kudryavtsev, Vladimir
and
Chapron, Bertrand
2016.
On Growth Rate of Wind Waves: Impact of Short-Scale Breaking Modulations.
Journal of Physical Oceanography,
Vol. 46,
Issue. 1,
p.
349.
Xie, Shengbai
Yang, Di
Liu, Yi
and
Shen, Lian
2016.
Simulation-based study of wind loads on semi-submersed object in ocean wave field.
Physics of Fluids,
Vol. 28,
Issue. 1,
Jiang, Qingfang
Sullivan, Peter
Wang, Shouping
Doyle, James
and
Vincent, Linwood
2016.
Impact of Swell on Air–Sea Momentum Flux and Marine Boundary Layer under Low-Wind Conditions.
Journal of the Atmospheric Sciences,
Vol. 73,
Issue. 7,
p.
2683.
Resio, Donald T.
Vincent, Linwood
and
Ardag, Dorukhan
2016.
Characteristics of directional wave spectra and implications for detailed-balance wave modeling.
Ocean Modelling,
Vol. 103,
Issue. ,
p.
38.
Zavadsky, Andrey
and
Shemer, Lev
2017.
Water waves excited by near-impulsive wind forcing.
Journal of Fluid Mechanics,
Vol. 828,
Issue. ,
p.
459.
Allaerts, Dries
and
Meyers, Johan
2017.
Boundary-layer development and gravity waves in conventionally neutral wind farms.
Journal of Fluid Mechanics,
Vol. 814,
Issue. ,
p.
95.
Yang, Di
and
Shen, Lian
2017.
Direct numerical simulation of scalar transport in turbulent flows over progressive surface waves.
Journal of Fluid Mechanics,
Vol. 819,
Issue. ,
p.
58.
Young, I.R.
and
Donelan, M.A.
2018.
On the determination of global ocean wind and wave climate from satellite observations.
Remote Sensing of Environment,
Vol. 215,
Issue. ,
p.
228.
Grare, Laurent
Lenain, Luc
and
Melville, W. Kendall
2018.
Vertical Profiles of the Wave-Induced Airflow above Ocean Surface Waves.
Journal of Physical Oceanography,
Vol. 48,
Issue. 12,
p.
2901.
Donelan, Mark A.
2018.
On the Decrease of the Oceanic Drag Coefficient in High Winds.
Journal of Geophysical Research: Oceans,
Vol. 123,
Issue. 2,
p.
1485.
Li, Qi
Bou-Zeid, Elie
Vercauteren, Nikki
and
Parlange, Marc
2018.
Signatures of Air–Wave Interactions Over a Large Lake.
Boundary-Layer Meteorology,
Vol. 167,
Issue. 3,
p.
445.
Wang, Qing
Alappattu, Denny P.
Billingsley, Stephanie
Blomquist, Byron
Burkholder, Robert J.
Christman, Adam J.
Creegan, Edward D.
de Paolo, Tony
Eleuterio, Daniel P.
Fernando, Harindra Joseph S.
Franklin, Kyle B.
Grachev, Andrey A.
Haack, Tracy
Hanley, Thomas R.
Hocut, Christopher M.
Holt, Teddy R.
Horgan, Kate
Jonsson, Haflidi H.
Hale, Robert A.
Kalogiros, John A.
Khelif, Djamal
Leo, Laura S.
Lind, Richard J.
Lozovatsky, Iossif
Planella-Morato, Jesus
Mukherjee, Swagato
Nuss, Wendell A.
Pozderac, Jonathan
Ted Rogers, L.
Savelyev, Ivan
Savidge, Dana K.
Kipp Shearman, R.
Shen, Lian
Terrill, Eric
Marcela Ulate, A.
Wang, Qi
Travis Wendt, R.
Wiss, Russell
Woods, Roy K.
Xu, Luyao
Yamaguchi, Ryan T.
and
Yardim, Caglar
2018.
CASPER: Coupled Air–Sea Processes and Electromagnetic Ducting Research.
Bulletin of the American Meteorological Society,
Vol. 99,
Issue. 7,
p.
1449.
Calderer, Antoni
Guo, Xin
Shen, Lian
and
Sotiropoulos, Fotis
2018.
Fluid–structure interaction simulation of floating structures interacting with complex, large-scale ocean waves and atmospheric turbulence with application to floating offshore wind turbines.
Journal of Computational Physics,
Vol. 355,
Issue. ,
p.
144.
Xiao, Shuolin
and
Yang, Di
2019.
Large-Eddy Simulation-Based Study of Effect of Swell-Induced Pitch Motion on Wake-Flow Statistics and Power Extraction of Offshore Wind Turbines.
Energies,
Vol. 12,
Issue. 7,
p.
1246.
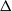 $\Delta $, while the effects of subgrid-scale (SGS) waves on the wind need to be modelled. Usually, a surface-layer model based on the law of the wall is used; but the surface roughness has been known to depend on the local wind and wave conditions and is difficult to parameterize. In this study, a dynamic model for the SGS sea-surface roughness is developed, with the roughness corresponding to the SGS waves expressed as
$\Delta $, while the effects of subgrid-scale (SGS) waves on the wind need to be modelled. Usually, a surface-layer model based on the law of the wall is used; but the surface roughness has been known to depend on the local wind and wave conditions and is difficult to parameterize. In this study, a dynamic model for the SGS sea-surface roughness is developed, with the roughness corresponding to the SGS waves expressed as  ${\alpha }_{w} \hspace{0.167em} { \sigma }_{\eta }^{\Delta } $. Here,
${\alpha }_{w} \hspace{0.167em} { \sigma }_{\eta }^{\Delta } $. Here, 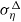 ${ \sigma }_{\eta }^{\Delta } $ is the effective amplitude of the SGS waves, modelled as a weighted integral of the SGS wave spectrum based on the geometric and kinematic properties of the waves for which five candidate expressions are examined. Moreover,
${ \sigma }_{\eta }^{\Delta } $ is the effective amplitude of the SGS waves, modelled as a weighted integral of the SGS wave spectrum based on the geometric and kinematic properties of the waves for which five candidate expressions are examined. Moreover, 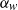 ${\alpha }_{w} $ is an unknown dimensionless model coefficient determined dynamically based on the first-principles constraint that the total surface drag force or average surface stress must be independent of the LES filter scale
${\alpha }_{w} $ is an unknown dimensionless model coefficient determined dynamically based on the first-principles constraint that the total surface drag force or average surface stress must be independent of the LES filter scale  $\Delta $. The feasibility and consistency of the dynamic sea-surface roughness models are assessed by a priori tests using data from high-resolution LES with near-surface resolution, appropriately filtered. Also, these data are used for a posteriori tests of the dynamic sea-surface roughness models in LES with near-surface modelling. It is found that the dynamic modelling approach can successfully capture the effects of SGS waves on the wind turbulence without ad hoc prescription of the model parameter
$\Delta $. The feasibility and consistency of the dynamic sea-surface roughness models are assessed by a priori tests using data from high-resolution LES with near-surface resolution, appropriately filtered. Also, these data are used for a posteriori tests of the dynamic sea-surface roughness models in LES with near-surface modelling. It is found that the dynamic modelling approach can successfully capture the effects of SGS waves on the wind turbulence without ad hoc prescription of the model parameter 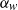 ${\alpha }_{w} $. Also, for
${\alpha }_{w} $. Also, for 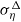 ${ \sigma }_{\eta }^{\Delta } $, a model based on the kinematics of wind–wave relative motion achieves the best performance among the five candidate models.
${ \sigma }_{\eta }^{\Delta } $, a model based on the kinematics of wind–wave relative motion achieves the best performance among the five candidate models.