In this paper I am going to set forth a formal system based on five inter-class relations. These relations exist respectively between a class of a's and a class of b's.

(i) if and only if every a is a b and every b is an a,

(ii) if and only if every a is a b and not every b is an a,

(iii) if and only if it is not the case that either every a is a b or every b is an a or no a is a b,

(iv) if and only if every b is an a and not every a is a b,

(v) if and only if no a is a b.

These relations between classes, which correspond, as will be seen, to the five relations between two circles a and b shown in the well-known Eulerian diagrams,

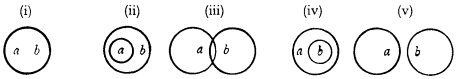
are of course connected in an intimate way with the four forms of proposition, A, E, I, O, of the traditional syllogistic logic. The French mathematician, J. D. Gergonne, seems to have been the first to recognize these relations explicitly and to understand their importance in syllogistic theory. It is therefore appropriate that they should be called by his name.

Gergonne first of all showed with reference to these relations what are the sufficient and necessary conditions of the truth of propositions of each of the four traditional forms: for example, an A proposition, ‘All a is b’ is true if and only if either the first or the second relation exists between the class of a's and the class of b's.

In certain applications of truth-functional logic it is of interest to determine classes of formulas equivalent to a given formula Φ under the hypothesis that certain conjunctions of letters of Φ are always false. Of especial interest is the case where the class to be determined is that of simplest normal truth functions. The problem of giving a calculation procedure for this question is evidently a more general form of the simplification problem as considered in [1] and [2]. The purpose of this note is to indicate how the procedure of [1] applies.

Let Π, Π′, Π″, … be fundamental formulas, in the sense of [2], such that every literal of Π, etc., is a literal of Φ and such that Π, Π′, Π″, … are all false for all values of the constituent literals. Then we say that Ψ is a weak simplest disjunctive normal equivalent of Φ if and only if Ψ ≡ Φ, under the hypothesis, and there is no Ψ′ such that Ψ′ is simpler than Ψ and Ψ′ ≡ Φ, under the hypothesis. Similarly, under exactly the same hypothesis, we say that X is a weak simplest conjunctive normal equivalent of Φ. When the class of always false fundamental formulas is empty, as in [1] and [2], we may speak of strong simplest forms and hence of the more general problem of strong simplification.

In the following pages we wish to clarify some questions concerning definitions of relations in terms of other relations. A study is made of how a definition of a relation R1 in terms of another relation R2 works in a given specified universe U. The definition is regarded as a correlation rule between the “possible extensions” of R1 and R2. There are no difficulties in formulating necessary, and in a certain sense also sufficient, formal conditions for the existence of a definition relation between R1 and R2.

In particular, we are concerned with questions such as: “Is every relation of the form s1 definable in terms of some relation of the form s2?” (§ IV) A related question concerns the criterion used by Goodman1 for degrees of complexity, namely, whether for every relation R2 of the form s1 there is some relation R2 of the form s2 such that R1 and R2 are mutually definable (§ V). Some concrete results concerning this condition are stated in the theorems 22–24.

The “complexity function” which is defined (definition 17) and which very naturally suggests itself from the discussion of the definability conditions, seems to furnish an adequate measure of the sort of complexity discussed by Goodman.

The purpose of this paper is to analyze rational betting. In particular, we concentrate on one necessary feature of rational betting, the avoidance of certainty of losing to a clever opponent. If a bettor is quite foolish in his choice of the rates at which he will bet, ah opponent can win money from him no matter what happens.

This phenomenon is well known to professional bettors—especially bookmakers, who must as a matter of practical necessity avoid its occurrence. Such a losing book is called by them a “dutch book.” Our investigations are thus concerned with necessary and sufficient conditions that a book not be “dutch.”

De Finetti [3] has started with the same idea and used it as a foundation for the theory of probability. It is our aim to consider the same subject more precisely and attempt to answer some questions about desirable features of a confirmation function. We wish to connect the ideas of De Finetti with those of Carnap [1] and Hossiasson-Lindenbaum [6]. The results expressed by Theorem 1 are essentially contained in De Finetti's work. Theorems 3 and 4 seem to be new.

The confirmation functions which we consider will be functions with two sentences as arguments taking real numbers as values. Intuitively, C(h, e) will represent the rate at which a bettor would be willing to bet on the hypothesis h if he knew the information expressed by the sentence e, the evidence.

The question of what constitutes fairness in betting quotients has been studied by Ramsey, deFinetti, and Shimony. Thanks to their combined efforts we now have a satisfactory definition of fairness.

On the other hand, the explication of the concept of degree of confirmation (inductive probability) has progressed rapidly in recent years, thanks primarily to Carnap. This explication has usually proceeded by laying down the axioms for frequency-probabilities, and elaborating on these. While in the case where a frequency interpretation is intended these axioms are clearly justified, in our case they have been laid down without any justification. Carnap's presentation has been criticized for just this reason.

The purpose of this paper is to show that the probability axioms are necessary and sufficient conditions to assure that the degrees of confirmation form a set of fair betting quotients. In addition it will be shown that one additional, highly controversial, axiom is precisely the condition needed to assure that not only deFinetti's weaker criterion but Shimony's criterion of fairness is also satisfied.