Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Chunikhin, S. A.
and
Shemetkov, L. A.
1973.
Finite groups.
Journal of Soviet Mathematics,
Vol. 1,
Issue. 3,
p.
291.
Mann, Avinoam
1978.
Conjugacy classes in finite groups.
Israel Journal of Mathematics,
Vol. 31,
Issue. 1,
p.
78.
López, Antonio Vera
1984.
Conjugacy classes in finite solvable groups.
Israel Journal of Mathematics,
Vol. 47,
Issue. 2-3,
p.
241.
López, Antonio Vera
1987.
Conjugacy classes in finite groups.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 105,
Issue. 1,
p.
259.
López, Antonio Vera
and
de Elguea, Lourdes Ortiz
1988.
On the number of conjugacy classes in a finite group.
Journal of Algebra,
Vol. 115,
Issue. 1,
p.
46.
Vera-López, Antonio
and
Larrea, MA Concepción
1988.
On the number of conjugacy classes in a finite group II.
Israel Journal of Mathematics,
Vol. 64,
Issue. 1,
p.
87.
Vera-L�pez, Antonio
and
Concepci�n Larrea, Ma
1989.
On the number of conjugacy classes in a finitep-group.
Archiv der Mathematik,
Vol. 53,
Issue. 2,
p.
126.
Vera-López, Antonio
and
Larrea, Begoña
1991.
On p-groups of maximal class.
Journal of Algebra,
Vol. 137,
Issue. 1,
p.
77.
Vera-López, Antonio
and
Fernández-Alcober, Gustavo A
1991.
On p-groups of maximal class, II.
Journal of Algebra,
Vol. 143,
Issue. 1,
p.
179.
Vera-López, Antonio
and
Fernández-Alcober, Gustavo A.
1994.
The conjugacy vector of ap-group of maximal class.
Israel Journal of Mathematics,
Vol. 86,
Issue. 1-3,
p.
233.
Shalev, A.
1995.
Finite and Locally Finite Groups.
p.
401.
Poland, John
and
Rhemtulla, Akbar
1996.
The number of conjugacy classes of non-normal subgroups in nilpotent groups.
Communications in Algebra,
Vol. 24,
Issue. 10,
p.
3237.
Fulman, Jason
1996.
Divisibility results on the number of conjugacy classes in finite groups.
Archiv der Mathematik,
Vol. 67,
Issue. 1,
p.
11.
1998.
Character Theory of Finite Groups.
p.
607.
Pálfy, P. P.
1998.
The number of conjugacy classes in some quotients of the Nottingham group.
Proceedings of the Edinburgh Mathematical Society,
Vol. 41,
Issue. 2,
p.
369.
Fernández-Alcober, Gustavo A.
and
Shepherd, Raymond T.
1998.
On the Order ofp-Groups of Abundance Zero.
Journal of Algebra,
Vol. 201,
Issue. 2,
p.
392.
Longobardi, Patrizia
Maj, Mercede
and
Mann, Avinoam
1999.
Minimal classes and maximal class inp-groups.
Israel Journal of Mathematics,
Vol. 110,
Issue. 1,
p.
93.
Mann, A.
1999.
Minimal characters of p-groups.
Journal of Group Theory,
Vol. 2,
Issue. 3,
Jaikin-Zapirain, A.
2000.
On the abundance of finite p-groups.
Journal of Group Theory,
Vol. 3,
Issue. 3,
Boston, Nigel
and
Walker, Judy L.
2000.
2-groups with few conjugacy classes.
Proceedings of the Edinburgh Mathematical Society,
Vol. 43,
Issue. 1,
p.
211.
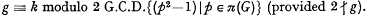 By the same methods we prove g ≡ k modulo G.C.D. {(p–1)2 p ∈ π(G)} and that if G is a p-group, g = h modulo (p−1)(p2−1). It follows that k has the form (n+r(p−1)) (p2−1)+pe where r and n are integers ≧ 0, p is a prime, e is 0 or 1, and g = p2n+e. This has been established using representation theory by Philip Hall [3] (see also [5]). If
By the same methods we prove g ≡ k modulo G.C.D. {(p–1)2 p ∈ π(G)} and that if G is a p-group, g = h modulo (p−1)(p2−1). It follows that k has the form (n+r(p−1)) (p2−1)+pe where r and n are integers ≧ 0, p is a prime, e is 0 or 1, and g = p2n+e. This has been established using representation theory by Philip Hall [3] (see also [5]). If 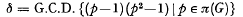 then simple examples show (for 6 ∤ g obviously) that g ≡ k modulo σ or even σ/2 is not generally true.
then simple examples show (for 6 ∤ g obviously) that g ≡ k modulo σ or even σ/2 is not generally true.