Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Grigorian, S.A.
and
Gumerov, R.N.
2006.
On the structure of finite coverings of compact connected groups.
Topology and its Applications,
Vol. 153,
Issue. 18,
p.
3598.
Çakallı, Hüseyin
2012.
Sequential definitions of connectedness.
Applied Mathematics Letters,
Vol. 25,
Issue. 3,
p.
461.
Quang, Nguyen Tien
Phung, Che Thi Kim
and
Tung, Ngo Sy
2013.
ABELIAN CROSSED MODULES AND STRICT PICARD CATEGORIES.
Albanian Journal of Mathematics,
Vol. 7,
Issue. 1,
Nguyen, Tien Quang
Pham, Thi Cuc
and
Nguyen, Thu Thuy
2014.
CROSSED MODULES AND STRICT GR-CATEGORIES.
Communications of the Korean Mathematical Society,
Vol. 29,
Issue. 1,
p.
9.
Quang, N. T.
Phung, C. T. K.
and
Cuc, P. T.
2014.
Braided equivariant crossed modules and cohomology of Γ-modules.
Indian Journal of Pure and Applied Mathematics,
Vol. 45,
Issue. 6,
p.
953.
CUC, PHAM THI
and
QUANG, NGUYEN TIEN
2015.
EQUIVARIANT CROSSED MODULES AND COHOMOLOGY OF GROUPS WITH OPERATORS.
Bulletin of the Korean Mathematical Society,
Vol. 52,
Issue. 4,
p.
1077.
Mucuk, Osman
Şahan, Tunçar
and
Alemdar, Nazmiye
2015.
Normality and Quotients in Crossed Modules and Group-groupoids.
Applied Categorical Structures,
Vol. 23,
Issue. 3,
p.
415.
Şahan, Tunçar
and
Erciyes, Ayhan
2018.
Actions of internal groupoids in the category of Leibniz algebras.
Communications Faculty Of Science University of Ankara Series A1Mathematics and Statistics,
Vol. 68,
Issue. 1,
p.
619.
Şahan, Tunçar
and
Mucuk, Osman
2019.
Normality and quotient of crossed modules within group with operations.
Vol. 2091,
Issue. ,
p.
030032.
Mucuk, Osman
and
Şahan, Tunçar
2019.
Group-groupoid actions and liftings of crossed modules.
Georgian Mathematical Journal,
Vol. 26,
Issue. 3,
p.
437.
Akız, H. Fulya
Mucuk, Osman
and
Şahan, Tunçar
2019.
Liftings of crossed modules in the category of groups with operations.
Boletim da Sociedade Paranaense de Matemática,
Vol. 38,
Issue. 7,
p.
181.
Alemdar, Nazmiye
2019.
Topolojik R-Modül Grupoid Örtüleri.
Süleyman Demirel Üniversitesi Fen Bilimleri Enstitüsü Dergisi,
Vol. 23,
Issue. 1,
p.
272.
Rumynin, Dmitriy
Vakhrameev, Demyan
and
Westaway, Matthew
2019.
Covering groups of nonconnected topological groups and 2-groups.
Communications in Algebra,
Vol. 47,
Issue. 12,
p.
5207.
Şahan, Tunçar
and
Mucuk, Osman
2019.
Normality and quotient in the category of crossed modules within the category of groups with operations.
Boletim da Sociedade Paranaense de Matemática,
Vol. 38,
Issue. 7,
p.
169.
Akız, H. Fulya
and
Mucuk, Osman
2019.
Topological aspect of monodromy groupoid for a topological internal groupoid.
Vol. 2183,
Issue. ,
p.
030005.
MUCUK, Osman
and
AKIZ, Hürmet Fulya
2020.
Covering morphisms of topological internal groupoids.
Hacettepe Journal of Mathematics and Statistics,
Vol. 49,
Issue. 3,
p.
1020.
DEMİR, Serap
and
MUCUK, Osman
2020.
Actions of Double Group-groupoids and Covering Morphism.
Gazi University Journal of Science,
Vol. 33,
Issue. 4,
p.
810.
TEMEL, Sedat
2020.
Internal Categories in Crossed Semimodules and Schreier Internal Categories.
Mathematical Sciences and Applications E-Notes,
Vol. 8,
Issue. 2,
p.
86.
Datuashvili, Tamar
Mucuk, Osman
and
Şahan, Tunçar
2020.
Groups up to congruence relation and from categorical groups to c-crossed modules.
Journal of Homotopy and Related Structures,
Vol. 15,
Issue. 3-4,
p.
625.
Mucuk, Osman
and
Çakallı, Hüseyin
2021.
G-compactness and local G-compactness of topological groups with operations.
Vol. 2334,
Issue. ,
p.
020007.
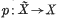 be the universal cover of the underlying space of X. It follows easily from classical properties of lifting maps to covering spaces that for any point ẽ in
be the universal cover of the underlying space of X. It follows easily from classical properties of lifting maps to covering spaces that for any point ẽ in 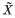 with pẽ = e there is a unique structure of topological group on
with pẽ = e there is a unique structure of topological group on 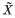 such that ẽ is the identity and
such that ẽ is the identity and 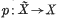 is a morphism of groups. We say that the structure of topological group on X lifts to
is a morphism of groups. We say that the structure of topological group on X lifts to 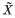 .
.