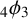A q-SUPERCONGRUENCE ARISING FROM ANDREWS’ $_4\phi _3$ IDENTITY

Abstract

We establish a q-analogue of a supercongruence related to a supercongruence of Rodriguez-Villegas, which extends a q-congruence of Guo and Zeng [‘Some q-analogues of supercongruences of Rodriguez-Villegas’, J. Number Theory 145 (2014), 301–316]. The important ingredients in the proof include Andrews’ $_4\phi _3$ terminating identity.

1. Introduction

In 2003, Rodriguez-Villegas [5] investigated hypergeometric families of Calabi–Yau manifolds. He observed numerically some remarkable supercongruences between the values of the truncated hypergeometric series and expressions derived from the number of $\mathbb {F}_p$ -points of the associated Calabi–Yau manifolds. For manifolds of dimension $d=1$ , he conjectured four interesting supercongruences associated to certain elliptic curves, one of which is

(1.1) $$ \begin{align} \sum_{k=0}^{p-1} \frac{{2k\choose k}^2}{16^k}\equiv (-1)^{({p-1)}/{2}} \pmod{p^2}, \end{align} $$

where $p\ge 5$ is a prime. The conjectural supercongruence (1.1) was first proved by Mortenson [4].

For polynomials $A_1(q), A_2(q),P(q)\in \mathbb {Z}[q]$ , the q-congruence

$$ \begin{align*}A_1(q)/A_2(q)\equiv 0\pmod{P(q)}\end{align*} $$

is understood as $A_1(q)$ is divisible by $P(q)$ , and $A_2(q)$ is coprime with $P(q)$ . In general, for rational functions $A(q),B(q)\in \mathbb {Q}(q)$ and polynomial $P(q)\in \mathbb {Z}[q]$ ,

$$ \begin{align*} A(q)\equiv B(q)\pmod{P(q)}\Longleftrightarrow A(q)-B(q)\equiv 0\pmod{P(q)}. \end{align*} $$

Guo and Zeng [3, Corollary 2.2] established a q-analogue of (1.1) as follows:

$$ \begin{align*} \sum_{k=0}^{p-1}\frac{(q;q^2)_k^2}{(q^2;q^2)_k^2}\equiv (-1)^{{(p-1)}/{2}}q^{{(1-p^2)}/{4}} \pmod{[p]^2}. \end{align*} $$

Here and in what follows, the q-analogue of the natural number n is defined by $[n]=(1-q^n)/(1-q)$ , and for $n\ge 1$ , the q-shifted factorial is defined by $(a;q)_n=(1-a)(1-aq)\cdots (1-aq^{n-1})$ with $(a;q)_0=1$ .

In 2011, Sun [7, Conjecture 5.5] conjectured a supercongruence related to (1.1): modulo $p^2$ ,

(1.2) $$ \begin{align} \sum_{k=0}^{(p-1)/2}\frac{{2k\choose k}^2}{32^k}\equiv \begin{cases} \displaystyle 2x-\frac{p}{2x}&\text{if}\ p\equiv 1\pmod{4} \text{ and}\ p=x^2+y^2 \text{ with}\ 4\mid (x-1),\\ 0 &\text{if}\ p\equiv 3\pmod{4}, \end{cases} \end{align} $$

which was proved by Tauraso [8] and Sun [6, Theorem 2.2].

Guo and Zeng [3, Corollary 2.7] established a partial q-analogue of (1.2):

(1.3) $$ \begin{align} \sum_{k=0}^{(p-1)/2}\frac{(q;q^2)_k^2}{(q^2;q^2)_k (q^4;q^4)_k}q^{2k}\equiv 0\pmod{[p]^2} \end{align} $$

for all primes $p\equiv 3\pmod {4}$ .

To continue the q-story of (1.2), we recall some q-series notation. The basic hypergeometric series is defined by

$$ \begin{align*} {}_{r+1}\phi_{r}\bigg[ \begin{matrix} a_1,a_2,\ldots,a_{r+1}\\[5pt] b_1,b_2,\ldots,b_r\end{matrix};q,z\bigg]= \sum_{k=0}^{\infty}\frac{(a_1,a_2,\ldots,a_{r+1};q)_k}{(q,b_1,b_2,\ldots,b_r;q)_k}z^k, \end{align*} $$

where $(a_1,a_2,\ldots ,a_{m};q)_k=(a_1;q)_k(a_2;q)_k\cdots (a_m;q)_k$ . The nth cyclotomic polynomial is given by

$$ \begin{align*} \Phi_n(q)=\prod_{\substack{1\le k \le n\\[3pt](n,k)=1}} (q-\zeta^k), \end{align*} $$

where $\zeta $ denotes a primitive nth root of unity.

The motivation for this paper is to extend the q-congruence (1.3) of Guo and Zeng, and establish a complete q-analogue of (1.2).

Theorem 1.1. Let n be an odd positive integer. Then, modulo $\Phi _n(q)^2$ ,

(1.4) $$ \begin{align} \sum_{k=0}^{(n-1)/2} & \frac{(q;q^2)_k^2}{(q^2;q^2)_k (q^4;q^4)_k}q^{2k}\notag\\[5pt] &\equiv \begin{cases} \displaystyle (-1)^{(n-1)/4}q^{(n-1)(n+3)/8}\frac{(q^2;q^4)_{(n-1)/4}}{(q^4;q^4)_{(n-1)/4}} &\text{if}\ n\equiv 1\pmod{4},\\ 0 &\text{if}\ n\equiv 3\pmod{4}. \end{cases} \end{align} $$

The important ingredients in the proof of (1.4) include Andrews’ $_4\phi _3$ terminating identity [2, (II.17), page 355]:

(1.5) $$ \begin{align} {}_{4}\phi_{3}\bigg[ \begin{matrix} q^{-n},a^2q^{n+1},c,-c\\[5pt] aq,-aq,c^2\end{matrix};q,q\bigg] =\begin{cases} 0 &\text{if}\ n\equiv 1\pmod{2},\\ \displaystyle \frac{c^n(q,a^2q^2/c^2;q^2)_{n/2}}{(a^2q^2,c^2q;q^2)_{n/2}} &\text{if}\ n\equiv 0\pmod{2}. \end{cases} \end{align} $$

The rest of the paper is organised as follows. In the next section, we shall explain why (1.4) is a q-analogue of (1.2). The proof of Theorem 1.1 will be presented in Section 3.

2. Why (1.4) is a q-analogue of (1.2)

Let p be an odd prime. It is clear that

$$ \begin{align*} \Phi_p(q)=1+q+\cdots+q^{p-1}. \end{align*} $$

Setting $n\to p$ and $q\to 1$ on both sides of (1.4) gives, modulo $p^2$ ,

(2.1) $$ \begin{align} \sum_{k=0}^{(p-1)/2}\frac{{2k\choose k}^2}{32^k} \equiv \begin{cases} \displaystyle \frac{(-1)^{(p-1)/4}}{2^{(p-1)/2}}{(p-1)/2\choose (p-1)/4} &\text{if}\ p\equiv 1\pmod{4},\\ 0 &\text{if}\ p\equiv 3\pmod{4}. \end{cases} \end{align} $$

By a result due to Chowla et al. [1],

$$ \begin{align*} {(p-1)/2\choose (p-1)/4}\equiv \frac{2^{p-1}+1}{2}\bigg(2x-\frac{p}{2x}\bigg)\pmod{p^2}, \end{align*} $$

where $p\equiv 1\pmod {4}$ and $p=x^2+y^2$ with $4\mid (x-1)$ . It follows that

(2.2) $$ \begin{align} \frac{(-1)^{(p-1)/4}}{2^{(p-1)/2}}{(p-1)/2\choose (p-1)/4} &\equiv (-1)^{(p-1)/4}\frac{2^{p-1}+1}{ 2^{(p+1)/2}}\bigg(2x-\frac{p}{2x}\bigg)\notag\\[5pt] &\equiv 2x-\frac{p}{2x}\pmod{p^2}, \end{align} $$

where we have used the fact [6, page 1918]:

$$ \begin{align*} \frac{2^{p-1}+1}{ 2^{(p+1)/2}}\equiv (-1)^{(p-1)/4}\pmod{p^2}. \end{align*} $$

Combining (2.1) and (2.2), we arrive at (1.2). Thus, (1.4) is indeed a q-analogue of (1.2).

3. Proof of Theorem 1.1

Let n be an odd positive integer. Setting $n\to (n-1)/2,q\to q^2,a\to 1$ on both sides of (1.5) gives

(3.1) $$ \begin{align} \sum_{k=0}^{(n-1)/2}\frac{(q^{1-n},q^{1+n},c,-c;q^2)_k}{(q^2,q^2,-q^2,c^2;q^2)_k}q^{2k} =\begin{cases} \displaystyle \frac{c^{(n-1)/2}(q^2,q^4/c^2;q^4)_{(n-1)/4}}{(q^4,c^2q^2;q^4)_{(n-1)/4}} &\text{if}\ n\equiv 1\pmod{4},\\ 0 &\text{if}\ n\equiv 3\pmod{4}. \end{cases} \end{align} $$

Letting $c\to 0$ on both sides of (3.1) and noting that for $n\equiv 1\pmod {4}$ ,

$$ \begin{align*} \lim_{c\to 0}(c,-c;q^2)_k=\lim_{c\to 0}(c^2;q^2)_k=\lim_{c\to 0}(c^2q^2;q^4)_{(n-1)/4}=1 \end{align*} $$

and

$$ \begin{align*} \lim_{c\to 0}c^{(n-1)/2}(q^4/c^2;q^4)_{(n-1)/4}=(-1)^{(n-1)/4}q^{(n-1)(n+3)/8}, \end{align*} $$

we obtain

(3.2) $$ \begin{align} \sum_{k=0}^{(n-1)/2} &\frac{(q^{1-n},q^{1+n};q^2)_k}{(q^2;q^2)_k(q^4;q^4)_k}q^{2k} \notag\\ & =\begin{cases} \displaystyle (-1)^{(n-1)/4}q^{(n-1)(n+3)/8}\frac{(q^2;q^4)_{(n-1)/4}}{(q^4;q^4)_{(n-1)/4}} &\text{if}\ n\equiv 1\pmod{4},\\ 0 &\text{if}\ n\equiv 3\pmod{4}. \end{cases} \end{align} $$

Since

$$ \begin{align*} (1-q^{n-2j+1})(1-q^{n+2j-1})+(1-q^{2j-1})^2q^{n-2j+1}=(1-q^n)^2 \end{align*} $$

and $1-q^n\equiv 0\pmod {\Phi _n(q)}$ ,

$$ \begin{align*} (1-q^{n-2j+1})(1-q^{n+2j-1})\equiv -(1-q^{2j-1})^2q^{n-2j+1}\pmod{\Phi_n(q)^2}. \end{align*} $$

It follows that

$$ \begin{align*} (1-q^{-n+2j-1})(1-q^{n+2j-1})\equiv (1-q^{2j-1})^2 \pmod{\Phi_n(q)^2}. \end{align*} $$

Thus,

(3.3) $$ \begin{align} (q^{1-n},q^{1+n};q^2)_k &=\prod_{j=1}^k (1-q^{-n+2j-1})(1-q^{n+2j-1})\notag\\ &\equiv \prod_{j=1}^k (1-q^{2j-1})^2 =(q;q^2)_k^2 \pmod{\Phi_n(q)^2}. \end{align} $$

Finally, substituting (3.3) into the left-hand side of (3.2) gives, modulo $\Phi _n(q)^2$ ,

$$ \begin{align*} \sum_{k=0}^{(n-1)/2} & \frac{(q;q^2)_k^2}{(q^2;q^2)_k(q^4;q^4)_k}q^{2k} \\ & \equiv \begin{cases} \displaystyle (-1)^{(n-1)/4}q^{(n-1)(n+3)/8}\frac{(q^2;q^4)_{(n-1)/4}}{(q^4;q^4)_{(n-1)/4}} &\text{if}\ n\equiv 1\pmod{4},\\ 0 &\text{if}\ n\equiv 3\pmod{4}, \end{cases} \end{align*} $$

as desired.