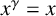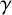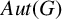1 Introduction
An automorphism of a group G is said to be a central automorphism if it acts trivially on the centre factor group
![]() $G/Z(G)$
. It is easy to show that an automorphism of G is central if and only if it is normal, that is, it commutes with all inner automorphisms of G. It follows that the set
$G/Z(G)$
. It is easy to show that an automorphism of G is central if and only if it is normal, that is, it commutes with all inner automorphisms of G. It follows that the set
![]() $Aut_c(G)$
of all central automorphisms of G is a normal subgroup of the full automorphism group
$Aut_c(G)$
of all central automorphisms of G is a normal subgroup of the full automorphism group
![]() $Aut(G)$
of G. Clearly, if G has trivial centre, then the identity is the unique central automorphism of G, while all automorphisms of G are central if G is abelian. We also note that each inner automorphism of G is central if and only if G is nilpotent with class at most
$Aut(G)$
of G. Clearly, if G has trivial centre, then the identity is the unique central automorphism of G, while all automorphisms of G are central if G is abelian. We also note that each inner automorphism of G is central if and only if G is nilpotent with class at most
![]() $2$
.
$2$
.
Recall that an automorphism
![]() $\alpha $
of a group G is said to be a power automorphism if
$\alpha $
of a group G is said to be a power automorphism if
![]() $X^{\alpha }=X$
for each subgroup X of G. A relevant theorem by Cooper [Reference Cooper5] ensures that the (normal) subgroup
$X^{\alpha }=X$
for each subgroup X of G. A relevant theorem by Cooper [Reference Cooper5] ensures that the (normal) subgroup
![]() $PAut(G)$
of
$PAut(G)$
of
![]() $Aut(G)$
consisting of all power automorphisms of G is contained in
$Aut(G)$
consisting of all power automorphisms of G is contained in
![]() $Aut_c(G)$
. This result was extended to cyclic automorphisms in [Reference de Giovanni, Newell and Russo7]. (Recall here that an automorphism
$Aut_c(G)$
. This result was extended to cyclic automorphisms in [Reference de Giovanni, Newell and Russo7]. (Recall here that an automorphism
![]() $\alpha $
of a group G is said to be a cyclic automorphism if the subgroup
$\alpha $
of a group G is said to be a cyclic automorphism if the subgroup
![]() $\langle x,x^{\alpha }\rangle $
is cyclic for any element
$\langle x,x^{\alpha }\rangle $
is cyclic for any element
![]() $x\in G$
(see also [Reference Brescia and Russo3]).)
$x\in G$
(see also [Reference Brescia and Russo3]).)
Let G be a group. It is easy to show that if
![]() $\gamma $
is a central automorphism of a group G, then the map
$\gamma $
is a central automorphism of a group G, then the map
is a homomorphism from G into
![]() $Z(G)$
. In particular,
$Z(G)$
. In particular,
![]() $\gamma $
acts trivially over the commutator subgroup
$\gamma $
acts trivially over the commutator subgroup
![]() $G'$
of G. Following [Reference Catino, de Giovanni and Miccoli4], we define the central kernel of G as the subgroup
$G'$
of G. Following [Reference Catino, de Giovanni and Miccoli4], we define the central kernel of G as the subgroup
 $$ \begin{align*}K(G)=\bigcap\limits_{\gamma \in Aut_c(G)}\ker f_{\gamma}\end{align*} $$
$$ \begin{align*}K(G)=\bigcap\limits_{\gamma \in Aut_c(G)}\ker f_{\gamma}\end{align*} $$
of G consisting of all elements
![]() $x\in G$
such that
$x\in G$
such that
![]() $x^{\gamma }=x$
for every central automorphism
$x^{\gamma }=x$
for every central automorphism
![]() $\gamma $
of G. Obviously,
$\gamma $
of G. Obviously,
![]() $K(G)$
is a characteristic subgroup of G since
$K(G)$
is a characteristic subgroup of G since
![]() $Aut_c(G)$
is a normal subgroup of
$Aut_c(G)$
is a normal subgroup of
![]() $Aut(G)$
. The subgroup
$Aut(G)$
. The subgroup
![]() $K(G)$
was first considered by Haimo [Reference Haimo8] in 1955 and its importance was pointed out later by Pettet [Reference Pettet11]. More recently, Catino et al. [Reference Catino, de Giovanni and Miccoli4] have investigated finite-by-nilpotent groups in which the central kernel is large in some sense. (Recall here that a group G is said to be finite-by-nilpotent if there exists a positive integer n such that the term
$K(G)$
was first considered by Haimo [Reference Haimo8] in 1955 and its importance was pointed out later by Pettet [Reference Pettet11]. More recently, Catino et al. [Reference Catino, de Giovanni and Miccoli4] have investigated finite-by-nilpotent groups in which the central kernel is large in some sense. (Recall here that a group G is said to be finite-by-nilpotent if there exists a positive integer n such that the term
![]() $\gamma _n(G)$
of the lower central series of G is finite.) They proved, among other results, that a finite-by-nilpotent group G is central-by-finite whenever its central kernel
$\gamma _n(G)$
of the lower central series of G is finite.) They proved, among other results, that a finite-by-nilpotent group G is central-by-finite whenever its central kernel
![]() $K(G)$
has finite index (and hence the celebrated Schur’s theorem [Reference Robinson12, Theorem 10.1.4] yields that G is finite-by-abelian). Moreover, the subgroup consisting of all elements of finite order of G is finite (see [Reference Catino, de Giovanni and Miccoli4, Theorem A]).
$K(G)$
has finite index (and hence the celebrated Schur’s theorem [Reference Robinson12, Theorem 10.1.4] yields that G is finite-by-abelian). Moreover, the subgroup consisting of all elements of finite order of G is finite (see [Reference Catino, de Giovanni and Miccoli4, Theorem A]).
In this short article, we improve the latter result showing that if G is a finite-by-nilpotent group whose central kernel has finite index, then the full automorphism group
![]() $Aut(G)$
of G is finite. As a consequence, we obtain the theorem of Hegarty [Reference Hegarty9] stating that the autocommutator subgroup
$Aut(G)$
of G is finite. As a consequence, we obtain the theorem of Hegarty [Reference Hegarty9] stating that the autocommutator subgroup
![]() $[G, Aut(G)]$
of a group G is finite whenever its absolute centre
$[G, Aut(G)]$
of a group G is finite whenever its absolute centre
![]() $C_G(Aut(G))$
has finite index.
$C_G(Aut(G))$
has finite index.
Most of our notation is standard and can be found in [Reference Robinson12].
2 Results
Let G be a group, and consider a group
![]() $\Gamma $
of automorphisms of G. The interaction between
$\Gamma $
of automorphisms of G. The interaction between
![]() $\Gamma $
and the subgroups
$\Gamma $
and the subgroups
and
of G was first investigated in 1952 by Baer [Reference Baer2], who proved in particular the following result.
Lemma 2.1. Let G be a group and let
![]() $\Gamma $
be a group of automorphisms of G. Then the finiteness of any two between
$\Gamma $
be a group of automorphisms of G. Then the finiteness of any two between
![]() $\Gamma $
,
$\Gamma $
,
![]() $|G:C_G(\Gamma )|$
and
$|G:C_G(\Gamma )|$
and
![]() $[G, \Gamma ]$
implies the finiteness of the third.
$[G, \Gamma ]$
implies the finiteness of the third.
Our next result gives information of this type concerning the group of central automorphisms of a group.
Lemma 2.2. Let G be any group. If the subgroup
![]() $A=K(G)\cap Z(G)$
has finite index in G, then the subgroup
$A=K(G)\cap Z(G)$
has finite index in G, then the subgroup
![]() $[G, Aut_c(G)]$
of G has finite exponent.
$[G, Aut_c(G)]$
of G has finite exponent.
Proof. Put
![]() $|G:A|=n$
. As the subgroup A lies in the centre of G, the transfer homomorphism of G into A is the map
$|G:A|=n$
. As the subgroup A lies in the centre of G, the transfer homomorphism of G into A is the map
Let g and
![]() $\gamma $
be elements of G and
$\gamma $
be elements of G and
![]() $Aut_c(G)$
, respectively. Then
$Aut_c(G)$
, respectively. Then
![]() $(g^{\gamma })^n=(g^n)^{\gamma }=g^n$
, so
$(g^{\gamma })^n=(g^n)^{\gamma }=g^n$
, so
It follows that
![]() $[G, Aut_c(G)]$
is contained in the kernel of
$[G, Aut_c(G)]$
is contained in the kernel of
![]() $\tau $
and
$\tau $
and
![]() $[G, Aut_c(G)]^n=\{1\}$
.
$[G, Aut_c(G)]^n=\{1\}$
.
As in many investigations concerning automorphisms, we will use in our arguments some cohomological methods. Let
be a central extension of a group A by a group Q. Without loss of generality, it can be assumed that A is a central subgroup of G,
![]() $\mu $
is the embedding of A into G, Q is the factor group
$\mu $
is the embedding of A into G, Q is the factor group
![]() $G/A$
and
$G/A$
and
![]() $\varepsilon $
is the natural projection. Recall that a transversal map
$\varepsilon $
is the natural projection. Recall that a transversal map
of
![]() $\Sigma $
is a function such that
$\Sigma $
is a function such that
![]() $\tau \varepsilon =\iota _Q$
, so that the set
$\tau \varepsilon =\iota _Q$
, so that the set
is a transversal to A in G. If
![]() $x,y\in Q$
, we have
$x,y\in Q$
, we have
It follows that there exists a unique element
![]() $\varphi (x,y)\in A(=\ker \varepsilon $
) such that
$\varphi (x,y)\in A(=\ker \varepsilon $
) such that
If
![]() $x,y,z$
are elements of Q, from the equality
$x,y,z$
are elements of Q, from the equality
![]() $x^{\tau }(y^{\tau }z^{\tau })=(x^{\tau }y^{\tau })z^{\tau }$
, it follows that
$x^{\tau }(y^{\tau }z^{\tau })=(x^{\tau }y^{\tau })z^{\tau }$
, it follows that
Therefore, the map
is a 2-cocycle of Q in A. The coset
is an element of the second cohomology group
![]() $H^2(Q,A)$
of Q with coefficients in A, which depends only on the extension
$H^2(Q,A)$
of Q with coefficients in A, which depends only on the extension
![]() $\Sigma $
and not on the choice of the transversal function. The element
$\Sigma $
and not on the choice of the transversal function. The element
![]() $\Delta $
is called the cohomology class of
$\Delta $
is called the cohomology class of
![]() $\Sigma $
.
$\Sigma $
.
Let
![]() $\alpha $
be an automorphism of A. An easy application of [Reference Stammbach13, Proposition II 4.3] shows that
$\alpha $
be an automorphism of A. An easy application of [Reference Stammbach13, Proposition II 4.3] shows that
![]() $\alpha $
can be extended to an automorphism
$\alpha $
can be extended to an automorphism
![]() $\beta $
of G inducing the identity on
$\beta $
of G inducing the identity on
![]() $G/A$
if
$G/A$
if
for all
![]() $x,y\in Q$
. Clearly,
$x,y\in Q$
. Clearly,
![]() $\beta $
is a central automorphism G. As an immediate consequence of the previous considerations, we have the following result which is useful for constructing central automorphisms.
$\beta $
is a central automorphism G. As an immediate consequence of the previous considerations, we have the following result which is useful for constructing central automorphisms.
Lemma 2.3. Let G be a group and consider the central extension
where
![]() $A=K(G)\cap Z(G)$
. If
$A=K(G)\cap Z(G)$
. If
![]() $\Delta =\varphi +B^2(Q,A)$
is the cohomology class of
$\Delta =\varphi +B^2(Q,A)$
is the cohomology class of
![]() $\Sigma $
and
$\Sigma $
and
![]() $\alpha $
is an automorphism of A such that
$\alpha $
is an automorphism of A such that
for all
![]() $xA,yA\in G/A$
, then
$xA,yA\in G/A$
, then
![]() $\alpha $
is the identity of A.
$\alpha $
is the identity of A.
Now we are in a position to prove our main result.
Theorem 2.4. Let G be a finite-by-nilpotent group in which the central kernel
![]() $K(G)$
has finite index. Then the full automorphism group
$K(G)$
has finite index. Then the full automorphism group
![]() $Aut(G)$
of G is finite. In particular, the subgroup of all elements of finite order of G is finite.
$Aut(G)$
of G is finite. In particular, the subgroup of all elements of finite order of G is finite.
Proof. First we note that the factor group
![]() $G/Z(G)$
is finite by [Reference Catino, de Giovanni and Miccoli4, Theorem A]. As
$G/Z(G)$
is finite by [Reference Catino, de Giovanni and Miccoli4, Theorem A]. As
![]() $A=K(G)\cap Z(G)$
is a characteristic subgroup of G, then every automorphism
$A=K(G)\cap Z(G)$
is a characteristic subgroup of G, then every automorphism
![]() $\gamma $
of G induces an automorphism
$\gamma $
of G induces an automorphism
![]() $\bar \gamma $
on the finite group
$\bar \gamma $
on the finite group
![]() $G/A$
. Therefore, we may consider the homomorphism
$G/A$
. Therefore, we may consider the homomorphism
Clearly, the kernel
![]() $\Gamma $
of f is a subgroup of
$\Gamma $
of f is a subgroup of
![]() $Aut_c(G)$
, and hence the subgroup
$Aut_c(G)$
, and hence the subgroup
![]() $[G, \Gamma ]$
has finite exponent by Lemma 2.2.
$[G, \Gamma ]$
has finite exponent by Lemma 2.2.
Assume for a contradiction that
![]() $\Gamma $
is infinite, so that
$\Gamma $
is infinite, so that
![]() $[G, \Gamma ]$
is likewise infinite by Lemma 2.1. Since
$[G, \Gamma ]$
is likewise infinite by Lemma 2.1. Since
![]() $[G, \Gamma ]$
is a subgroup of A with finite exponent, it follows that the p-component P of A has infinite rank for some prime p. Let D be the largest divisible subgroup of P. Then A splits over D, so that
$[G, \Gamma ]$
is a subgroup of A with finite exponent, it follows that the p-component P of A has infinite rank for some prime p. Let D be the largest divisible subgroup of P. Then A splits over D, so that
![]() $A=D\times E$
and hence
$A=D\times E$
and hence
![]() $P=D\times R$
, where
$P=D\times R$
, where
![]() $R=E\cap P$
is a reduced subgroup. First suppose that the subgroup D has finite rank. Then there exists a sequence
$R=E\cap P$
is a reduced subgroup. First suppose that the subgroup D has finite rank. Then there exists a sequence
![]() $(X_n)_{n\in \mathbb {N}}$
of cyclic nontrivial subgroups of R such that
$(X_n)_{n\in \mathbb {N}}$
of cyclic nontrivial subgroups of R such that
for all positive integers n and suitable subgroups
![]() $R_n$
(see [Reference Robinson12, Propositions 4.3.3 and 4.3.8]). Moreover, since
$R_n$
(see [Reference Robinson12, Propositions 4.3.3 and 4.3.8]). Moreover, since
![]() $X_1\times \cdots \times X_n$
is a finite direct factor of P and P is pure in A for each n, there exists a subgroup
$X_1\times \cdots \times X_n$
is a finite direct factor of P and P is pure in A for each n, there exists a subgroup
![]() $A_n$
of A such that
$A_n$
of A such that
Clearly, if D has infinite rank, we have a similar decomposition of A for each n, where all the subgroups
![]() $X_1, \ldots , X_n$
are of type
$X_1, \ldots , X_n$
are of type
![]() $p^{\infty }$
.
$p^{\infty }$
.
Let
![]() $\Delta =\varphi +B^2(G/A,A)$
be the cohomology class of the central extension
$\Delta =\varphi +B^2(G/A,A)$
be the cohomology class of the central extension
By hypothesis, the set
![]() $\{\varphi (xA,yA) \mid xA,yA\in G/A\}$
is finite. It follows that for a sufficiently large n, there exist two direct factors U and V of the decomposition
$\{\varphi (xA,yA) \mid xA,yA\in G/A\}$
is finite. It follows that for a sufficiently large n, there exist two direct factors U and V of the decomposition
![]() $A=X_1\times \cdots \times X_n\times A_n$
of A such that
$A=X_1\times \cdots \times X_n\times A_n$
of A such that
for all
![]() $xA,yA\in G/A$
. Let
$xA,yA\in G/A$
. Let
![]() $\alpha $
be a nonidentity automorphism of
$\alpha $
be a nonidentity automorphism of
![]() $U\times V$
. Clearly,
$U\times V$
. Clearly,
![]() $\alpha $
can be extended to an automorphism
$\alpha $
can be extended to an automorphism
![]() $\beta $
of A acting trivially over all direct factors other than U and V of the decomposition
$\beta $
of A acting trivially over all direct factors other than U and V of the decomposition
![]() $A=X_1\times \cdots \times X_n\times A_n$
of A. This is a contradiction by Lemma 2.3. Therefore, the full automorphism group
$A=X_1\times \cdots \times X_n\times A_n$
of A. This is a contradiction by Lemma 2.3. Therefore, the full automorphism group
![]() $Aut(G)$
of G is finite as required.
$Aut(G)$
of G is finite as required.
Finally, as the commutator subgroup
![]() $G'$
of G is finite by Schur’s theorem, it follows that the set T of all elements of finite order of G is a subgroup, and hence T is finite by a result of Nagrebeckiı̆ [Reference Nagrebeckiĭ10].
$G'$
of G is finite by Schur’s theorem, it follows that the set T of all elements of finite order of G is a subgroup, and hence T is finite by a result of Nagrebeckiı̆ [Reference Nagrebeckiĭ10].
Corollary 2.5. Let G be a finitely generated infinite nilpotent group such that the index
![]() $|G:K(G)|$
is finite. Then G contains a central infinite cyclic subgroup of finite index.
$|G:K(G)|$
is finite. Then G contains a central infinite cyclic subgroup of finite index.
Proof. By Theorem 2.4, the full automorphism group
![]() $Aut(G)$
of G is finite, and hence the statement follows from a celebrated result by Alperin (see [Reference Alperin1, Theorem 1]).
$Aut(G)$
of G is finite, and hence the statement follows from a celebrated result by Alperin (see [Reference Alperin1, Theorem 1]).
We note that the above result has been proved with different arguments also in [Reference Catino, de Giovanni and Miccoli4, Proposition 2.1].
Let G be a group. Following [Reference Hegarty9], the absolute centre (or autocentre) of G is the characteristic subgroup
![]() $C_G(Aut(G))$
of G consisting of all elements of G fixed by every automorphism of G. Clearly,
$C_G(Aut(G))$
of G consisting of all elements of G fixed by every automorphism of G. Clearly,
![]() $C_G(Aut(G))$
is contained in the central kernel
$C_G(Aut(G))$
is contained in the central kernel
![]() $K(G)$
of G. Therefore, if the index
$K(G)$
of G. Therefore, if the index
![]() $|G:C_G(Aut(G))|$
is finite, then Theorem 2.4 yields that
$|G:C_G(Aut(G))|$
is finite, then Theorem 2.4 yields that
![]() $Aut(G)$
is finite, and hence the autocommutator subgroup
$Aut(G)$
is finite, and hence the autocommutator subgroup
![]() $[G,Aut(G)]$
of G is likewise finite by Lemma 2.1. Thus, we have obtained the following result that was first proved by Hegarty [Reference Hegarty9].
$[G,Aut(G)]$
of G is likewise finite by Lemma 2.1. Thus, we have obtained the following result that was first proved by Hegarty [Reference Hegarty9].
Corollary 2.6. If the absolute centre
![]() $C_G(Aut(G))$
of a group G has finite index, then the autocommutator subgroup
$C_G(Aut(G))$
of a group G has finite index, then the autocommutator subgroup
![]() $[G,Aut(G)]$
is finite.
$[G,Aut(G)]$
is finite.
We point out that another generalisation of Hegarty’s theorem was obtained by de Giovanni, Newell and the author [Reference de Giovanni, Newell and Russo6] in 2014.
Let G be a nilpotent group with class at most
![]() $2$
. Then
$2$
. Then
![]() ${\mathit {Inn}}(G)\leq Aut_c(G)$
and hence
${\mathit {Inn}}(G)\leq Aut_c(G)$
and hence
![]() $K(G)\leq Z(G)$
. Conversely, as a central automorphism acts trivially over the commutator subgroup
$K(G)\leq Z(G)$
. Conversely, as a central automorphism acts trivially over the commutator subgroup
![]() $G'$
of G, we see that G is nilpotent with class at most
$G'$
of G, we see that G is nilpotent with class at most
![]() $2$
if the central kernel of G is contained in
$2$
if the central kernel of G is contained in
![]() $Z(G)$
. Now we construct an infinite nonabelian group G such that
$Z(G)$
. Now we construct an infinite nonabelian group G such that
![]() $Z(G)=K(G)\neq C_G(Aut(G))$
.
$Z(G)=K(G)\neq C_G(Aut(G))$
.
Let
![]() $A=\langle x\rangle $
and
$A=\langle x\rangle $
and
![]() $B=\langle y\rangle $
be a cyclic group of order 9 and an infinite cyclic group, respectively. Consider the semidirect product
$B=\langle y\rangle $
be a cyclic group of order 9 and an infinite cyclic group, respectively. Consider the semidirect product
where
![]() $x^y=x^4$
and
$x^y=x^4$
and
![]() $[x,B^3]=\{1\}$
. Clearly,
$[x,B^3]=\{1\}$
. Clearly,
![]() $Z(G)=B^3\times \langle x^3\rangle $
and
$Z(G)=B^3\times \langle x^3\rangle $
and
![]() $G'=\langle x^3\rangle $
. Let
$G'=\langle x^3\rangle $
. Let
![]() $\gamma $
be a central automorphism of G. Then
$\gamma $
be a central automorphism of G. Then
![]() $y^{\gamma }=yz$
for some central element z. Moreover,
$y^{\gamma }=yz$
for some central element z. Moreover,
![]() $\gamma $
induces an automorphism over the characteristic subgroup
$\gamma $
induces an automorphism over the characteristic subgroup
![]() $B^9$
. First suppose that
$B^9$
. First suppose that
![]() $(y^9)^{\gamma }=y^{-9}$
. If
$(y^9)^{\gamma }=y^{-9}$
. If
![]() $z=y^{3i}x^{3j}$
for some integers i and j, then
$z=y^{3i}x^{3j}$
for some integers i and j, then
and so
![]() $y^{27i+18}=1$
, which is a contradiction. Therefore,
$y^{27i+18}=1$
, which is a contradiction. Therefore,
![]() $y^9=(y^9)^{\gamma }=y^9z^9$
and hence
$y^9=(y^9)^{\gamma }=y^9z^9$
and hence
![]() $z=x^{3t}$
for some nonnegative integer t. It follows that
$z=x^{3t}$
for some nonnegative integer t. It follows that
![]() $(y^3)^{\gamma }=y^3$
so that
$(y^3)^{\gamma }=y^3$
so that
![]() $\gamma $
acts trivially on
$\gamma $
acts trivially on
![]() $Z(G)$
. Thus,
$Z(G)$
. Thus,
![]() $K(G)=Z(G)$
. Clearly, every inner automorphism of G is central. However, it is easy to show that
$K(G)=Z(G)$
. Clearly, every inner automorphism of G is central. However, it is easy to show that
![]() $Aut_c(G)\simeq \mbox {Hom}(G/Z(G),Z(G))\simeq \mathbb {Z}_3\times \mathbb {Z}_3 $
, so that
$Aut_c(G)\simeq \mbox {Hom}(G/Z(G),Z(G))\simeq \mathbb {Z}_3\times \mathbb {Z}_3 $
, so that
![]() ${\mathit {Inn}}(G)=Aut_c(G)$
.
${\mathit {Inn}}(G)=Aut_c(G)$
.
Finally, consider the automorphism
![]() $\alpha :x\mapsto x^2$
of A and
$\alpha :x\mapsto x^2$
of A and
![]() $\beta =\iota _B$
of B. Since
$\beta =\iota _B$
of B. Since
there exists an automorphism
![]() $\gamma $
of G inducing
$\gamma $
of G inducing
![]() $\alpha $
and
$\alpha $
and
![]() $\beta $
. We note that
$\beta $
. We note that
![]() $\gamma $
cannot be central since
$\gamma $
cannot be central since
![]() $(x^3)^{\alpha }=x^{-3}$
. It follows that
$(x^3)^{\alpha }=x^{-3}$
. It follows that
![]() $C_G(Aut(G))\neq K(G)$
.
$C_G(Aut(G))\neq K(G)$
.
Let G be a group, and denote by
![]() $\overline K(G)$
the set of all elements x of G such that
$\overline K(G)$
the set of all elements x of G such that
![]() $x^{\alpha }=x$
for every power automorphism
$x^{\alpha }=x$
for every power automorphism
![]() $\alpha $
of G. Clearly,
$\alpha $
of G. Clearly,
![]() $\overline K(G)$
is a characteristic subgroup of G containing the central kernel
$\overline K(G)$
is a characteristic subgroup of G containing the central kernel
![]() $K(G)$
of G and the subgroup
$K(G)$
of G and the subgroup
![]() $G[2]=\langle g\in G\;|\;g^2=1\rangle $
. Note that the consideration of the direct product
$G[2]=\langle g\in G\;|\;g^2=1\rangle $
. Note that the consideration of the direct product
![]() $G=A\times B$
, where A is a cyclic group of order
$G=A\times B$
, where A is a cyclic group of order
![]() $3$
and B is a countably infinite abelian group of exponent
$3$
and B is a countably infinite abelian group of exponent
![]() $2$
, shows that we cannot replace in Theorem 2.4 the central kernel by the subgroup
$2$
, shows that we cannot replace in Theorem 2.4 the central kernel by the subgroup
![]() $\overline K(G)$
. Nevertheless, it is easy to prove the following result.
$\overline K(G)$
. Nevertheless, it is easy to prove the following result.
Proposition 2.7. Let G be a finite-by-nilpotent group such that the index
![]() $|G:\overline K(G)|$
is finite. Then the group
$|G:\overline K(G)|$
is finite. Then the group
![]() $PAut(G)$
of all power automorphisms of G is finite.
$PAut(G)$
of all power automorphisms of G is finite.
Proof. First suppose that G is a nonperiodic group. By hypothesis, the term
![]() $\gamma _n(G)$
of the lower central series of G is finite for some positive integer n. It follows that the set of periodic elements of G is a subgroup, so that G is a weak group. Thus,
$\gamma _n(G)$
of the lower central series of G is finite for some positive integer n. It follows that the set of periodic elements of G is a subgroup, so that G is a weak group. Thus,
![]() $PAut(G)$
is finite of order at most
$PAut(G)$
is finite of order at most
![]() $2$
(see [Reference Cooper5, Corollary 4.2.3]).
$2$
(see [Reference Cooper5, Corollary 4.2.3]).
If G is periodic, then there exists a finite subgroup F of G such that
![]() $G=F\overline K(G)$
. Let
$G=F\overline K(G)$
. Let
![]() $\alpha $
be a power automorphism of G. Then
$\alpha $
be a power automorphism of G. Then
![]() $F^{\alpha }=F$
and
$F^{\alpha }=F$
and
![]() $x^{\alpha }=x$
for every
$x^{\alpha }=x$
for every
![]() $x\in \overline K(G)$
. It follows that the map
$x\in \overline K(G)$
. It follows that the map
is injective and hence
![]() $PAut(G)$
is again finite.
$PAut(G)$
is again finite.