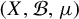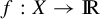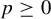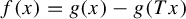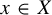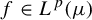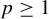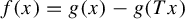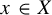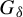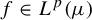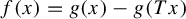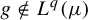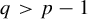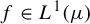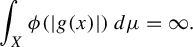1 Introduction
We give new fundamental results concerning solutions to the coboundary equation:
There has been substantial progress in many cases such as homogeneous spaces, smooth actions, Lie groups, as well as many other important families of dynamical systems. Most previous research focuses on the case where a measurable transformation, or topological dynamical system is specified, and a solution g is sought for individual f or families of functions f (e.g., Hölder f). In this paper, we study the situation from the general perspective of solutions T and g where f may be any real-valued measurable function, or function
![]() $f \in L^p$
for
$f \in L^p$
for
![]() $p \geq 0$
.
$p \geq 0$
.
In this paper, we assume all measurable dynamical systems are defined on a Lebesgue space
![]() $(X, {\mathcal B}, \mu )$
. Thus, by the Rokhlin isomorphism theorem [Reference Rokhlin38, Reference Rokhlin39], we take
$(X, {\mathcal B}, \mu )$
. Thus, by the Rokhlin isomorphism theorem [Reference Rokhlin38, Reference Rokhlin39], we take
![]() $X=[0,1)$
,
$X=[0,1)$
,
![]() $\mu $
is Lebesgue measure, and
$\mu $
is Lebesgue measure, and
![]() ${\mathcal B}$
is the collection of Lebesgue measurable sets. For
${\mathcal B}$
is the collection of Lebesgue measurable sets. For
![]() $p>~0$
, define the standard
$p>~0$
, define the standard
![]() $L^p$
space,
$L^p$
space,
![]() . For
. For
![]() $p\geq 1$
, define
$p\geq 1$
, define
![]() $L^p_0 = \{ f\in L^p : \int _X f\,d\mu = 0 \}$
. Also,
$L^p_0 = \{ f\in L^p : \int _X f\,d\mu = 0 \}$
. Also,
![]() $L^{\infty }$
is the set of essentially bounded measurable functions on
$L^{\infty }$
is the set of essentially bounded measurable functions on
![]() $(X, {\mathcal B}, \mu )$
and similarly,
$(X, {\mathcal B}, \mu )$
and similarly,
![]() $L^{\infty }_0$
are functions in
$L^{\infty }_0$
are functions in
![]() $L^{\infty }$
with zero integral. The space
$L^{\infty }$
with zero integral. The space
![]() $L^0$
is the set of measurable functions on
$L^0$
is the set of measurable functions on
![]() $(X, {\mathcal B}, \mu )$
. Let
$(X, {\mathcal B}, \mu )$
. Let
![]() $\mathcal {M}$
be the family of invertible measure-preserving transformations defined on
$\mathcal {M}$
be the family of invertible measure-preserving transformations defined on
![]() $(X, {\mathcal B}, \mu )$
and
$(X, {\mathcal B}, \mu )$
and
![]() $\mathcal {E}$
is the family of ergodic invertible measure-preserving transformations on
$\mathcal {E}$
is the family of ergodic invertible measure-preserving transformations on
![]() $(X, {\mathcal B}, \mu )$
. We obtain the following main positive result.
$(X, {\mathcal B}, \mu )$
. We obtain the following main positive result.
Theorem 1.1. (Existence of solutions)
Let
![]() $1 \leq p \leq \infty $
and suppose
$1 \leq p \leq \infty $
and suppose
![]() $f \in L^p_0$
. There exist
$f \in L^p_0$
. There exist
![]() $T \in \mathcal {E}$
and
$T \in \mathcal {E}$
and
![]() $g\in L^{p-1}$
such that
$g\in L^{p-1}$
such that
![]() $f(x) = g(x) - g(Tx)$
for almost every (a.e.)
$f(x) = g(x) - g(Tx)$
for almost every (a.e.)
![]() $x \in X$
.
$x \in X$
.
In some sense, Theorem 1.1 gives the best possible positive result. The following theorem demonstrates a major limitation for solutions to the coboundary equation. In particular, typically, there is no solution g in the same integrability class as f, even when allowing T to range over all of
![]() $\mathcal {E}$
.
$\mathcal {E}$
.
Theorem 1.2. (
 $L^q$
non-existence)
$L^q$
non-existence)
Given
![]() $1 \leq p < \infty $
, there exist
$1 \leq p < \infty $
, there exist
![]() $f \in L^p_0$
such that for any solution
$f \in L^p_0$
such that for any solution
![]() $T \in \mathcal {E}$
and measurable g to the coboundary equation
$T \in \mathcal {E}$
and measurable g to the coboundary equation
![]() $f = g - g \circ T$
, then
$f = g - g \circ T$
, then
![]() $g \notin L^q$
for
$g \notin L^q$
for
![]() $q> p - 1$
. More generally, there exists a dense
$q> p - 1$
. More generally, there exists a dense
![]() $G_{\delta }$
set
$G_{\delta }$
set
![]() $\mathcal {G}_p \subset L^p_0$
such that for any
$\mathcal {G}_p \subset L^p_0$
such that for any
![]() $f \in \mathcal {G}_p$
, and any solution pair
$f \in \mathcal {G}_p$
, and any solution pair
![]() $T, g$
with
$T, g$
with
![]() $T \in \mathcal {E}$
, then
$T \in \mathcal {E}$
, then
![]() $g \notin L^q$
for
$g \notin L^q$
for
![]() $q> p - 1$
.
$q> p - 1$
.
The solution g is referred to as the transfer function for coboundary f. Theorem 1.2 implies that for generic mean-zero
![]() $f \in L^p$
for
$f \in L^p$
for
![]() $p < 2$
, any transfer function is not integrable, regardless of
$p < 2$
, any transfer function is not integrable, regardless of
![]() $T \in \mathcal {E}$
. However, for
$T \in \mathcal {E}$
. However, for
![]() $f \in L^1_0$
, we can always find a solution with measurable
$f \in L^1_0$
, we can always find a solution with measurable
![]() $g \in L^0$
.
$g \in L^0$
.
For the case where f is only assumed to be measurable, we give a straightforward equivalent condition for the existence of a measurable transfer function. Also, Theorem 1.3 highlights the need to control T, or the inter-dependence of T and f, if one hopes to find a measurable transfer function.
Theorem 1.3. (Measurable transfer functions)
Suppose
![]() $(X, {\mathcal B}, \mu )$
is a standard probability space and
$(X, {\mathcal B}, \mu )$
is a standard probability space and
![]() $f \in L^0$
is non-zero on a set of positive measure.
$f \in L^0$
is non-zero on a set of positive measure.
-
• The coboundary equation
 $f = g - g\circ T$
has a solution pair,
$f = g - g\circ T$
has a solution pair,
 $T \in \mathcal {E}$
,
$T \in \mathcal {E}$
,
 $g \in L^0$
, if and only if
$g \in L^0$
, if and only if
 $\int _{f> 0} f\,d\mu = - \int _{f < 0} f\,d\mu $
, whether both integrals are
$\int _{f> 0} f\,d\mu = - \int _{f < 0} f\,d\mu $
, whether both integrals are
 $\infty $
or finite.
$\infty $
or finite. -
• The class of ergodic invertible measure-preserving transformations T such that
 $f = g - g \circ T$
has a measurable solution g is first category (that is, meager).
$f = g - g \circ T$
has a measurable solution g is first category (that is, meager).
2 Connections to previous research
There has been substantial interest in the study of the cohomology of dynamical systems. Much of the recent focus is on smooth dynamics including hyperbolic actions or actions of Lie groups. Powerful rigidity or local rigidity results have been obtained involving cocycles. Some of the earliest results include [Reference Katok and Spatzier27, Reference Katok and Spatzier28]. Cocycle rigidity depends closely on solving the coboundary equation, since the difference between cohomologous cocycles is a coboundary. Liv
![]() $\breve{\mathrm{s}}$
ic [Reference Livšic34] provided one of the earliest regularity results in this setting by demonstrating Hölder cocycle rigidity for families of U-systems, topological Markov chains, and Smale systems. More recently, this Hölder regularity has been extended to non-uniformly expanding Markov maps [Reference Gouëzel22], and to Weyl chamber flows or twisted Weyl chamber flows [Reference Vinhage42]. In [Reference Veech41], Veech proves that the coboundary equation
$\breve{\mathrm{s}}$
ic [Reference Livšic34] provided one of the earliest regularity results in this setting by demonstrating Hölder cocycle rigidity for families of U-systems, topological Markov chains, and Smale systems. More recently, this Hölder regularity has been extended to non-uniformly expanding Markov maps [Reference Gouëzel22], and to Weyl chamber flows or twisted Weyl chamber flows [Reference Vinhage42]. In [Reference Veech41], Veech proves that the coboundary equation
![]() $f = g - g\circ T$
admits a
$f = g - g\circ T$
admits a
![]() $C^{\infty }$
solution g for
$C^{\infty }$
solution g for
![]() $C^{\infty } f$
when T is an ergodic toral endomorphism and f sums to zero over every periodic orbit. Also, a connection is made to the generalized Riemann hypothesis.
$C^{\infty } f$
when T is an ergodic toral endomorphism and f sums to zero over every periodic orbit. Also, a connection is made to the generalized Riemann hypothesis.
We will consider the coboundary equation in a general context. In the setting of topological dynamics, the following early result was observed in Gottschalk and Hedlund [Reference Gottschalk and Hedlund21]: a bounded continuous function f is a coboundary for a minimal homeomorphism on a compact space if and only if the following is uniformly bounded for positive n,
 $$ \begin{align*} \bigg| \sum_{i=0}^{n-1} f ( T^i x ) \bigg|. \end{align*} $$
$$ \begin{align*} \bigg| \sum_{i=0}^{n-1} f ( T^i x ) \bigg|. \end{align*} $$
More recently, Quas [Reference Quas37] proves for a
![]() $\mu $
-invariant minimal homeomorphism on a compact probability space, if a continuous f is a coboundary with an
$\mu $
-invariant minimal homeomorphism on a compact probability space, if a continuous f is a coboundary with an
![]() $L^{\infty }(\mu )$
transfer function, then f is a coboundary with a continuous transfer function. Also, we would like to mention a result of Baggett, Medina, and Merrill which is in the same spirit of Theorem 1.3. They prove in [Reference Baggett, Medina and Merrill5] that if
$L^{\infty }(\mu )$
transfer function, then f is a coboundary with a continuous transfer function. Also, we would like to mention a result of Baggett, Medina, and Merrill which is in the same spirit of Theorem 1.3. They prove in [Reference Baggett, Medina and Merrill5] that if
![]() $f\in L^1_0(S^1)$
is not a trigonometric polynomial, then the set of irrational rotations of the unit circle
$f\in L^1_0(S^1)$
is not a trigonometric polynomial, then the set of irrational rotations of the unit circle
![]() $S^1$
, for which f is a coboundary with an integrable transfer function, is of first category.
$S^1$
, for which f is a coboundary with an integrable transfer function, is of first category.
2.1 The Halász–Schmidt condition
The following associated condition for measurable dynamics can be found in [Reference Halász25, Reference Schmidt40]. A measurable function f is a coboundary for
![]() $T\in \mathcal {E}$
if and only if for each
$T\in \mathcal {E}$
if and only if for each
![]() $\delta> 0$
, there exists
$\delta> 0$
, there exists
![]() such that for
such that for
![]() ,
,
 $$ \begin{align} \mu \bigg( \bigg\{ x \in X : \bigg| \sum_{i=0}^{n-1} f (T^i x ) \bigg| \leq M_{\delta} \bigg\} \bigg)> 1 - \delta. \end{align} $$
$$ \begin{align} \mu \bigg( \bigg\{ x \in X : \bigg| \sum_{i=0}^{n-1} f (T^i x ) \bigg| \leq M_{\delta} \bigg\} \bigg)> 1 - \delta. \end{align} $$
This condition will be used in §5 to show for any measurable function f that is essentially non-zero, then the class of ergodic invertible measure-preserving transformations T such that
![]() $f = g - g\circ T$
has a measurable solution g is meager (first category). Anosov [Reference Anosov4, Theorem 1] demonstrated that there are no measurable solutions g in the case that f is integrable and
$f = g - g\circ T$
has a measurable solution g is meager (first category). Anosov [Reference Anosov4, Theorem 1] demonstrated that there are no measurable solutions g in the case that f is integrable and
![]() $\int _{X} f\,d\mu \neq 0$
. However, our category results apply in the situation that
$\int _{X} f\,d\mu \neq 0$
. However, our category results apply in the situation that
![]() $\int _{X} f\,d\mu = 0$
.
$\int _{X} f\,d\mu = 0$
.
2.2 Non-measurable solutions
Using the axiom of choice, we can always obtain a solution g. Partition X into orbits (mod measure zero). For each orbit
![]() $\mathcal {O}$
, choose a single point
$\mathcal {O}$
, choose a single point
![]() $x_0 \in \mathcal {O}$
. The coboundary equation leads to the following telescoping series, for
$x_0 \in \mathcal {O}$
. The coboundary equation leads to the following telescoping series, for
![]() $n> 0$
,
$n> 0$
,
 $$ \begin{align*} g( T^n x ) = g(x) - \sum_{i=0}^{n-1} f ( T^i x ) , \end{align*} $$
$$ \begin{align*} g( T^n x ) = g(x) - \sum_{i=0}^{n-1} f ( T^i x ) , \end{align*} $$
and for backward iterates,
 $$ \begin{align*} g( T^{-n} x ) = g(x) + \sum_{i=1}^{n} f ( T^{-i} x ). \end{align*} $$
$$ \begin{align*} g( T^{-n} x ) = g(x) + \sum_{i=1}^{n} f ( T^{-i} x ). \end{align*} $$
If we define
![]() $g(x_0) = 0$
, then the recursion formulas above uniquely determine g at all points along the orbit of
$g(x_0) = 0$
, then the recursion formulas above uniquely determine g at all points along the orbit of
![]() $x_0$
. It is easily checked that
$x_0$
. It is easily checked that
![]() $f(y)=g(y)-g(Ty)$
on the orbit of
$f(y)=g(y)-g(Ty)$
on the orbit of
![]() $x_0$
. By invoking the Axiom of Choice to select a point on each orbit, g is defined at a.e.
$x_0$
. By invoking the Axiom of Choice to select a point on each orbit, g is defined at a.e.
![]() $x\in X$
. However, the result of Anosov implies this g is not measurable when f has a non-zero integral.
$x\in X$
. However, the result of Anosov implies this g is not measurable when f has a non-zero integral.
Here is another case where this construction clearly leads to a non-measurable solution. Suppose
![]() $\alpha $
is irrational and
$\alpha $
is irrational and
![]() $0 < \alpha < 1$
. Define f on
$0 < \alpha < 1$
. Define f on
![]() $[0,1]$
by
$[0,1]$
by
 $$ \begin{align*} f(x)= \begin{cases} \alpha, & \textrm{if}\ x \leq \dfrac{1}{1+\alpha}, \\[4pt] - 1 & \textrm{if}\ x> \dfrac{1}{1+\alpha}. \end{cases} \end{align*} $$
$$ \begin{align*} f(x)= \begin{cases} \alpha, & \textrm{if}\ x \leq \dfrac{1}{1+\alpha}, \\[4pt] - 1 & \textrm{if}\ x> \dfrac{1}{1+\alpha}. \end{cases} \end{align*} $$
The integral of f is zero. Since
![]() $g(x) = 0$
for a single point in each orbit, then the space X equals the following disjoint union (modulo measure zero sets),
$g(x) = 0$
for a single point in each orbit, then the space X equals the following disjoint union (modulo measure zero sets),
 $$ \begin{align*} \bigcup_{i = -\infty}^{\infty} T^i ( \{ x\in X : g(x) = 0 \} ). \end{align*} $$
$$ \begin{align*} \bigcup_{i = -\infty}^{\infty} T^i ( \{ x\in X : g(x) = 0 \} ). \end{align*} $$
Since T is measure preserving, the set
![]() $\{ x\in X : g(x) = 0 \}$
is not measurable and consequently, g is not measurable.
$\{ x\in X : g(x) = 0 \}$
is not measurable and consequently, g is not measurable.
There are cases where it is known that the coboundary equation has no measurable solution g. It was pointed out in [Reference Halász25] that if f is a non-trivial mean-zero step function taking on two values, then the transformation T must have a non-trivial eigenvalue. Thus, if T is weakly mixing and f is a two-step function, there is no measurable solution g. This implies for a two-step mean-zero non-zero function, the ergodic invertible measure preserving transformation obtained in Theorem 1.1 is never weakly mixing.
In [Reference Guenais and Parreau24], given an irrational rotation T, the authors give necessary and sufficient conditions for a step function
![]() $\phi $
and
$\phi $
and
![]() for there to exist measurable solutions to the multiplicative cohomological equation:
for there to exist measurable solutions to the multiplicative cohomological equation:
This result is critical in the study of eigenvalues of interval exchange transformations.
2.3 Bounded coboundaries
This raises the question of when do solutions exist for classes of measurable functions f, when T is allowed to range over
![]() $\mathcal {E}$
. In [Reference Adams and Rosenblatt2] (Theorem 11.2), it is shown that any finite step, mean-zero function is a coboundary for some ergodic invertible measure-preserving transformation with a bounded transfer function g. In particular, T may be chosen in one of the following categories:
$\mathcal {E}$
. In [Reference Adams and Rosenblatt2] (Theorem 11.2), it is shown that any finite step, mean-zero function is a coboundary for some ergodic invertible measure-preserving transformation with a bounded transfer function g. In particular, T may be chosen in one of the following categories:
-
(1) T is a transformation with a discrete spectrum;
-
(2) T is a product of rotations;
-
(3) T is a finite extension of a product of rotations.
Also, Theorems 11.2 and 12.1 in [Reference Adams and Rosenblatt2] show the existence of solutions is extended to mean-zero bounded functions. The case of general
![]() $L^p_0$
functions is more subtle and addressed in this paper.
$L^p_0$
functions is more subtle and addressed in this paper.
The paper [Reference Kwapień32] partially addresses the case of bounded coboundaries. However, the arguments given in [Reference Kwapień32] are viewed as containing a gap, and the main theorem does not apply in general beyond the case of continuous functions f.
2.4 Operator viewpoint
The coboundary equation has been viewed from the perspective of operator theory. Note that the coboundary equation may be written as
where
![]() $U_T$
is the Koopman operator defined by
$U_T$
is the Koopman operator defined by
![]() $U_T(g) = g\circ T$
, and I represents the identity operator. Study of the operator
$U_T(g) = g\circ T$
, and I represents the identity operator. Study of the operator
![]() $( I - T )$
when T is a linear operator (and not necessarily unitary) goes back to the
$( I - T )$
when T is a linear operator (and not necessarily unitary) goes back to the
![]() $19^{th}$
century [Reference Neumann35]. Similar to the case of real or complex numbers, for an operator T with norm
$19^{th}$
century [Reference Neumann35]. Similar to the case of real or complex numbers, for an operator T with norm
![]() $| T | < 1$
, then
$| T | < 1$
, then
![]() $I - T$
has an inverse and
$I - T$
has an inverse and
 $$ \begin{align*} ( I - T )^{-1} = \sum_{i=0}^{\infty} T^k. \end{align*} $$
$$ \begin{align*} ( I - T )^{-1} = \sum_{i=0}^{\infty} T^k. \end{align*} $$
However, for measure-preserving transformations,
![]() $| U_T | = 1$
, and solving
$| U_T | = 1$
, and solving
![]() $f = (I - U_T) g$
becomes more complicated. Browder [Reference Browder8] provided the following equivalent condition for a given contraction T on a reflexive Banach space E. The function
$f = (I - U_T) g$
becomes more complicated. Browder [Reference Browder8] provided the following equivalent condition for a given contraction T on a reflexive Banach space E. The function
![]() $f \in (I - T)E$
, if and only if
$f \in (I - T)E$
, if and only if
 $$ \begin{align*} \sup_{n} \bigg\| \sum_{i=0}^{n-1} T^i f \bigg\| < \infty. \end{align*} $$
$$ \begin{align*} \sup_{n} \bigg\| \sum_{i=0}^{n-1} T^i f \bigg\| < \infty. \end{align*} $$
A two-dimensional version of Browder’s result was proved by Cohen and Lin in [Reference Cohen and Lin10].
Iterative techniques were given in [Reference Dotson13–Reference Dotson15, Reference Groetsch23] as an aid for solving the coboundary equation in this setting. The paper [Reference Lin and Sine33] of Lin and Sine shows that for a given T, when a solution exists, it may be obtained in closed form as the following point-wise limit almost everywhere:
 $$ \begin{align*} g(x) = \lim_{n\to \infty} \frac{1}{n} \sum_{k=1}^{n} \sum_{i=0}^{n-1} f ( T^i x ). \end{align*} $$
$$ \begin{align*} g(x) = \lim_{n\to \infty} \frac{1}{n} \sum_{k=1}^{n} \sum_{i=0}^{n-1} f ( T^i x ). \end{align*} $$
Also, the authors extend their results from the classical Poisson equation,
![]() $f = (I - U_T) g$
to the case of fractional coboundaries [Reference Derriennic and Lin12]. Their main results produce equivalent conditions for solutions to occur for fixed T. Also, in the case of a unitary operator U on a Hilbert space H, a result from [Reference Kozma and Lev31] shows that
$f = (I - U_T) g$
to the case of fractional coboundaries [Reference Derriennic and Lin12]. Their main results produce equivalent conditions for solutions to occur for fixed T. Also, in the case of a unitary operator U on a Hilbert space H, a result from [Reference Kozma and Lev31] shows that
![]() $f \in ( I-U )H$
, if and only if
$f \in ( I-U )H$
, if and only if
 $$ \begin{align*} \sup_{N} \frac{1}{N} \sum_{n=1}^{N} \bigg\| \sum_{k=0}^{n-1} U^k f \bigg\|^2 < \infty. \end{align*} $$
$$ \begin{align*} \sup_{N} \frac{1}{N} \sum_{n=1}^{N} \bigg\| \sum_{k=0}^{n-1} U^k f \bigg\|^2 < \infty. \end{align*} $$
Also, of note, the authors prove in [Reference el Abdalaoui, El Machkouri and Nogueira16] that the following condition is equivalent to T being weak mixing:

Our main results can be recast in terms of operators in the following way.
Corollary 2.1. (Operator theoretic statement of Theorems 1.1 and 1.2)
Let
![]() $(X, {\mathcal B}, \mu )$
be a standard probability space and
$(X, {\mathcal B}, \mu )$
be a standard probability space and
![]() $\mathcal {E}$
be the set of all ergodic invertible measure-preserving transformations on
$\mathcal {E}$
be the set of all ergodic invertible measure-preserving transformations on
![]() $(X, {\mathcal B}, \mu )$
. Then Theorems 1.1 and 1.2 are equivalent to the following statements respectively:
$(X, {\mathcal B}, \mu )$
. Then Theorems 1.1 and 1.2 are equivalent to the following statements respectively:
and
 $$ \begin{align*} L^p_0 \cap \bigcup_{T\in \mathcal{E}} \bigcup_{q> p - 1} ( I - U_T ) ( L^{q} ) \ \textrm{is meager in}\ \ L^p_0. \end{align*} $$
$$ \begin{align*} L^p_0 \cap \bigcup_{T\in \mathcal{E}} \bigcup_{q> p - 1} ( I - U_T ) ( L^{q} ) \ \textrm{is meager in}\ \ L^p_0. \end{align*} $$
2.5 Ergodic averages
One of the main applications of coboundary solutions is to find functions for which the ergodic averages are controlled and converge rapidly. Kachurovskii observed in [Reference Kachurovskii26] that the cohomology equation has a solution if and only if the rate of convergence in the Birkhoff theorem [Reference Birkhoff7] is the highest possible rate and convergence is uniform. We observe, in the case where f is a coboundary for T with integrable transfer function g, all moving averages
![]() $(v_n, L_n)$
converge pointwise for an increasing sequence
$(v_n, L_n)$
converge pointwise for an increasing sequence
![]() ,
,
 $$ \begin{align*} \frac{1}{L_n} \sum_{i=1}^{L_n} f ( T^{v_n + i} x ) \to 0. \end{align*} $$
$$ \begin{align*} \frac{1}{L_n} \sum_{i=1}^{L_n} f ( T^{v_n + i} x ) \to 0. \end{align*} $$
A proof of this fact is given in Appendix B. Other results [Reference Volny and Weiss44] characterize the rate of convergence in measure of ergodic averages of
![]() $L^{\infty }$
functions using uniform approximation by coboundaries where the transfer function lands in a specific
$L^{\infty }$
functions using uniform approximation by coboundaries where the transfer function lands in a specific
![]() $L^p$
space. For
$L^p$
space. For
![]() $p\geq 1$
, the rate is of the order of
$p\geq 1$
, the rate is of the order of
![]() $n^{-p}$
. For stationary processes exhibiting randomness (e.g., positive entropy, random fields), there is a technique for decomposing the process into coboundary and martingale components. See [Reference Giraudo18–Reference Gordin20, Reference Korepanov, Kosloff and Melbourne29, Reference Volny43] and the references contained therein for background on this technique and its applications. This has made it possible to establish common statistical laws (central limit theorem, weak invariance principle) in these cases.
$n^{-p}$
. For stationary processes exhibiting randomness (e.g., positive entropy, random fields), there is a technique for decomposing the process into coboundary and martingale components. See [Reference Giraudo18–Reference Gordin20, Reference Korepanov, Kosloff and Melbourne29, Reference Volny43] and the references contained therein for background on this technique and its applications. This has made it possible to establish common statistical laws (central limit theorem, weak invariance principle) in these cases.
2.6 Non-singular transformations
There is also extensive research on the connections of coboundaries to non-singular transformations. We do not discuss this in detail, but encourage the interested reader to check [Reference Aaronson1, Reference Danilenko and Silva11] for its connections, including the existence of equivalent finite or sigma-finite invariant measures. Techniques developed from the operator theoretic viewpoint can also be applied to non-singular transformations including [Reference Lin and Sine33], and [Reference Alonso, Hong and Obaya3], which develops a different approach for addressing
![]() $L^p$
integrability constraints on solutions.
$L^p$
integrability constraints on solutions.
3 Coboundary existence theorem
In this section, we prove Theorem 1.1, although it is restated here in an equivalent form. We will also show later that this is generally the best possible result.
Theorem 3.1. Let
![]() be such that
be such that
![]() $p \geq 1$
. Suppose
$p \geq 1$
. Suppose
![]() $(X,{\mathcal B},\mu )$
is a standard probability space and
$(X,{\mathcal B},\mu )$
is a standard probability space and
![]() $f \in L^p_0(X,\mu )$
. There exist an ergodic invertible measure-preserving transformation T and a function
$f \in L^p_0(X,\mu )$
. There exist an ergodic invertible measure-preserving transformation T and a function
![]() $g \in L^{p-1}(X,\mu )$
such that
$g \in L^{p-1}(X,\mu )$
such that
![]() $f(x) = g(Tx) - g(x)$
for almost every
$f(x) = g(Tx) - g(x)$
for almost every
![]() $x\in X$
.
$x\in X$
.
For the case of
![]() $L^\infty $
, this theorem follows from Theorems 11.2 and 12.1 in [Reference Adams and Rosenblatt2]. However, [Reference Adams and Rosenblatt2] did not handle unbounded functions.
$L^\infty $
, this theorem follows from Theorems 11.2 and 12.1 in [Reference Adams and Rosenblatt2]. However, [Reference Adams and Rosenblatt2] did not handle unbounded functions.
To prove the existence result for unbounded functions, we will give a construction which reduces the problem to bounded functions. A geometric tower of sets is constructed based loosely on the method of cutting and stacking. (The proof of Theorem 1.2 is not based on cutting and stacking, although it is constructive in nature.) See [Reference Friedman17] for a guide to the cutting and stacking method in ergodic theory. Also, we will use a connection between coboundaries for an induced transformation and the coboundary for the full transformation. In addition to looking at induced transformations, we obtain our limiting transformation by iteratively gluing together an ensemble of transformations defined on subsets (towers) of the full space.
3.1 Induced transformations and coboundaries
In this section, we show how to extend a coboundary for an induced transformation to a coboundary for the full transformation. Let
![]() $T: X \to X$
be an ergodic measure-preserving transformation. Let
$T: X \to X$
be an ergodic measure-preserving transformation. Let
![]() $A \subset X$
be a set of positive measure. Suppose
$A \subset X$
be a set of positive measure. Suppose
where
![]() $n_A(x)$
is the smallest positive integer n such that
$n_A(x)$
is the smallest positive integer n such that
![]() $T^n x\in A$
. Note,
$T^n x\in A$
. Note,
![]() $n_A(x)$
is defined (and finite) for a.e.
$n_A(x)$
is defined (and finite) for a.e.
![]() $x\in A$
since the measure
$x\in A$
since the measure
![]() $\mu $
is not atomic. This defines the so-called induced transformation on A. See [Reference Friedman17, p. 9, Theorem 1.18] or [Reference Petersen36] for further details on induced transformations. Given measurable function
$\mu $
is not atomic. This defines the so-called induced transformation on A. See [Reference Friedman17, p. 9, Theorem 1.18] or [Reference Petersen36] for further details on induced transformations. Given measurable function
![]() and
and
![]() $x \in A$
, define
$x \in A$
, define
 $$ \begin{align*} f_A (x) = \sum_{i=0}^{n_A(x) - 1} f ( T^i x ). \end{align*} $$
$$ \begin{align*} f_A (x) = \sum_{i=0}^{n_A(x) - 1} f ( T^i x ). \end{align*} $$
We have the following lemma which will be used to prove Theorem 3.1.
Lemma 3.2. Let
![]() be a measurable function and
be a measurable function and
![]() $T:X\to X$
be an ergodic measure-preserving invertible transformation. Suppose
$T:X\to X$
be an ergodic measure-preserving invertible transformation. Suppose
![]() $f_A$
is a coboundary for induced transformation
$f_A$
is a coboundary for induced transformation
![]() $T_A:A\to A$
with transfer function
$T_A:A\to A$
with transfer function
![]() $g_A$
such that
$g_A$
such that
![]() $f_A = g_A \circ T_A - g_A$
. Given
$f_A = g_A \circ T_A - g_A$
. Given
![]() $z\in X\setminus A$
, define
$z\in X\setminus A$
, define
![]() and for
and for
![]() $z \in A$
,
$z \in A$
,
![]() $j_z = 0$
. Since T is ergodic and invertible, then
$j_z = 0$
. Since T is ergodic and invertible, then
![]() $j_z < \infty $
for a.e.
$j_z < \infty $
for a.e.
![]() $z \in X$
and in particular,
$z \in X$
and in particular,
![]() $0 \leq j_z \leq n_A(T^{-j_z}z)$
almost everywhere. Then f is a coboundary for transformation T with transfer function g defined such that for
$0 \leq j_z \leq n_A(T^{-j_z}z)$
almost everywhere. Then f is a coboundary for transformation T with transfer function g defined such that for
![]() $z \in A$
,
$z \in A$
,
![]() $g(z) = g_A(z)$
and for a.e.
$g(z) = g_A(z)$
and for a.e.
![]() $z \in X\setminus A$
,
$z \in X\setminus A$
,
 $$ \begin{align*} g( z ) = g_A ( T^{-j_z}z ) + \sum_{i=0}^{j_z-1} f ( T^{i-j_z}z ). \end{align*} $$
$$ \begin{align*} g( z ) = g_A ( T^{-j_z}z ) + \sum_{i=0}^{j_z-1} f ( T^{i-j_z}z ). \end{align*} $$
Proof. For a.e.
![]() $z \in X$
, let
$z \in X$
, let
![]() $x = T^{-j_z}z$
. If
$x = T^{-j_z}z$
. If
![]() $0\leq j_z < n_A(x) - 1$
, then
$0\leq j_z < n_A(x) - 1$
, then
 $$ \begin{align*} g ( T z ) - g ( z ) = g_A(x) + \sum_{i=0}^{j_z} f ( T^i x ) - g_A(x) - \sum_{i=0}^{j_z-1} f ( T^i x ) = f ( T^{j_z} x ) = f(z). \end{align*} $$
$$ \begin{align*} g ( T z ) - g ( z ) = g_A(x) + \sum_{i=0}^{j_z} f ( T^i x ) - g_A(x) - \sum_{i=0}^{j_z-1} f ( T^i x ) = f ( T^{j_z} x ) = f(z). \end{align*} $$
Now suppose
![]() $j_z = n_A(x) - 1$
. Then
$j_z = n_A(x) - 1$
. Then
 $$ \begin{align} g ( T z ) - g ( z ) &= g_A ( T_A x ) - \bigg( g_A ( x ) + \sum_{i=0}^{n_A(x)-2} f ( T^i x ) \bigg) \end{align} $$
$$ \begin{align} g ( T z ) - g ( z ) &= g_A ( T_A x ) - \bigg( g_A ( x ) + \sum_{i=0}^{n_A(x)-2} f ( T^i x ) \bigg) \end{align} $$
 $$ \begin{align} &= f_A ( x ) - \sum_{i=0}^{n_A(x)-2} f ( T^i x ) \end{align} $$
$$ \begin{align} &= f_A ( x ) - \sum_{i=0}^{n_A(x)-2} f ( T^i x ) \end{align} $$
 $$ \begin{align} &= \sum_{i=0}^{n_A(x)-1} f ( T^i x ) - \sum_{i=0}^{n_A(x)-2} f ( T^i x ) \end{align} $$
$$ \begin{align} &= \sum_{i=0}^{n_A(x)-1} f ( T^i x ) - \sum_{i=0}^{n_A(x)-2} f ( T^i x ) \end{align} $$
This proves that f is a coboundary for T with transfer function g for a.e.
![]() $z \in X$
.
$z \in X$
.
3.2 Tower constructions on subsets of the measure space
To construct the transformation and transfer function, we will first decompose the measure space into disjoint subsets and then construct towers on each subset. Using the construction from a previous paper [Reference Adams and Rosenblatt2], we will define the transformation on the top of the towers such that the full transformation is ergodic measure preserving and invertible (that is, it glues all the towers together). This construction will produce a transfer function with the required integrability properties.
Suppose
![]() is a measurable function and
is a measurable function and
![]() $A \subset X$
is a measurable subset. Define
$A \subset X$
is a measurable subset. Define
![]() as the restriction of f to A:
as the restriction of f to A:
For convenience, we may write
![]() $\digamma = f_{|A}$
. To emphasize that a transformation is restricted to a subset A, we use the notation
$\digamma = f_{|A}$
. To emphasize that a transformation is restricted to a subset A, we use the notation
![]() $T_{|A} : A \to A$
or, for convenience,
$T_{|A} : A \to A$
or, for convenience,
![]() $\tau : A \to A$
. This is distinct from the notion of induced transformation which is written as
$\tau : A \to A$
. This is distinct from the notion of induced transformation which is written as
![]() $T_A$
. Now we prove the following lemma which is a basic building block for the construction of our full transformation T.
$T_A$
. Now we prove the following lemma which is a basic building block for the construction of our full transformation T.
Lemma 3.3. Suppose
![]() $A \subset X$
has positive measure and
$A \subset X$
has positive measure and
![]() takes on two steps with mean zero (that is,
takes on two steps with mean zero (that is,
![]() $\int _{A} \digamma \,d\mu = 0$
, where
$\int _{A} \digamma \,d\mu = 0$
, where
![]() $\digamma = f_{|A}$
). Given
$\digamma = f_{|A}$
). Given
![]() and
and
![]() $\epsilon> 0$
, there exist
$\epsilon> 0$
, there exist
![]() $h_1, h_2> h$
, disjoint
$h_1, h_2> h$
, disjoint
![]() $I_1, I_2 \subseteq A$
, and an invertible measure-preserving map
$I_1, I_2 \subseteq A$
, and an invertible measure-preserving map
![]() $\tau :A\to A$
such that
$\tau :A\to A$
such that
 $$ \begin{align} \mu \bigg( \bigcup_{i=0}^{h_1 -1} \tau^i I_1 \cup \bigcup_{i=0}^{h_2 -1} \tau^i I_2 \bigg) = \mu (A) , \end{align} $$
$$ \begin{align} \mu \bigg( \bigcup_{i=0}^{h_1 -1} \tau^i I_1 \cup \bigcup_{i=0}^{h_2 -1} \tau^i I_2 \bigg) = \mu (A) , \end{align} $$
 $$ \begin{align} \bigg|\sum\limits_{i=0}^{k} \digamma(\tau^i x) \bigg| \leq \| \digamma \|_{\infty}\quad \textrm{for } x\in I_j, k < h_j , j=1,2, \end{align} $$
$$ \begin{align} \bigg|\sum\limits_{i=0}^{k} \digamma(\tau^i x) \bigg| \leq \| \digamma \|_{\infty}\quad \textrm{for } x\in I_j, k < h_j , j=1,2, \end{align} $$
 $$ \begin{align} \bigg| \sum\limits_{i=0}^{h_j -1} \digamma (\tau^i x) \bigg| < \epsilon \quad\textrm{for } x\in I_j , j=1,2, \end{align} $$
$$ \begin{align} \bigg| \sum\limits_{i=0}^{h_j -1} \digamma (\tau^i x) \bigg| < \epsilon \quad\textrm{for } x\in I_j , j=1,2, \end{align} $$
 $$ \begin{align} \sum\limits_{i=0}^{h_j -1} \digamma (\tau^i x) = \sum\limits_{i=0}^{h_j -1} \digamma (\tau^i y) \quad\textrm{for } x, y\in I_j , j=1,2, \end{align} $$
$$ \begin{align} \sum\limits_{i=0}^{h_j -1} \digamma (\tau^i x) = \sum\limits_{i=0}^{h_j -1} \digamma (\tau^i y) \quad\textrm{for } x, y\in I_j , j=1,2, \end{align} $$
Proof. Without loss of generality, assume
![]() $A \subset X = [0,1]$
. Suppose
$A \subset X = [0,1]$
. Suppose
![]() $\digamma = b I_B - c I_C$
is mean zero for
$\digamma = b I_B - c I_C$
is mean zero for
![]() $b, c> 0$
and disjoint
$b, c> 0$
and disjoint
![]() $B, C$
such that
$B, C$
such that
![]() $B \cup C = A$
. The case where
$B \cup C = A$
. The case where
![]() ${b}/{c}$
is rational is straightforward, so we assume
${b}/{c}$
is rational is straightforward, so we assume
![]() ${b} / {c}$
is irrational. There exist
${b} / {c}$
is irrational. There exist
![]() $\delta _1, \delta _2$
of the same sign, and
$\delta _1, \delta _2$
of the same sign, and
![]() $p_1, q_1, p_2, q_2$
such that
$p_1, q_1, p_2, q_2$
such that
![]() $| \delta _2 | < | \delta _1 | < \epsilon $
,
$| \delta _2 | < | \delta _1 | < \epsilon $
,
![]() $p_1 < \epsilon p_2$
,
$p_1 < \epsilon p_2$
,
![]() $q_1 < \epsilon q_2$
,
$q_1 < \epsilon q_2$
,
![]() $p_2 + q_2 - p_1 - q_1> h$
,
$p_2 + q_2 - p_1 - q_1> h$
,
![]() $p_1 b - q_1 c = \delta _1$
, and
$p_1 b - q_1 c = \delta _1$
, and
![]() $p_2 b - q_2 c = \delta _2$
. Without loss of generality, assume
$p_2 b - q_2 c = \delta _2$
. Without loss of generality, assume
![]() $0 < \delta _2 < \delta _1 < \epsilon $
. The case where
$0 < \delta _2 < \delta _1 < \epsilon $
. The case where
![]() $\delta _1, \delta _2$
are negative follows similarly. Let
$\delta _1, \delta _2$
are negative follows similarly. Let
![]() $p_3 = p_2 - p_1$
and
$p_3 = p_2 - p_1$
and
![]() $q_3 = q_2 - q_1$
. Note,
$q_3 = q_2 - q_1$
. Note,
Let
![]() $\delta _3 = \delta _1 - \delta _2$
. Split B into two disjoint sets
$\delta _3 = \delta _1 - \delta _2$
. Split B into two disjoint sets
![]() $B_1, B_2$
such that
$B_1, B_2$
such that
Note,
Similarly, split
![]() $C = C_1 \cup C_2$
such that
$C = C_1 \cup C_2$
such that
Divide
![]() $B_1$
into
$B_1$
into
![]() $p_2$
disjoint equimeasurable sets
$p_2$
disjoint equimeasurable sets
![]() $B_{1,j}$
for
$B_{1,j}$
for
![]() $j \in \{ 1, 2, \ldots , p_2 \}$
. Divide
$j \in \{ 1, 2, \ldots , p_2 \}$
. Divide
![]() $C_1$
into
$C_1$
into
![]() $q_2$
disjoint equimeasurable sets
$q_2$
disjoint equimeasurable sets
![]() $C_{1,j}$
for
$C_{1,j}$
for
![]() $j \in \{ 1, 2, \ldots , q_2 \}$
. Divide
$j \in \{ 1, 2, \ldots , q_2 \}$
. Divide
![]() $B_2$
into
$B_2$
into
![]() $p_3$
disjoint equimeasurable sets
$p_3$
disjoint equimeasurable sets
![]() $B_{2,j}$
for
$B_{2,j}$
for
![]() $j \in \{ 1, 2, \ldots , p_3 \}$
. Divide
$j \in \{ 1, 2, \ldots , p_3 \}$
. Divide
![]() $C_2$
into
$C_2$
into
![]() $q_3$
disjoint equimeasurable sets
$q_3$
disjoint equimeasurable sets
![]() $C_{2,j}$
for
$C_{2,j}$
for
![]() $j \in \{ 1, 2, \ldots , q_3 \}$
. Thus,
$j \in \{ 1, 2, \ldots , q_3 \}$
. Thus,
![]() $\mu (C_{1, j}) = \mu (B_{1,k})$
for
$\mu (C_{1, j}) = \mu (B_{1,k})$
for
![]() $j \in \{ 1,2, \ldots , p_2 \}$
and
$j \in \{ 1,2, \ldots , p_2 \}$
and
![]() $k \in \{ 1,2, \ldots , q_2 \}$
. Also,
$k \in \{ 1,2, \ldots , q_2 \}$
. Also,
![]() $\mu (C_{2, j}) = \mu (B_{2,k})$
for
$\mu (C_{2, j}) = \mu (B_{2,k})$
for
![]() $j \in \{ 1,2, \ldots , p_3 \}$
and
$j \in \{ 1,2, \ldots , p_3 \}$
and
![]() $k \in \{ 1,2, \ldots , q_3 \}$
. Let
$k \in \{ 1,2, \ldots , q_3 \}$
. Let
![]() $I_1 = B_{1,1}$
and
$I_1 = B_{1,1}$
and
![]() $I_2 = B_{2,1}$
. Stack the sets
$I_2 = B_{2,1}$
. Stack the sets
![]() $B_{1,j}$
and
$B_{1,j}$
and
![]() $C_{1,k}$
such that whenever the sum of the values is negative, place a B next, and otherwise place a C set next. Stack the sets
$C_{1,k}$
such that whenever the sum of the values is negative, place a B next, and otherwise place a C set next. Stack the sets
![]() $B_{2,j}$
and
$B_{2,j}$
and
![]() $C_{2,k}$
such that whenever the sum of the values is negative, place a B next, and otherwise place a C set next. As long as
$C_{2,k}$
such that whenever the sum of the values is negative, place a B next, and otherwise place a C set next. As long as
![]() $\delta _1 < { \min { \{ c, b \} } }/{2}$
, then we have the precise number of level sets B and C to complete the two towers. In this case, let
$\delta _1 < { \min { \{ c, b \} } }/{2}$
, then we have the precise number of level sets B and C to complete the two towers. In this case, let
![]() $h_1 = p_2 + q_2$
and
$h_1 = p_2 + q_2$
and
![]() $h_2 = p_3 + q_3$
. Thus, the condition
$h_2 = p_3 + q_3$
. Thus, the condition
![]() $h_2 = p_3 + q_3 = p_2 - p_1 + q_2 - q_1> h$
implies
$h_2 = p_3 + q_3 = p_2 - p_1 + q_2 - q_1> h$
implies
![]() $h_1 = p_2 + q_2> h$
.
$h_1 = p_2 + q_2> h$
.
For
![]() $x \in I_1$
,
$x \in I_1$
,
 $$ \begin{align*} \bigg| \sum_{i=0}^{h_1 - 1} \digamma ( \tau^i x ) \bigg| = | p_2 b - q_2 c | = \delta_2 < \epsilon , \end{align*} $$
$$ \begin{align*} \bigg| \sum_{i=0}^{h_1 - 1} \digamma ( \tau^i x ) \bigg| = | p_2 b - q_2 c | = \delta_2 < \epsilon , \end{align*} $$
and for
![]() $x \in I_2$
,
$x \in I_2$
,
 $$ \begin{align*} \bigg| \sum_{i=0}^{h_2 - 1} \digamma ( \tau^i x ) \bigg| = | p_3 b - q_3 c | = \delta_1 - \delta_2 < \epsilon. \end{align*} $$
$$ \begin{align*} \bigg| \sum_{i=0}^{h_2 - 1} \digamma ( \tau^i x ) \bigg| = | p_3 b - q_3 c | = \delta_1 - \delta_2 < \epsilon. \end{align*} $$
Equation (3.7) holds due to the greedy stacking algorithm used. The other conditions in the lemma hold by construction.
Lemma 3.4. Suppose
![]() $A\subset X$
is a set of positive measure and
$A\subset X$
is a set of positive measure and
![]() is a mean-zero, non-zero finite step function. Explicitly, let
is a mean-zero, non-zero finite step function. Explicitly, let
![]() , where
, where
![]() $A = \bigcup _{i=1}^{m} I_i$
is a disjoint union of sets
$A = \bigcup _{i=1}^{m} I_i$
is a disjoint union of sets
![]() $I_i$
of positive measure, and
$I_i$
of positive measure, and
![]() $a_i$
are distinct real numbers for
$a_i$
are distinct real numbers for
![]() $1\leq i \leq m$
and
$1\leq i \leq m$
and
![]() $m \geq 2$
. There exist disjoint measurable sets
$m \geq 2$
. There exist disjoint measurable sets
![]() $J_1, J_2, \ldots , J_{m-1}$
such that
$J_1, J_2, \ldots , J_{m-1}$
such that
![]() $\digamma $
takes on at most two values almost everywhere on
$\digamma $
takes on at most two values almost everywhere on
![]() $J_i$
and
$J_i$
and
![]() $\int _{J_i} \digamma \,d\mu = 0$
for
$\int _{J_i} \digamma \,d\mu = 0$
for
![]() $1\leq i \leq m-1$
.
$1\leq i \leq m-1$
.
Proof. We prove this by induction on m. Clearly, this is true for
![]() $m=2$
. Suppose it is true for
$m=2$
. Suppose it is true for
![]() $m=n$
and all finite measure spaces. Let
$m=n$
and all finite measure spaces. Let
![]() $m = n + 1$
. Choose j such that for
$m = n + 1$
. Choose j such that for
![]() $1\leq i \leq n+1$
,
$1\leq i \leq n+1$
,
 $$ \begin{align*} \int_{I_j} | \digamma |\,d\mu = | a_j | \mu ( I_j ) \leq \int_{I_i} | \digamma |\,d\mu = | a_i | \mu ( I_i ). \end{align*} $$
$$ \begin{align*} \int_{I_j} | \digamma |\,d\mu = | a_j | \mu ( I_j ) \leq \int_{I_i} | \digamma |\,d\mu = | a_i | \mu ( I_i ). \end{align*} $$
If
![]() $a_j \leq 0$
, choose
$a_j \leq 0$
, choose
![]() $k \neq j$
such that
$k \neq j$
such that
![]() $a_k \geq 0$
, otherwise choose k such that
$a_k \geq 0$
, otherwise choose k such that
![]() $a_k \leq 0$
. Choose
$a_k \leq 0$
. Choose
![]() $I^{\prime } \subset I_k$
such that
$I^{\prime } \subset I_k$
such that
Define
![]() $J_n = I_j \cup I^{\prime }$
. Thus,
$J_n = I_j \cup I^{\prime }$
. Thus,
![]() $\digamma $
takes on at most n steps on the subset
$\digamma $
takes on at most n steps on the subset
![]() $A \setminus J_n$
. By induction, there exist
$A \setminus J_n$
. By induction, there exist
![]() $J_1, J_2, \ldots , J_{n-1}$
such that
$J_1, J_2, \ldots , J_{n-1}$
such that
![]() $\digamma $
takes on at most two steps on
$\digamma $
takes on at most two steps on
![]() $J_i$
. Therefore, our lemma is proved by induction.
$J_i$
. Therefore, our lemma is proved by induction.
The next lemma uses the notion of a TUB tower which was defined in [Reference Adams and Rosenblatt2]. For completeness, we present the definition here. The proof of the following Lemma 3.5 uses Lemma A.2 which is stated and proved in Appendix A.2.
Let A be a measurable subset of X and
![]() a bounded, mean-zero function. Given a finite measurable partition Q,
a bounded, mean-zero function. Given a finite measurable partition Q,
![]() , and
, and
![]() $\epsilon> 0$
, an
$\epsilon> 0$
, an
![]() $\epsilon $
-balanced and uniform tower for f is a set of disjoint measurable sets
$\epsilon $
-balanced and uniform tower for f is a set of disjoint measurable sets
![]() $I_i\subset A$
for
$I_i\subset A$
for
![]() $i=1,2,\ldots ,h$
and an invertible measure-preserving map
$i=1,2,\ldots ,h$
and an invertible measure-preserving map
![]() $T: I_i \to I_{i+1}$
for
$T: I_i \to I_{i+1}$
for
![]() $i=1,2,\ldots ,h-1$
, such that
$i=1,2,\ldots ,h-1$
, such that
 $$ \begin{align} \mu \bigg(\bigcup_{i=1}^h I_i\bigg) > \mu (A) - \epsilon ,\end{align} $$
$$ \begin{align} \mu \bigg(\bigcup_{i=1}^h I_i\bigg) > \mu (A) - \epsilon ,\end{align} $$
 $$ \begin{align} \bigg|\sum\limits_{i=0}^{k} f(T^i x) \bigg| < \| f \|_{\infty} + \epsilon \quad\textrm{for } x\in I_1, k < h , \end{align} $$
$$ \begin{align} \bigg|\sum\limits_{i=0}^{k} f(T^i x) \bigg| < \| f \|_{\infty} + \epsilon \quad\textrm{for } x\in I_1, k < h , \end{align} $$
 $$ \begin{align} \sum_{i=1}^{h} \int_{I_i} f\,d\mu = \int_{A} f\,d\mu , \end{align} $$
$$ \begin{align} \sum_{i=1}^{h} \int_{I_i} f\,d\mu = \int_{A} f\,d\mu , \end{align} $$
 $$ \begin{align} \bigg| \sum\limits_{i=0}^{h-1} f(T^i x) \bigg| < \epsilon \quad\textrm{for } x\in I_1 , \end{align} $$
$$ \begin{align} \bigg| \sum\limits_{i=0}^{h-1} f(T^i x) \bigg| < \epsilon \quad\textrm{for } x\in I_1 , \end{align} $$
 $$ \begin{align} \textrm{for each } q\in Q, \text{there exists } \mathcal{I} \subset \{ 1,\ldots ,h \} \textrm{ such that } \mu \bigg( q \triangle \bigg( \bigcup_{i \in \mathcal{I}} I_i \bigg) \bigg) < \epsilon. \end{align} $$
$$ \begin{align} \textrm{for each } q\in Q, \text{there exists } \mathcal{I} \subset \{ 1,\ldots ,h \} \textrm{ such that } \mu \bigg( q \triangle \bigg( \bigcup_{i \in \mathcal{I}} I_i \bigg) \bigg) < \epsilon. \end{align} $$
We refer to this type of tower as a TUB
![]() $(\epsilon , h, Q)$
tower for
$(\epsilon , h, Q)$
tower for
![]() $f_{|A}$
.
$f_{|A}$
.
Lemma 3.5. Suppose
![]() $A\subset X$
is a set of positive measure and
$A\subset X$
is a set of positive measure and
![]() is bounded and mean zero. Given
is bounded and mean zero. Given
![]() $\epsilon _i> 0$
for
$\epsilon _i> 0$
for
![]() such that
such that
![]() $\lim _{i\to \infty } \epsilon _i = 0$
, there exist an invertible measure-preserving map T, disjoint sets
$\lim _{i\to \infty } \epsilon _i = 0$
, there exist an invertible measure-preserving map T, disjoint sets
![]() $I_i \subset A$
and natural numbers
$I_i \subset A$
and natural numbers
![]() $h_i$
such that:
$h_i$
such that:
-
•
 $A = \bigcup _{i=1}^{\infty } \bigcup _{j=0}^{h_i-1} T^j I_i$
is a disjoint union;
$A = \bigcup _{i=1}^{\infty } \bigcup _{j=0}^{h_i-1} T^j I_i$
is a disjoint union; -
•
 $ | \sum _{j=0}^{h_i-1} \digamma ( T^j x ) | < \epsilon _i$
for
$ | \sum _{j=0}^{h_i-1} \digamma ( T^j x ) | < \epsilon _i$
for
 $x\in I_i$
; and
$x\in I_i$
; and -
•
 $ | \sum _{j=0}^{k} \digamma ( T^j x ) | < \| \digamma \|_{\infty } + \epsilon _i$
for
$ | \sum _{j=0}^{k} \digamma ( T^j x ) | < \| \digamma \|_{\infty } + \epsilon _i$
for
 $x\in I_i$
and
$x\in I_i$
and
 $0 \leq k < h_i$
.
$0 \leq k < h_i$
.
Proof. If
![]() $\digamma $
is a finite step function, then the lemma follows by applying Lemmas 3.4 and 3.3 with a finite number of sets
$\digamma $
is a finite step function, then the lemma follows by applying Lemmas 3.4 and 3.3 with a finite number of sets
![]() $J_i$
. If
$J_i$
. If
![]() $\digamma $
is not a finite step function, then we apply Lemma A.2, iteratively and potentially infinitely many times, to construct a sequence of TUB towers that satisfy this lemma. By Lemma A.2, there exist
$\digamma $
is not a finite step function, then we apply Lemma A.2, iteratively and potentially infinitely many times, to construct a sequence of TUB towers that satisfy this lemma. By Lemma A.2, there exist
![]() $I_1 \subset A$
,
$I_1 \subset A$
,
![]() and T which is invertible and measure preserving such that
and T which is invertible and measure preserving such that
![]() $T^{i}(I_1) \subset A$
are disjoint for
$T^{i}(I_1) \subset A$
are disjoint for
![]() $i=0,1,\ldots , h_1-1$
and
$i=0,1,\ldots , h_1-1$
and
![]() $\mu ( \bigcup _{i=0}^{h_1 - 1} T^{i} ( I_1 ) )> \mu (A) - \epsilon _1$
. Also, using Lemma A.2, we can obtain the last two conditions of Lemma 3.5. Let
$\mu ( \bigcup _{i=0}^{h_1 - 1} T^{i} ( I_1 ) )> \mu (A) - \epsilon _1$
. Also, using Lemma A.2, we can obtain the last two conditions of Lemma 3.5. Let
![]() $A_1 = A \setminus \bigcup _{i=0}^{h_1 - 1} T^{i} ( I_1 )$
. In a similar fashion, apply Lemma A.2 to
$A_1 = A \setminus \bigcup _{i=0}^{h_1 - 1} T^{i} ( I_1 )$
. In a similar fashion, apply Lemma A.2 to
![]() $A_1$
using parameter
$A_1$
using parameter
![]() $\epsilon _2$
. Thus, in general,
$\epsilon _2$
. Thus, in general,
 $$ \begin{align*} A_k = A \setminus \bigcup_{j=1}^{k} \bigcup_{i=0}^{h_j-1} T^{i} ( I_j ) \end{align*} $$
$$ \begin{align*} A_k = A \setminus \bigcup_{j=1}^{k} \bigcup_{i=0}^{h_j-1} T^{i} ( I_j ) \end{align*} $$
with
![]() $\mu (A_k) < \epsilon _k \to 0$
as
$\mu (A_k) < \epsilon _k \to 0$
as
![]() $k\to \infty $
and our lemma is satisfied.
$k\to \infty $
and our lemma is satisfied.
Remark. The transformation T constructed in Lemma 3.5 does not need to be defined on all of A. It may not be defined on the top of the towers, and instead,
 $$ \begin{align*} T:A\setminus \bigg( \bigcup_{i=1}^{\infty} T^{h_i-1} I_i \bigg) \to A\setminus \bigg( \bigcup_{i=1}^{\infty} I_i \bigg). \end{align*} $$
$$ \begin{align*} T:A\setminus \bigg( \bigcup_{i=1}^{\infty} T^{h_i-1} I_i \bigg) \to A\setminus \bigg( \bigcup_{i=1}^{\infty} I_i \bigg). \end{align*} $$
The following proposition was previously proved in [Reference Adams and Rosenblatt2]. A direct proof is given in Appendix A.2.
Proposition 3.6. Let
![]() $(X,{\mathcal B},\mu )$
be a standard probability space and
$(X,{\mathcal B},\mu )$
be a standard probability space and
![]() $A \subset X$
a set of positive measure. Suppose
$A \subset X$
a set of positive measure. Suppose
![]() is measurable, mean zero, and bounded. There exist an ergodic measure-preserving transformation
is measurable, mean zero, and bounded. There exist an ergodic measure-preserving transformation
![]() $T:A\to A$
and bounded function g such that
$T:A\to A$
and bounded function g such that
![]() $f = g - g\circ T$
almost everywhere. Moreover, the transformation T and transfer function g may be constructed such that for any
$f = g - g\circ T$
almost everywhere. Moreover, the transformation T and transfer function g may be constructed such that for any
![]() $\delta> 0$
,
$\delta> 0$
,
![]() $\| g \|_{\infty } < \| f \|_{\infty } + \delta $
.
$\| g \|_{\infty } < \| f \|_{\infty } + \delta $
.
3.3 Proof of the main positive result
Now we are ready to proceed with the proof of Theorem 3.1.
Proof. Without loss of generality, we prove this theorem for the case
![]() $X = [0,1)$
and
$X = [0,1)$
and
![]() $\mu $
equal to Lebesgue measure. Also, we may assume
$\mu $
equal to Lebesgue measure. Also, we may assume
![]() $f \notin L^{\infty }$
, since this case was handled previously in [Reference Adams and Rosenblatt2], Proposition 3.6, and also by Ber et al. [Reference Ber, Borst and Sukochev6]. If f does not take on essentially infinitely many bounded values on a compact set, then first apply Lemma 3.3 to generate countable towers and transformation such that the sums are bounded, that is, less than
$f \notin L^{\infty }$
, since this case was handled previously in [Reference Adams and Rosenblatt2], Proposition 3.6, and also by Ber et al. [Reference Ber, Borst and Sukochev6]. If f does not take on essentially infinitely many bounded values on a compact set, then first apply Lemma 3.3 to generate countable towers and transformation such that the sums are bounded, that is, less than
![]() $\epsilon _i$
for the ith tower. Let k be the minimum positive integer such that
$\epsilon _i$
for the ith tower. Let k be the minimum positive integer such that
![]() $\mu ( \{ x: 0 < f(x) \leq k \} )> 0$
, and similarly let
$\mu ( \{ x: 0 < f(x) \leq k \} )> 0$
, and similarly let
![]() $\ell $
be the minimum positive integer such that
$\ell $
be the minimum positive integer such that
![]() $\mu ( \{ x: 0> f(x) \geq -\ell \} ) > 0$
. If no such k and no such
$\mu ( \{ x: 0> f(x) \geq -\ell \} ) > 0$
. If no such k and no such
![]() $\ell $
exist, then f must equal zero almost everywhere, which contradicts our assumption. Let
$\ell $
exist, then f must equal zero almost everywhere, which contradicts our assumption. Let
![]() $X_1 = \{ x: k-1 < f(x) \leq k \} $
and
$X_1 = \{ x: k-1 < f(x) \leq k \} $
and
![]() $Y_1 = \{ x: 1 - \ell> f(x) \geq -\ell \}$
. If
$Y_1 = \{ x: 1 - \ell> f(x) \geq -\ell \}$
. If
![]() $\int _{X_1} f\,d\mu + \int _{Y_1} f\,d\mu \leq 0$
, define
$\int _{X_1} f\,d\mu + \int _{Y_1} f\,d\mu \leq 0$
, define
![]() $Y_1^{\prime } \subseteq Y_1$
such that
$Y_1^{\prime } \subseteq Y_1$
such that
 $$ \begin{align*} \int_{X_1} f\,d\mu + \int_{Y_1^{\prime}} f\,d\mu = 0. \end{align*} $$
$$ \begin{align*} \int_{X_1} f\,d\mu + \int_{Y_1^{\prime}} f\,d\mu = 0. \end{align*} $$
In this case, let
![]() $X_1^{\prime } = X_1$
. Otherwise, choose
$X_1^{\prime } = X_1$
. Otherwise, choose
![]() $X_1^{\prime } \subset X_1$
such that
$X_1^{\prime } \subset X_1$
such that
 $$ \begin{align*} \int_{X_1^{\prime}} f\,d\mu + \int_{Y_1} f\,d\mu = 0. \end{align*} $$
$$ \begin{align*} \int_{X_1^{\prime}} f\,d\mu + \int_{Y_1} f\,d\mu = 0. \end{align*} $$
In this case, set
![]() $Y_1^{\prime } = Y_1$
. Also, define
$Y_1^{\prime } = Y_1$
. Also, define
![]() $k_1 = k$
,
$k_1 = k$
,
![]() $\ell _1 = \ell $
, and
$\ell _1 = \ell $
, and
![]() $X_0 = \{ x: f(x) = 0 \}$
. We may continue this procedure inductively to choose disjoint sets
$X_0 = \{ x: f(x) = 0 \}$
. We may continue this procedure inductively to choose disjoint sets
![]() $X_n^{\prime }, Y_n^{\prime }$
for
$X_n^{\prime }, Y_n^{\prime }$
for
![]() $n=1, 2, \ldots ,$
and sequences of positive integers
$n=1, 2, \ldots ,$
and sequences of positive integers
![]() $k_n, \ell _n$
such that:
$k_n, \ell _n$
such that:
-
(1)
 $k_n - 1 < f(x) \leq k_n$
for
$k_n - 1 < f(x) \leq k_n$
for
 $x \in X_n^{\prime }$
;
$x \in X_n^{\prime }$
; -
(2)
 $1 - \ell _n> f(x) \geq - \ell _n$
for
$1 - \ell _n> f(x) \geq - \ell _n$
for
 $x \in Y_n^{\prime }$
;
$x \in Y_n^{\prime }$
; -
(3)
 $k_{n+1} \geq k_n$
,
$k_{n+1} \geq k_n$
,
 $\ell _{n+1} \geq \ell _n$
;
$\ell _{n+1} \geq \ell _n$
; -
(4)
 $\lim _{n\to \infty } k_n + \ell _n = \infty $
;
$\lim _{n\to \infty } k_n + \ell _n = \infty $
; -
(5)
 $\int _{X_n^{\prime }} f\,d\mu + \int _{Y_n^{\prime }} f\,d\mu = 0$
;
$\int _{X_n^{\prime }} f\,d\mu + \int _{Y_n^{\prime }} f\,d\mu = 0$
; -
(6)
 $\mu ( \bigcup _{n=1}^{\infty } ( X_n^{\prime } \cup Y_n^{\prime } ) ) = \mu (X\setminus X_0)$
.
$\mu ( \bigcup _{n=1}^{\infty } ( X_n^{\prime } \cup Y_n^{\prime } ) ) = \mu (X\setminus X_0)$
.
Item (4) above is true, since
![]() $f \notin L^{\infty }$
. Either
$f \notin L^{\infty }$
. Either
![]() $k_n \to \infty $
or
$k_n \to \infty $
or
![]() $\ell _n \to \infty $
. Assume without loss of generality that
$\ell _n \to \infty $
. Assume without loss of generality that
![]() $k_n \to \infty $
and
$k_n \to \infty $
and
![]() $k_n> \ell _n$
for infinitely many
$k_n> \ell _n$
for infinitely many
![]() . If
. If
![]() $\ell _n = 1$
for all
$\ell _n = 1$
for all
![]() , then we need to be careful about the choice of
, then we need to be careful about the choice of
![]() $Y_n^{\prime }$
. Let
$Y_n^{\prime }$
. Let
![]() $\gamma _0 = 0$
. We can choose a sequence of non-decreasing real numbers
$\gamma _0 = 0$
. We can choose a sequence of non-decreasing real numbers
![]() such that
such that
![]() $0 < \gamma _n \leq 1$
and
$0 < \gamma _n \leq 1$
and
If
![]() $\ell _n$
is eventually greater than 1, then we can set
$\ell _n$
is eventually greater than 1, then we can set
![]() $\gamma _n = \ell _n$
for
$\gamma _n = \ell _n$
for
![]() . In either case, for
. In either case, for
![]() $x\in Y_n^{\prime }$
,
$x\in Y_n^{\prime }$
,
Let
![]() $\epsilon> 0$
. For each
$\epsilon> 0$
. For each
![]() , apply Lemma 3.5 to f defined on
, apply Lemma 3.5 to f defined on
![]() $Z_n = X_n^{\prime } \cup Y_n^{\prime }$
to obtain a decomposition into potentially infinitely many towers satisfying the conditions of Lemma 3.5. Thus, there exists an invertible measure-preserving
$Z_n = X_n^{\prime } \cup Y_n^{\prime }$
to obtain a decomposition into potentially infinitely many towers satisfying the conditions of Lemma 3.5. Thus, there exists an invertible measure-preserving
![]() $T_n$
defined on a subset of
$T_n$
defined on a subset of
![]() $Z_n$
such that
$Z_n$
such that
 $$ \begin{align*} Z_n = \bigcup_{i=1}^{\infty} \bigcup_{j=0}^{h_{n,i}-1} T_n^j I_{n,i}. \end{align*} $$
$$ \begin{align*} Z_n = \bigcup_{i=1}^{\infty} \bigcup_{j=0}^{h_{n,i}-1} T_n^j I_{n,i}. \end{align*} $$
Using Lemma 3.5, we can require
 $$ \begin{align*} \bigg| \sum_{j=0}^{h_{n,i}-1} f( T_n^j x ) \bigg| < \epsilon, \end{align*} $$
$$ \begin{align*} \bigg| \sum_{j=0}^{h_{n,i}-1} f( T_n^j x ) \bigg| < \epsilon, \end{align*} $$
and for
![]() $0 \leq k < h_{n,i}$
,
$0 \leq k < h_{n,i}$
,
 $$ \begin{align*} \bigg| \sum_{j=0}^{k} f( T_n^j x ) \bigg| < \| f_{|Z_n} \|_{\infty} + \epsilon. \end{align*} $$
$$ \begin{align*} \bigg| \sum_{j=0}^{k} f( T_n^j x ) \bigg| < \| f_{|Z_n} \|_{\infty} + \epsilon. \end{align*} $$
Since
![]() $Z_n$
are disjoint, we can use T to represent the ensemble of
$Z_n$
are disjoint, we can use T to represent the ensemble of
![]() $T_n$
. Let
$T_n$
. Let
 $$ \begin{align*} A = \bigcup_{n=1}^{\infty} \bigcup_{i=1}^{\infty} I_{n,i} \end{align*} $$
$$ \begin{align*} A = \bigcup_{n=1}^{\infty} \bigcup_{i=1}^{\infty} I_{n,i} \end{align*} $$
and define the function
![]() $f_A$
by
$f_A$
by
 $$ \begin{align*} f_A(x) = \sum_{j=0}^{h_{n,i}-1} f( T^j x )\quad\textrm{for } x\in I_{n,i}. \end{align*} $$
$$ \begin{align*} f_A(x) = \sum_{j=0}^{h_{n,i}-1} f( T^j x )\quad\textrm{for } x\in I_{n,i}. \end{align*} $$
Thus,
![]() $| f_A(x) | < \epsilon $
for
$| f_A(x) | < \epsilon $
for
![]() $x\in A$
. Apply Proposition 3.6 to construct an ergodic invertible measure-preserving transformation
$x\in A$
. Apply Proposition 3.6 to construct an ergodic invertible measure-preserving transformation
![]() $\tau :A\to A$
along with a bounded measurable function
$\tau :A\to A$
along with a bounded measurable function
![]() $g_A$
such that
$g_A$
such that
![]() $f_A$
is a coboundary for
$f_A$
is a coboundary for
![]() $\tau $
with transfer function
$\tau $
with transfer function
![]() $g_A$
. Moreover, we can require that
$g_A$
. Moreover, we can require that
![]() $|g_A(x)| < \| f_A \|_{\infty } + \epsilon < 2\epsilon $
for
$|g_A(x)| < \| f_A \|_{\infty } + \epsilon < 2\epsilon $
for
![]() $x\in A$
. Then apply Lemma 3.2 to show that the ergodic measure-preserving transformation T on X satisfying
$x\in A$
. Then apply Lemma 3.2 to show that the ergodic measure-preserving transformation T on X satisfying
![]() $T_A = \tau $
has coboundary f with a measurable transfer function g. By Lemma 3.2, g may be bounded such that for
$T_A = \tau $
has coboundary f with a measurable transfer function g. By Lemma 3.2, g may be bounded such that for
![]() $z\in Z_n$
,
$z\in Z_n$
,
 $$ \begin{align} | g(z) | &\leq | g_A(T^{-j_z}z) | + \bigg| \sum_{i=0}^{j_z-1} f(T^{i-j_z}z) \bigg|\end{align} $$
$$ \begin{align} | g(z) | &\leq | g_A(T^{-j_z}z) | + \bigg| \sum_{i=0}^{j_z-1} f(T^{i-j_z}z) \bigg|\end{align} $$
There exists j such that
![]() $k_j \geq \ell _j$
, and for
$k_j \geq \ell _j$
, and for
![]() $n> j$
,
$n> j$
,
![]() $k_n + 3\epsilon \leq 2 ( k_n - 1 )$
, and also for
$k_n + 3\epsilon \leq 2 ( k_n - 1 )$
, and also for
![]() $x\in Y_{n}^{\prime }$
,
$x\in Y_{n}^{\prime }$
,
Thus,
 $$ \begin{align} \int_{X} | g |^{p - 1}\,d\mu &= \sum_{n=1}^{\infty} \int_{Z_n} | g |^{p-1}\,d\mu \end{align} $$
$$ \begin{align} \int_{X} | g |^{p - 1}\,d\mu &= \sum_{n=1}^{\infty} \int_{Z_n} | g |^{p-1}\,d\mu \end{align} $$
 $$ \begin{align} &= \sum_{n=1}^{j} \int_{Z_n} | g |^{p-1}\,d\mu + \sum_{n=j+1}^{\infty} \int_{Z_n} | g |^{p-1}\,d\mu. \end{align} $$
$$ \begin{align} &= \sum_{n=1}^{j} \int_{Z_n} | g |^{p-1}\,d\mu + \sum_{n=j+1}^{\infty} \int_{Z_n} | g |^{p-1}\,d\mu. \end{align} $$
Since
![]() $|g|^{p-1}$
is bounded on
$|g|^{p-1}$
is bounded on
![]() $Z_n$
for
$Z_n$
for
![]() $n \leq j$
, then
$n \leq j$
, then
![]() $\sum _{n=1}^{j} \int _{Z_n} | g |^{p-1}\,d\mu < \infty $
. For each
$\sum _{n=1}^{j} \int _{Z_n} | g |^{p-1}\,d\mu < \infty $
. For each
![]() , let
, let
![]() $m_n = \max {\!\{k_n, \gamma _n\} }$
. If
$m_n = \max {\!\{k_n, \gamma _n\} }$
. If
![]() $m_n = k_n$
, let
$m_n = k_n$
, let
![]() $V_n = X_n^{\prime }$
and
$V_n = X_n^{\prime }$
and
![]() $W_n = Y_n^{\prime }$
. Otherwise, let
$W_n = Y_n^{\prime }$
. Otherwise, let
![]() $V_n = Y_n^{\prime }$
and
$V_n = Y_n^{\prime }$
and
![]() $W_n = X_n^{\prime }$
. Thus, for
$W_n = X_n^{\prime }$
. Thus, for
![]() $n> j$
,
$n> j$
,
 $$ \begin{align} \int_{V_n} | f |\,d\mu &\leq \int_{V_n} (m_n)\,d\mu\end{align} $$
$$ \begin{align} \int_{V_n} | f |\,d\mu &\leq \int_{V_n} (m_n)\,d\mu\end{align} $$
 $$ \begin{align} &= \frac{(m_n)\mu(V_n)}{(\gamma_{j})\mu(W_n)} ( \gamma_j ) \mu ( W_n )\end{align} $$
$$ \begin{align} &= \frac{(m_n)\mu(V_n)}{(\gamma_{j})\mu(W_n)} ( \gamma_j ) \mu ( W_n )\end{align} $$
 $$ \begin{align} &\leq \frac{(m_n)\mu(V_n)}{(\gamma_{j})\mu(W_n)} \int_{W_n} | f |\,d\mu.\end{align} $$
$$ \begin{align} &\leq \frac{(m_n)\mu(V_n)}{(\gamma_{j})\mu(W_n)} \int_{W_n} | f |\,d\mu.\end{align} $$
This implies
 $$ \begin{align*} \frac{ \mu( W_n ) }{ m_{n} } \leq \frac{ \mu(V_n) }{ \gamma_{j} }. \end{align*} $$
$$ \begin{align*} \frac{ \mu( W_n ) }{ m_{n} } \leq \frac{ \mu(V_n) }{ \gamma_{j} }. \end{align*} $$
Thus,
 $$ \begin{align*} \sum_{n=j+1}^{\infty} \int_{Z_n} |g|^{p-1}\,d\mu &= \sum_{n=j+1}^{\infty} \int_{V_n} |g|^{p-1}\,d\mu + \sum_{n=j+1}^{\infty} \int_{W_n} |g|^{p-1}\,d\mu \\ &\leq \sum_{n=j+1}^{\infty} \int_{V_n} (m_n + 3\epsilon)^{p-1}\,d\mu + \sum_{n=j+1}^{\infty} \int_{W_n} (m_n + 3\epsilon)^{p-1}\,d\mu \\ &\leq \sum_{n=j+1}^{\infty} \int_{V_n} (m_n + 3\epsilon)^{p-1}\,d\mu + \sum_{n=j+1}^{\infty} \int_{V_n} (m_n + 3\epsilon)^{p} \frac{1}{\gamma_j}\,d\mu \\ &= \sum_{n=j+1}^{\infty} \int_{V_n} (m_n - 1)^{p-1} \frac{(m_n + 3\epsilon)^{p-1}}{(m_n - 1)^{p-1}}\,d\mu\\ &\quad+ \frac{1}{\gamma_j} \sum_{n=j+1}^{\infty} \int_{V_n} (m_n - 1)^{p} \frac{(m_n + 3\epsilon)^{p}}{(m_n - 1)^{p}}\,d\mu \\ &\leq 2^{p-1} \sum_{n=j+1}^{\infty} \int_{V_n} (m_n - 1)^{p-1}\,d\mu + \frac{2^p}{\gamma_j} \sum_{n=j+1}^{\infty} \int_{V_n} (m_n - 1)^{p}\,d\mu \\ &\leq 2^{p-1} \sum_{n=j+1}^{\infty} \int_{V_n} | f |^{p-1}\,d\mu + \frac{2^p}{\gamma_j} \sum_{n=j+1}^{\infty} \int_{V_n} | f |^{p}\,d\mu \\ &\leq 2^{p-1} \| f \|_{p-1}^{p-1} + \frac{2^p}{\gamma_j} \| f \|_p^{p} < \infty. \end{align*} $$
$$ \begin{align*} \sum_{n=j+1}^{\infty} \int_{Z_n} |g|^{p-1}\,d\mu &= \sum_{n=j+1}^{\infty} \int_{V_n} |g|^{p-1}\,d\mu + \sum_{n=j+1}^{\infty} \int_{W_n} |g|^{p-1}\,d\mu \\ &\leq \sum_{n=j+1}^{\infty} \int_{V_n} (m_n + 3\epsilon)^{p-1}\,d\mu + \sum_{n=j+1}^{\infty} \int_{W_n} (m_n + 3\epsilon)^{p-1}\,d\mu \\ &\leq \sum_{n=j+1}^{\infty} \int_{V_n} (m_n + 3\epsilon)^{p-1}\,d\mu + \sum_{n=j+1}^{\infty} \int_{V_n} (m_n + 3\epsilon)^{p} \frac{1}{\gamma_j}\,d\mu \\ &= \sum_{n=j+1}^{\infty} \int_{V_n} (m_n - 1)^{p-1} \frac{(m_n + 3\epsilon)^{p-1}}{(m_n - 1)^{p-1}}\,d\mu\\ &\quad+ \frac{1}{\gamma_j} \sum_{n=j+1}^{\infty} \int_{V_n} (m_n - 1)^{p} \frac{(m_n + 3\epsilon)^{p}}{(m_n - 1)^{p}}\,d\mu \\ &\leq 2^{p-1} \sum_{n=j+1}^{\infty} \int_{V_n} (m_n - 1)^{p-1}\,d\mu + \frac{2^p}{\gamma_j} \sum_{n=j+1}^{\infty} \int_{V_n} (m_n - 1)^{p}\,d\mu \\ &\leq 2^{p-1} \sum_{n=j+1}^{\infty} \int_{V_n} | f |^{p-1}\,d\mu + \frac{2^p}{\gamma_j} \sum_{n=j+1}^{\infty} \int_{V_n} | f |^{p}\,d\mu \\ &\leq 2^{p-1} \| f \|_{p-1}^{p-1} + \frac{2^p}{\gamma_j} \| f \|_p^{p} < \infty. \end{align*} $$
This completes the proof that
![]() $g \in L^{p-1}(X)$
.
$g \in L^{p-1}(X)$
.
4 Non-existence of
 $L^q$
-coboundaries
$L^q$
-coboundaries
In [Reference Kornfeld30], Kornfeld shows that given
![]() $T \in \mathcal {E}$
, which is a homeomorphism on a compact space X, there exists a continuous and bounded coboundary f such that its associated transfer function is measurable, but not integrable. Also, it is pointed out that given T, f may be constructed such that the transfer function g is in
$T \in \mathcal {E}$
, which is a homeomorphism on a compact space X, there exists a continuous and bounded coboundary f such that its associated transfer function is measurable, but not integrable. Also, it is pointed out that given T, f may be constructed such that the transfer function g is in
![]() $L^p$
for specified
$L^p$
for specified
![]() $p \geq 1$
, but not contained in
$p \geq 1$
, but not contained in
![]() $L^q$
for
$L^q$
for
![]() $q> p$
. However, if the function
$q> p$
. However, if the function
![]() $f\in L^p_0$
is specified first, Kornfeld conjectured that there always exist an ergodic invertible measure-preserving transformation T and
$f\in L^p_0$
is specified first, Kornfeld conjectured that there always exist an ergodic invertible measure-preserving transformation T and
![]() $g \in L^p$
such that
$g \in L^p$
such that
![]() $f = g - g \circ T$
almost everywhere. (Kornfeld conveyed this conjecture to the second author verbally or through email.) In this section, we disprove this conjecture. Furthermore, we prove a strong non-existence result showing that for generic
$f = g - g \circ T$
almost everywhere. (Kornfeld conveyed this conjecture to the second author verbally or through email.) In this section, we disprove this conjecture. Furthermore, we prove a strong non-existence result showing that for generic
![]() $f \in L^p_0$
, there are no
$f \in L^p_0$
, there are no
![]() $T \in \mathcal {E}$
and
$T \in \mathcal {E}$
and
![]() $g \in L^q$
for
$g \in L^q$
for
![]() $q> p - 1$
such that
$q> p - 1$
such that
![]() $f = g - g \circ T$
almost everywhere. This is the statement of Theorem 1.2, and shows that generic
$f = g - g \circ T$
almost everywhere. This is the statement of Theorem 1.2, and shows that generic
![]() $L^p_0$
functions lead to ‘wild’ transfer functions (as termed in [Reference Kornfeld30]), universally for all
$L^p_0$
functions lead to ‘wild’ transfer functions (as termed in [Reference Kornfeld30]), universally for all
![]() $T \in \mathcal {E}$
. Remark 2 in [Reference Kwapień32] provides an argument for the existence of
$T \in \mathcal {E}$
. Remark 2 in [Reference Kwapień32] provides an argument for the existence of
![]() $L^{p}_0$
functions f for
$L^{p}_0$
functions f for
![]() $p \geq 2$
which are not coboundaries for any ergodic measure-preserving transformation T with transfer function
$p \geq 2$
which are not coboundaries for any ergodic measure-preserving transformation T with transfer function
![]() $g \in L^{p}$
. The argument in [Reference Kwapień32] can be extended to show there are functions
$g \in L^{p}$
. The argument in [Reference Kwapień32] can be extended to show there are functions
![]() $f \in L^{p}_0$
which are not coboundaries for any ergodic measure-preserving transformation T with transfer function
$f \in L^{p}_0$
which are not coboundaries for any ergodic measure-preserving transformation T with transfer function
![]() $g \in L^{q}$
for
$g \in L^{q}$
for
![]() $q> p - 1$
. This is proved in the following section. Then, in §4.2, we show this situation is generic for
$q> p - 1$
. This is proved in the following section. Then, in §4.2, we show this situation is generic for
![]() $f \in L^{p}_0$
.
$f \in L^{p}_0$
.
4.1 Extension of the Kwapień argument for the non-existence of
 $L^{q}$
-coboundaries
$L^{q}$
-coboundaries
The following proposition establishes the existence of
![]() $L^p$
functions f with no transfer function in
$L^p$
functions f with no transfer function in
![]() $L^q$
for
$L^q$
for
![]() $q> p - 1$
. The argument is due to Kwapień [Reference Kwapień32].
$q> p - 1$
. The argument is due to Kwapień [Reference Kwapień32].
Proposition 4.1. (Remark 2 in [Reference Kwapień32])
Given
![]() such that
such that
![]() $p \geq 2$
, there exists
$p \geq 2$
, there exists
![]() $f \in L^{p}$
such that for any solution pair T and g to the equation
$f \in L^{p}$
such that for any solution pair T and g to the equation
![]() $f = g - g\circ T$
, where T is an ergodic invertible measure-preserving transformation, then
$f = g - g\circ T$
, where T is an ergodic invertible measure-preserving transformation, then
![]() $g \notin L^q$
for
$g \notin L^q$
for
![]() $q> p - 1$
.
$q> p - 1$
.
Proof. Let
![]() $f \in L^p$
be such that
$f \in L^p$
be such that
![]() $\int f\,d\mu = 0$
,
$\int f\,d\mu = 0$
,
![]() $f(x) \geq -1$
for a.e. x, and for
$f(x) \geq -1$
for a.e. x, and for
![]() $r> p - 1$
,
$r> p - 1$
,
 $$ \begin{align*} \limsup_{n \to \infty} \bigg( n \int_{f> n} | f - n |^{r} \bigg)\,d\mu = \infty. \end{align*} $$
$$ \begin{align*} \limsup_{n \to \infty} \bigg( n \int_{f> n} | f - n |^{r} \bigg)\,d\mu = \infty. \end{align*} $$
We refer to this as the Kwapień condition. To obtain examples f that satisfy the Kwapień condition, suppose
![]() $p \geq 2$
,
$p \geq 2$
,
![]() $r> p - 1$
, and let
$r> p - 1$
, and let
![]() be such that
be such that
 $$ \begin{align} \sum_{k=1}^{\infty} N_k^{ - {(1+r-p)}/{2(r+1)} } < \frac{1}{2^{p+1}}. \end{align} $$
$$ \begin{align} \sum_{k=1}^{\infty} N_k^{ - {(1+r-p)}/{2(r+1)} } < \frac{1}{2^{p+1}}. \end{align} $$
Let
By (4.1),
Let
![]() $E_k$
be disjoint sets for
$E_k$
be disjoint sets for
![]() such that
such that
![]() $\mu (E_k) = {1}/{N_k^p}$
. Thus,
$\mu (E_k) = {1}/{N_k^p}$
. Thus,
 $$ \begin{align*} \sum_{k=1}^{\infty} N_k^{ - {p(1+r-p)}/{2(r+1)} } < {1}/{2^{p+1}}. \end{align*} $$
$$ \begin{align*} \sum_{k=1}^{\infty} N_k^{ - {p(1+r-p)}/{2(r+1)} } < {1}/{2^{p+1}}. \end{align*} $$
Define
![]() $f^{+}$
such that
$f^{+}$
such that
 $$ \begin{align*} f^{+} = \sum_{k=1}^{\infty} 2 N_k^{ 1 - \delta } I_{E_k}. \end{align*} $$
$$ \begin{align*} f^{+} = \sum_{k=1}^{\infty} 2 N_k^{ 1 - \delta } I_{E_k}. \end{align*} $$
We have shown that
![]() $\int f^{+}\,d\mu < {1} / {2}$
. Let
$\int f^{+}\,d\mu < {1} / {2}$
. Let
![]() $E_0$
be a subset disjoint from
$E_0$
be a subset disjoint from
![]() $\bigcup _{k=1}^{\infty } E_k$
such that
$\bigcup _{k=1}^{\infty } E_k$
such that
![]() $\mu (E_0) = \int f^{+}\,d\mu $
. Define
$\mu (E_0) = \int f^{+}\,d\mu $
. Define
![]() $f = f^{+} - I_{E_0}$
. Thus,
$f = f^{+} - I_{E_0}$
. Thus,
![]() $\int f\,d\mu = 0$
and
$\int f\,d\mu = 0$
and
![]() $\| f \|_p < \infty $
.
$\| f \|_p < \infty $
.
Let
![]() $L_k = n_k\int _{f> n_k} (f - n_k)^r\,d\mu $
, where
$L_k = n_k\int _{f> n_k} (f - n_k)^r\,d\mu $
, where
![]() $n_k = N_{k}^{1-\delta }$
. This
$n_k = N_{k}^{1-\delta }$
. This
![]() $n_k$
is not a whole number probably, but we are going to ignore that. Then
$n_k$
is not a whole number probably, but we are going to ignore that. Then
![]() $L_k \ge N_{k}^{1-\delta } \int _{E_{k}} ( N_{k_o}^{1-\delta })^r\,d\mu $
. We get
$L_k \ge N_{k}^{1-\delta } \int _{E_{k}} ( N_{k_o}^{1-\delta })^r\,d\mu $
. We get
Since
![]() $\lim _{k\to \infty } L_k = \infty $
and f satisfies the Kwapień condition.
$\lim _{k\to \infty } L_k = \infty $
and f satisfies the Kwapień condition.
Now we prove that f is not a coboundary with a transfer function in
![]() $L^r$
for any
$L^r$
for any
![]() $r> p - 1$
. Since
$r> p - 1$
. Since
![]() $f \geq -1$
almost everywhere, then for a.e. x,
$f \geq -1$
almost everywhere, then for a.e. x,
 $$ \begin{align*} \bigg| \sum_{i=0}^{n} f ( T^i x ) \bigg| \geq \sum_{i=0}^{n} ( f ( T^i x ) - n ) \mathbb{I} \{ f (T^i x)> n \}. \end{align*} $$
$$ \begin{align*} \bigg| \sum_{i=0}^{n} f ( T^i x ) \bigg| \geq \sum_{i=0}^{n} ( f ( T^i x ) - n ) \mathbb{I} \{ f (T^i x)> n \}. \end{align*} $$
Each term in the sum on the right-hand side of the inequality is non-negative and therefore,
 $$ \begin{align} \bigg\| \sum_{i=0}^{n} f ( T^i x ) \bigg\|_r^r & \geq \sum_{i=0}^{n} \int_{f\circ T^i> n} ( f ( T^i x ) - n )^r\,d\mu\end{align} $$
$$ \begin{align} \bigg\| \sum_{i=0}^{n} f ( T^i x ) \bigg\|_r^r & \geq \sum_{i=0}^{n} \int_{f\circ T^i> n} ( f ( T^i x ) - n )^r\,d\mu\end{align} $$
 $$ \begin{align} & = (n + 1) \int_{f> n} ( f - n )^r\,d\mu. \end{align} $$
$$ \begin{align} & = (n + 1) \int_{f> n} ( f - n )^r\,d\mu. \end{align} $$
Therefore,
![]() $\sum _{i=0}^{n} f ( T^i x ) = g(x) - g ( T^{n+1} x )$
is unbounded in
$\sum _{i=0}^{n} f ( T^i x ) = g(x) - g ( T^{n+1} x )$
is unbounded in
![]() $L^r$
.
$L^r$
.
4.2 Genericity of the strong non-existence result
A principal obstacle to solving the coboundary equation is imbalance between the positive and negative parts of a typical function
![]() $f \in L^p_0$
. Suppose
$f \in L^p_0$
. Suppose
![]() for
for
![]() is an increasing sequence of real numbers such that
is an increasing sequence of real numbers such that
![]() $\lim _{i\to \infty } a_i = \infty $
, and for all real
$\lim _{i\to \infty } a_i = \infty $
, and for all real
![]() $\alpha> 0$
,
$\alpha> 0$
,
An example of
![]() $a_i$
satisfying (4.4) is
$a_i$
satisfying (4.4) is
Given
![]() $f\in L^p$
and
$f\in L^p$
and
![]() , let
, let
We are ready to define our generic class of
![]() $L^p_0$
functions. Given
$L^p_0$
functions. Given
![]() , define
, define
 $$ \begin{align*} \mathcal{G}^p_n = \bigg\{ f\in L^p_0 : \text{there exists } i> n\ \mid \ \mu(v_i(f)) > \frac{1}{a_i^p i^2} \textrm{ and } \mu(u_{i-1} (f)) < \frac{1}{a_{i+1}^{p} i^2} \bigg\}. \end{align*} $$
$$ \begin{align*} \mathcal{G}^p_n = \bigg\{ f\in L^p_0 : \text{there exists } i> n\ \mid \ \mu(v_i(f)) > \frac{1}{a_i^p i^2} \textrm{ and } \mu(u_{i-1} (f)) < \frac{1}{a_{i+1}^{p} i^2} \bigg\}. \end{align*} $$
Below we prove that
![]() $\mathcal {G}^p_n$
is both open and dense, and
$\mathcal {G}^p_n$
is both open and dense, and
![]() $f \in \bigcap _{n=1}^{\infty } \mathcal {G}^p_n$
satisfies the required property. The key property of the sequence
$f \in \bigcap _{n=1}^{\infty } \mathcal {G}^p_n$
satisfies the required property. The key property of the sequence
![]() $a_n$
is the fast growth rate. The following lemma will be used to guarantee that coboundaries
$a_n$
is the fast growth rate. The following lemma will be used to guarantee that coboundaries
![]() $f\in \bigcap _{n=1}^{\infty } \mathcal {G}^p_n$
do not have transfer functions in
$f\in \bigcap _{n=1}^{\infty } \mathcal {G}^p_n$
do not have transfer functions in
![]() $L^q$
for
$L^q$
for
![]() $q> p - 1$
.
$q> p - 1$
.
Lemma 4.2. For any
![]() $\alpha> 0$
,
$\alpha> 0$
,
 $$ \begin{align*} \lim_{n\to \infty} \frac{a_{n+1}^{\alpha}}{a_n n^2} = \infty. \end{align*} $$
$$ \begin{align*} \lim_{n\to \infty} \frac{a_{n+1}^{\alpha}}{a_n n^2} = \infty. \end{align*} $$
Proof. Let
![]() $\alpha> 0$
. Define
$\alpha> 0$
. Define
![]() $\beta = \min {\{ {\alpha }/{2}, {1}/{2} \}}$
. By condition (4.4), for sufficiently large n,
$\beta = \min {\{ {\alpha }/{2}, {1}/{2} \}}$
. By condition (4.4), for sufficiently large n,
![]() $a_{n+1}^{\beta }> 2 a_n > 2 a_n^{\beta }$
. Thus,
$a_{n+1}^{\beta }> 2 a_n > 2 a_n^{\beta }$
. Thus,
![]() $a_{n+k}^{\beta }> 2^k$
for sufficiently large n and
$a_{n+k}^{\beta }> 2^k$
for sufficiently large n and
![]() . Hence,
. Hence,
 $$ \begin{align*} \lim_{n\to \infty} \frac{a_{n+1}^{{\alpha}/{2}}}{n^2} = \infty. \end{align*} $$
$$ \begin{align*} \lim_{n\to \infty} \frac{a_{n+1}^{{\alpha}/{2}}}{n^2} = \infty. \end{align*} $$
Therefore,
 $$ \begin{align*} \lim_{n\to \infty} \frac{a_{n+1}^{\alpha}}{n^2 a_n} = \lim_{n\to \infty} \bigg( \frac{a_{n+1}^{{\alpha}/{2}}}{n^2} \bigg) \bigg( \frac{a_{n+1}^{{\alpha}/{2}}}{a_n} \bigg) = \infty.\\[-34pt] \end{align*} $$
$$ \begin{align*} \lim_{n\to \infty} \frac{a_{n+1}^{\alpha}}{n^2 a_n} = \lim_{n\to \infty} \bigg( \frac{a_{n+1}^{{\alpha}/{2}}}{n^2} \bigg) \bigg( \frac{a_{n+1}^{{\alpha}/{2}}}{a_n} \bigg) = \infty.\\[-34pt] \end{align*} $$
Now we prove that
![]() $\mathcal {G}^p_n$
is dense in
$\mathcal {G}^p_n$
is dense in
![]() $L_p$
for each
$L_p$
for each
![]() $p\geq 1$
and
$p\geq 1$
and
![]() .
.
Lemma 4.3. For each
![]() , the set
, the set
![]() $\mathcal {G}^p_n$
is dense in
$\mathcal {G}^p_n$
is dense in
![]() $L^p_0$
.
$L^p_0$
.
Proof. Let
![]() $f \in L^p_0$
,
$f \in L^p_0$
,
![]() $\epsilon> 0$
, and
$\epsilon> 0$
, and
![]() . Since bounded measurable functions are dense in
. Since bounded measurable functions are dense in
![]() $L^p_0$
, we can choose a bounded mean zero
$L^p_0$
, we can choose a bounded mean zero
![]() $f_0 \in L^p$
such that
$f_0 \in L^p$
such that
Choose
![]() $i_1 \geq n$
such that
$i_1 \geq n$
such that
![]() $a_{i_1}> \| f_0 \|_{\infty }$
and
$a_{i_1}> \| f_0 \|_{\infty }$
and
 $$ \begin{align*} 4 \cdot \frac{2^{1/p}}{i_1^{2/p}} < \frac{\epsilon}{3}. \end{align*} $$
$$ \begin{align*} 4 \cdot \frac{2^{1/p}}{i_1^{2/p}} < \frac{\epsilon}{3}. \end{align*} $$
Choose a subset
![]() $Y \subset X$
such that
$Y \subset X$
such that
 $$ \begin{align*} \mu(Y) = \frac{2}{a_{i_1}^{p} i_1^2 } + \frac{4}{a_{i_1-1}a_{i_1}^{p-1} i_1^2 } \end{align*} $$
$$ \begin{align*} \mu(Y) = \frac{2}{a_{i_1}^{p} i_1^2 } + \frac{4}{a_{i_1-1}a_{i_1}^{p-1} i_1^2 } \end{align*} $$
and
![]() $\int _Y f_0\,d\mu = 0$
. To see that Y exists, note that our measure space
$\int _Y f_0\,d\mu = 0$
. To see that Y exists, note that our measure space
![]() $(X,\mu )$
is isomorphic to the unit circle
$(X,\mu )$
is isomorphic to the unit circle
![]() $S^1$
with normalized Lebesgue measure. Let
$S^1$
with normalized Lebesgue measure. Let
![]() $\mu _0 = {2}/{a_{i_1}^{p} i_1^2 } + {4}/{a_{i_1-1}a_{i_1}^{p-1} i_1^2 }$
. By Fubini’s theorem,
$\mu _0 = {2}/{a_{i_1}^{p} i_1^2 } + {4}/{a_{i_1-1}a_{i_1}^{p-1} i_1^2 }$
. By Fubini’s theorem,
 $$ \begin{align*} \int_{0}^{1} \bigg( \int_{0}^{\mu_0} f_0 (e^{2\pi i (t+x)})\, dt \bigg)\, dx = \int_{0}^{\mu_0} \bigg( \int_{0}^{1} f_0 (e^{2\pi i (t+x)})\, dx \bigg)\, dt = 0. \end{align*} $$
$$ \begin{align*} \int_{0}^{1} \bigg( \int_{0}^{\mu_0} f_0 (e^{2\pi i (t+x)})\, dt \bigg)\, dx = \int_{0}^{\mu_0} \bigg( \int_{0}^{1} f_0 (e^{2\pi i (t+x)})\, dx \bigg)\, dt = 0. \end{align*} $$
Thus, by a change of variable and the intermediate value theorem applied to the continuous function
![]() $x \mapsto \int _{x}^{x+\mu _0} f_0 (e^{2\pi i t}) \,dt$
, there exists
$x \mapsto \int _{x}^{x+\mu _0} f_0 (e^{2\pi i t}) \,dt$
, there exists
![]() $x_0 \in [0,1]$
such that
$x_0 \in [0,1]$
such that
 $$ \begin{align*} \int_{x_0}^{x_0+\mu_0} f_0 (e^{2\pi i t}) \,dt = \int_{0}^{\mu_0} f_0 (e^{2\pi i (t+x_0)}) \,dt = 0. \end{align*} $$
$$ \begin{align*} \int_{x_0}^{x_0+\mu_0} f_0 (e^{2\pi i t}) \,dt = \int_{0}^{\mu_0} f_0 (e^{2\pi i (t+x_0)}) \,dt = 0. \end{align*} $$
Since a set Y with measure
![]() $\mu _0$
exists in this case, by using a measure-space isomorphism, it exists for a general Lebesgue space.
$\mu _0$
exists in this case, by using a measure-space isomorphism, it exists for a general Lebesgue space.
Let
![]() $V\subset Y$
be such that
$V\subset Y$
be such that
![]() $\mu (V) = 2 ( a_{i_1}^p i_1^2 )^{-1}$
and define
$\mu (V) = 2 ( a_{i_1}^p i_1^2 )^{-1}$
and define
![]() $U = Y\setminus V$
. Define
$U = Y\setminus V$
. Define
![]() $f_1$
as a modification of
$f_1$
as a modification of
![]() $f_0$
in the following manner:
$f_0$
in the following manner:
 $$ \begin{align*} f_1(x)= \begin{cases} 2 a_{i_1} & \textrm{if}\ x\in V, \\ - a_{i_1 - 1} & \textrm{if}\ x\in U, \\ f_0(x) & \textrm{if}\ x\in X\setminus Y. \end{cases}\end{align*} $$
$$ \begin{align*} f_1(x)= \begin{cases} 2 a_{i_1} & \textrm{if}\ x\in V, \\ - a_{i_1 - 1} & \textrm{if}\ x\in U, \\ f_0(x) & \textrm{if}\ x\in X\setminus Y. \end{cases}\end{align*} $$
Thus,
 $$ \begin{align*} \| f - f_1 \|_{p} &\leq \| f - f_0 \|_{p} + \| f_0 - f_1 \|_{p} \\ & < \frac{\epsilon}{3} + 3 a_{i_1} \mu(V)^{1/p} + 2 a_{i_1-1} \mu(U)^{1/p} \\ & \leq \frac{\epsilon}{3} + 3 \cdot \frac{2^{1/p}}{i_1^{2/p}} + 2 \cdot \frac{4^{1/p}}{i_1^{2/p}} \\ & < \epsilon. \end{align*} $$
$$ \begin{align*} \| f - f_1 \|_{p} &\leq \| f - f_0 \|_{p} + \| f_0 - f_1 \|_{p} \\ & < \frac{\epsilon}{3} + 3 a_{i_1} \mu(V)^{1/p} + 2 a_{i_1-1} \mu(U)^{1/p} \\ & \leq \frac{\epsilon}{3} + 3 \cdot \frac{2^{1/p}}{i_1^{2/p}} + 2 \cdot \frac{4^{1/p}}{i_1^{2/p}} \\ & < \epsilon. \end{align*} $$
Also,
![]() $f_1 \in \mathcal {G}^p_n$
, which completes the proof.
$f_1 \in \mathcal {G}^p_n$
, which completes the proof.
Lemma 4.4. For each
![]() , the set
, the set
![]() $\mathcal {G}^p_n$
is open in
$\mathcal {G}^p_n$
is open in
![]() $L^p_0$
.
$L^p_0$
.
Proof. Suppose
![]() $f \in \mathcal {G}^p_n$
. Then there exists
$f \in \mathcal {G}^p_n$
. Then there exists
![]() $i \geq n$
such that
$i \geq n$
such that
 $$ \begin{align*} \mu_i &= \mu(v_i(f))> \frac{1}{a_i^p i^2} ,\\ \nu_i &= \mu(u_{i-1}(f)) < \frac{1}{a_{i+1}^{p} i^2}. \end{align*} $$
$$ \begin{align*} \mu_i &= \mu(v_i(f))> \frac{1}{a_i^p i^2} ,\\ \nu_i &= \mu(u_{i-1}(f)) < \frac{1}{a_{i+1}^{p} i^2}. \end{align*} $$
Thus, by right continuity of
![]() ${f> t}$
, there exist
${f> t}$
, there exist
![]() $a^{\prime }> a_i$
and
$a^{\prime }> a_i$
and
![]() $a^{\prime \prime }> a_{i-1}$
, and
$a^{\prime \prime }> a_{i-1}$
, and
![]() $\mu ^{\prime }, \nu ^{\prime }$
such that
$\mu ^{\prime }, \nu ^{\prime }$
such that
 $$ \begin{align*} \mu( \{ x: f(x)> a^{\prime} \} ) &> \mu^{\prime} > \frac{1}{a_i^p i^2 } , \\ \mu( \{ x: f(x) < - a^{\prime \prime} \} ) &< \nu^{\prime} < \frac{1}{a_{i+1}^{p} i^2 }. \end{align*} $$
$$ \begin{align*} \mu( \{ x: f(x)> a^{\prime} \} ) &> \mu^{\prime} > \frac{1}{a_i^p i^2 } , \\ \mu( \{ x: f(x) < - a^{\prime \prime} \} ) &< \nu^{\prime} < \frac{1}{a_{i+1}^{p} i^2 }. \end{align*} $$
Define
![]() $\epsilon> 0$
as
$\epsilon> 0$
as
 $$ \begin{align*} \epsilon = \min{ \bigg\{ \bigg( \mu^{\prime} - \frac{1}{a_i^p i^2} \bigg)^{1/p} ( a^{\prime} - a_i ) , \bigg( \frac{1}{a_{i+1}^{p} i^2 } - \nu^{\prime} \bigg)^{1/p} (a^{\prime \prime} - a_{i-1}) \bigg\} }. \end{align*} $$
$$ \begin{align*} \epsilon = \min{ \bigg\{ \bigg( \mu^{\prime} - \frac{1}{a_i^p i^2} \bigg)^{1/p} ( a^{\prime} - a_i ) , \bigg( \frac{1}{a_{i+1}^{p} i^2 } - \nu^{\prime} \bigg)^{1/p} (a^{\prime \prime} - a_{i-1}) \bigg\} }. \end{align*} $$
It is not difficult to see that the
![]() $\epsilon $
-ball centered at
$\epsilon $
-ball centered at
![]() $f\in L^p_0$
is contained in
$f\in L^p_0$
is contained in
![]() $\mathcal {G}^p_n$
.
$\mathcal {G}^p_n$
.
Let
 $$ \begin{align*} \mathcal{G}_p = \bigcap_{n=1}^{\infty} \mathcal{G}^p_n. \end{align*} $$
$$ \begin{align*} \mathcal{G}_p = \bigcap_{n=1}^{\infty} \mathcal{G}^p_n. \end{align*} $$
Note, for
![]() $f \in \mathcal {G}_n^p$
, also
$f \in \mathcal {G}_n^p$
, also
![]() $f\circ T \in \mathcal {G}_n^p$
for any T measure preserving. The same principle applies to
$f\circ T \in \mathcal {G}_n^p$
for any T measure preserving. The same principle applies to
![]() $\mathcal {G}_p$
;
$\mathcal {G}_p$
;
![]() $f \in \mathcal {G}_p$
implies
$f \in \mathcal {G}_p$
implies
![]() $f\circ T \in \mathcal {G}_p$
.
$f\circ T \in \mathcal {G}_p$
.
We have the following core result of this paper.
Proposition 4.5. Suppose
![]() $f \in \mathcal {G}_p$
,
$f \in \mathcal {G}_p$
,
![]() $T\in \mathcal {E}$
, and g is a measurable function. If the coboundary equation
$T\in \mathcal {E}$
, and g is a measurable function. If the coboundary equation
![]() $f = g - g\circ T$
is satisfied almost everywhere, then
$f = g - g\circ T$
is satisfied almost everywhere, then
![]() $g \notin L^q$
for
$g \notin L^q$
for
![]() $q> p - 1$
.
$q> p - 1$
.
Prior to proving Proposition 4.5, we prove the following basic lemma used in the proposition.
Lemma 4.6. Let T be an ergodic invertible measure-preserving transformation on a standard probability space
![]() $(X, {\mathcal B}, \mu )$
. Suppose
$(X, {\mathcal B}, \mu )$
. Suppose
![]() $B \subset X$
is a set of positive measure and
$B \subset X$
is a set of positive measure and
![]() . Let
. Let
![]() be a measurable map such that
be a measurable map such that
![]() $\ell (x) \leq K$
for
$\ell (x) \leq K$
for
![]() $x \in B$
. Define
$x \in B$
. Define
There exists a measurable set J such that for
![]() $x\neq y$
,
$x\neq y$
,
![]() $x,y \in J$
,
$x,y \in J$
,
and
 $$ \begin{align*} \mu \bigg( B \cap \bigcup_{x\in J} B(x) \bigg)> \frac{1}{2} \mu(B). \end{align*} $$
$$ \begin{align*} \mu \bigg( B \cap \bigcup_{x\in J} B(x) \bigg)> \frac{1}{2} \mu(B). \end{align*} $$
Proof. Choose
![]() such that
such that
Let
![]() $\overline {B}$
be a set of positive measure, and
$\overline {B}$
be a set of positive measure, and
![]() $\overline {B}, T\overline {B}, T^2 \overline {B}, \ldots , T^{H-1}\overline {B}$
a Rokhlin tower of height H such that
$\overline {B}, T\overline {B}, T^2 \overline {B}, \ldots , T^{H-1}\overline {B}$
a Rokhlin tower of height H such that
 $$ \begin{align*} \mu \bigg( \bigcup_{i=0}^{H-1} T^i \overline{B} \bigg)> 1 - \frac{K}{H}. \end{align*} $$
$$ \begin{align*} \mu \bigg( \bigcup_{i=0}^{H-1} T^i \overline{B} \bigg)> 1 - \frac{K}{H}. \end{align*} $$
Partition
![]() $\overline {B}$
into finitely many sets of positive measure such that
$\overline {B}$
into finitely many sets of positive measure such that
![]() $\overline {B} = \bigcup _{j=0}^{H^{\prime }} \overline {B}_j$
and for each i,
$\overline {B} = \bigcup _{j=0}^{H^{\prime }} \overline {B}_j$
and for each i,
![]() $0\leq i < H$
, either
$0\leq i < H$
, either
![]() $T^i \overline {B}_j \subset B$
or
$T^i \overline {B}_j \subset B$
or
![]() $T^i \overline {B}_j \subset B^c$
. These are B-pure subcolumns (
$T^i \overline {B}_j \subset B^c$
. These are B-pure subcolumns (
![]() $T^i \overline {B}_j$
,
$T^i \overline {B}_j$
,
![]() $0 \leq i < H-1$
). For each j, let
$0 \leq i < H-1$
). For each j, let
![]() $i_{j}$
be the minimum i such that
$i_{j}$
be the minimum i such that
![]() $T^i \overline {B}_j \subset B$
. If there is no minimum, we can discard that subcolumn. Partition
$T^i \overline {B}_j \subset B$
. If there is no minimum, we can discard that subcolumn. Partition
![]() $T^{i_j} \overline {B}_j$
into finitely many sets of positive measure with equal values of
$T^{i_j} \overline {B}_j$
into finitely many sets of positive measure with equal values of
![]() $\ell (x)$
. Call these sets
$\ell (x)$
. Call these sets
![]() $\overline {B}_{j,\ell }$
. Insert
$\overline {B}_{j,\ell }$
. Insert
![]() $\overline {B}_{j,\ell }$
in J. Consider
$\overline {B}_{j,\ell }$
in J. Consider
![]() $T^{\ell } \overline {B}_{j,\ell }$
and let
$T^{\ell } \overline {B}_{j,\ell }$
and let
![]() $i_{j,\ell }$
be the minimum i such that
$i_{j,\ell }$
be the minimum i such that
![]() $T^{\ell + i} \overline {B}_{j,\ell } \subset B$
. Then partition
$T^{\ell + i} \overline {B}_{j,\ell } \subset B$
. Then partition
![]() $T^{\ell + i_{j,\ell }} \overline {B}_{j,\ell }$
into subsets with the same value
$T^{\ell + i_{j,\ell }} \overline {B}_{j,\ell }$
into subsets with the same value
![]() $\ell (x)$
. Continue this process until each subcolumn is swept out up to at least
$\ell (x)$
. Continue this process until each subcolumn is swept out up to at least
![]() $H-K$
levels of the Rokhlin tower. Each time, a disjoint set is inserted into J. Since B is covered except for a subset of the top K levels, then
$H-K$
levels of the Rokhlin tower. Each time, a disjoint set is inserted into J. Since B is covered except for a subset of the top K levels, then
 $$ \begin{align*} \mu \bigg( B \cap \bigcup_{x\in J} B(x) \bigg)> \frac{1}{2} \mu(B).\\[-45pt] \end{align*} $$
$$ \begin{align*} \mu \bigg( B \cap \bigcup_{x\in J} B(x) \bigg)> \frac{1}{2} \mu(B).\\[-45pt] \end{align*} $$
Proof of Proposition 4.5
Let
![]() $p\geq 1$
,
$p\geq 1$
,
![]() $f \in \mathcal {G}_p$
and
$f \in \mathcal {G}_p$
and
![]() $q> p - 1$
. Choose integer
$q> p - 1$
. Choose integer
![]() $k> 1$
such that
$k> 1$
such that
![]() $kq> p$
and
$kq> p$
and
![]() such that for
such that for
![]() $n \geq N$
,
$n \geq N$
,
 $$ \begin{align*} \frac{a_{n+1}^{p}}{a_{n}^{k+p} }> 4. \end{align*} $$
$$ \begin{align*} \frac{a_{n+1}^{p}}{a_{n}^{k+p} }> 4. \end{align*} $$
Let
![]() $\operatorname {\mathrm {sgn}}$
be the standard sign function defined as
$\operatorname {\mathrm {sgn}}$
be the standard sign function defined as
![]() $\operatorname {\mathrm {sgn}}(i)=-1$
if
$\operatorname {\mathrm {sgn}}(i)=-1$
if
![]() $i<0$
,
$i<0$
,
![]() $\operatorname {\mathrm {sgn}}(i)=0$
if
$\operatorname {\mathrm {sgn}}(i)=0$
if
![]() $i=0$
, and
$i=0$
, and
![]() $\operatorname {\mathrm {sgn}}(i)=1$
if
$\operatorname {\mathrm {sgn}}(i)=1$
if
![]() $i>0$
. For
$i>0$
. For
![]() $i \in \mathbb {Z}$
, let
$i \in \mathbb {Z}$
, let
![]() $[ i ] = \{ j \in \mathbb {Z} : i \leq j < 0 \}$
if
$[ i ] = \{ j \in \mathbb {Z} : i \leq j < 0 \}$
if
![]() $i < 0$
, and
$i < 0$
, and
![]() $[ i ] = \{ j \in \mathbb {Z} : 0 \leq j < i \}$
if
$[ i ] = \{ j \in \mathbb {Z} : 0 \leq j < i \}$
if
![]() $i \geq 0$
. Note, for
$i \geq 0$
. Note, for
![]() $i \in \mathbb {Z}$
, the coboundary equation expands to the following:
$i \in \mathbb {Z}$
, the coboundary equation expands to the following:
 $$ \begin{align*} g(T^i x) = g(x) - \operatorname{\mathrm{sgn}} (i) \sum_{j \in [i]} f ( T^j x). \end{align*} $$
$$ \begin{align*} g(T^i x) = g(x) - \operatorname{\mathrm{sgn}} (i) \sum_{j \in [i]} f ( T^j x). \end{align*} $$
Define our specialized sign function
![]() $\rho _n: X \to \{ -1, 1 \}$
based on the following:
$\rho _n: X \to \{ -1, 1 \}$
based on the following:
-
(1) if
 $g(x) \leq {a_n}/{2}$
, let
$g(x) \leq {a_n}/{2}$
, let
 $\rho _n(x)=1$
;
$\rho _n(x)=1$
; -
(2) otherwise if
 $g(x)> {a_n}/{2}$
, then let
$g(x)> {a_n}/{2}$
, then let
 $\rho _n(x)=-1$
.
$\rho _n(x)=-1$
.
For
![]() , let
, let
![]() $c_n = {a_{n}} / {a_{n-1}}$
. Let
$c_n = {a_{n}} / {a_{n-1}}$
. Let
![]() $A_n = u_{n-1}(f)$
and
$A_n = u_{n-1}(f)$
and
![]() $B_n = v_{n}(f)$
. For
$B_n = v_{n}(f)$
. For
![]() $x \in B_n$
, let
$x \in B_n$
, let
 $$ \begin{align*} \ell_x = \min{ \bigg\{ \ell : \lceil ( c_n )^{h} \rceil \leq \ell < \lceil ( c_n )^{h+1} \rceil ,\ 1 \leq h < k ,\ | g( T^{ \rho_n(x) \ell } x ) | < \frac{ a_{n} }{4} ( c_n )^{h-1} \bigg\} }, \end{align*} $$
$$ \begin{align*} \ell_x = \min{ \bigg\{ \ell : \lceil ( c_n )^{h} \rceil \leq \ell < \lceil ( c_n )^{h+1} \rceil ,\ 1 \leq h < k ,\ | g( T^{ \rho_n(x) \ell } x ) | < \frac{ a_{n} }{4} ( c_n )^{h-1} \bigg\} }, \end{align*} $$
if the
![]() $\min $
exists, otherwise let
$\min $
exists, otherwise let
![]() $\ell _x = \lceil (c_n)^k \rceil $
. Given
$\ell _x = \lceil (c_n)^k \rceil $
. Given
![]() $x \in X$
, define the set
$x \in X$
, define the set
![]() $L_n (x) = [ \rho _n (x) \ell _x ]$
. Thus, for infinitely many
$L_n (x) = [ \rho _n (x) \ell _x ]$
. Thus, for infinitely many
![]() $n \geq N$
,
$n \geq N$
,
 $$ \begin{align*} \mu \bigg( \bigcup_{j=-c_n^{k}}^{c_n^{k}} T^{j} (A_n ) \bigg) < \frac{2 a_n^k}{a_{n+1}^p n^2} < \frac{1}{2} \frac{1}{a_n^p n^2} < \frac{1}{2} \mu (B_n). \end{align*} $$
$$ \begin{align*} \mu \bigg( \bigcup_{j=-c_n^{k}}^{c_n^{k}} T^{j} (A_n ) \bigg) < \frac{2 a_n^k}{a_{n+1}^p n^2} < \frac{1}{2} \frac{1}{a_n^p n^2} < \frac{1}{2} \mu (B_n). \end{align*} $$
Let
 $$ \begin{align*} B_n^{\prime} = B_n \setminus \bigg( \bigcup_{j=-c_n^{k}}^{c_n^{k}} T^{j} (A_n ) \bigg). \end{align*} $$
$$ \begin{align*} B_n^{\prime} = B_n \setminus \bigg( \bigcup_{j=-c_n^{k}}^{c_n^{k}} T^{j} (A_n ) \bigg). \end{align*} $$
Hence,
![]() $\mu (B_n^{\prime })> \tfrac 12 \mu (B_n)$
for
$\mu (B_n^{\prime })> \tfrac 12 \mu (B_n)$
for
![]() $n \geq N$
. We break the proof down into four separate cases and handle each separately.
$n \geq N$
. We break the proof down into four separate cases and handle each separately.
-
(1)
 $B_{n,1} = \{ x \in B_n^{\prime } : g(x) < {a_n}/{2}, \ell _x < \lceil ( c_n )^{k} \rceil \}$
.
$B_{n,1} = \{ x \in B_n^{\prime } : g(x) < {a_n}/{2}, \ell _x < \lceil ( c_n )^{k} \rceil \}$
. -
(2)
 $B_{n,2} = \{ x \in B_n^{\prime } : g(x) < {a_n}/{2}, \ell _x \geq \lceil ( c_n )^{k} \rceil \}$
.
$B_{n,2} = \{ x \in B_n^{\prime } : g(x) < {a_n}/{2}, \ell _x \geq \lceil ( c_n )^{k} \rceil \}$
. -
(3)
 $B_{n,3} = \{ x \in B_n^{\prime } : g(x) \geq {a_n}/{2}, \ell _x < \lceil ( c_n )^{k} \rceil \}$
.
$B_{n,3} = \{ x \in B_n^{\prime } : g(x) \geq {a_n}/{2}, \ell _x < \lceil ( c_n )^{k} \rceil \}$
. -
(4)
 $B_{n,4} = \{ x \in B_n^{\prime } : g(x) \geq {a_n}/{2}, \ell _x \geq \lceil ( c_n )^{k} \rceil \}$
.
$B_{n,4} = \{ x \in B_n^{\prime } : g(x) \geq {a_n}/{2}, \ell _x \geq \lceil ( c_n )^{k} \rceil \}$
.
At least one of the
![]() $B_{n,m}$
satisfies
$B_{n,m}$
satisfies
![]() $\mu (B_{n,m}) \geq ({1}/{8}) \mu (B_n)$
for
$\mu (B_{n,m}) \geq ({1}/{8}) \mu (B_n)$
for
![]() $m=1,2,3,4$
. We handle the case
$m=1,2,3,4$
. We handle the case
![]() $\mu (B_{n,1}) \geq ({1}/{8}) \mu (B_n)$
first. We create tiles in the following way. For
$\mu (B_{n,1}) \geq ({1}/{8}) \mu (B_n)$
first. We create tiles in the following way. For
![]() $x \in B_{n,1}$
, let
$x \in B_{n,1}$
, let
By Lemma 4.6 with
![]() $K = \lceil (c_n)^k \rceil $
, there exists
$K = \lceil (c_n)^k \rceil $
, there exists
![]() $J = J_{n,1}$
such that for
$J = J_{n,1}$
such that for
![]() $x \neq y$
,
$x \neq y$
,
![]() $x, y \in J$
,
$x, y \in J$
,
and
 $$ \begin{align*} \mu \bigg( B_{n,1} \cap \bigcup_{x \in J} B_{n,1}(x) \bigg)> \frac{1}{2} \mu (B_{n,1}). \end{align*} $$
$$ \begin{align*} \mu \bigg( B_{n,1} \cap \bigcup_{x \in J} B_{n,1}(x) \bigg)> \frac{1}{2} \mu (B_{n,1}). \end{align*} $$
The
![]() $L^q$
-norm of the transfer function g will blow up on the set J. Before completing the general proof, it is helpful to see how the argument goes in a special case. Suppose
$L^q$
-norm of the transfer function g will blow up on the set J. Before completing the general proof, it is helpful to see how the argument goes in a special case. Suppose
![]() $\ell _x = {a_n}/{a_{n-1}}$
for
$\ell _x = {a_n}/{a_{n-1}}$
for
![]() $x \in J$
. This implies for
$x \in J$
. This implies for
![]() $x\in J$
,
$x\in J$
,
![]() $T^{i}(x) \notin B_n$
of the order of
$T^{i}(x) \notin B_n$
of the order of
![]() ${a_n}/{a_{n-1}}$
times. Also, for this special case,
${a_n}/{a_{n-1}}$
times. Also, for this special case,
![]() $T^i (x)$
cannot fall in
$T^i (x)$
cannot fall in
![]() $B_n$
for
$B_n$
for
![]() $0 < i < \ell _x$
. Note that
$0 < i < \ell _x$
. Note that
![]() $T^i x$
,
$T^i x$
,
![]() $0\leq i < \ell _x$
, does not fall in
$0\leq i < \ell _x$
, does not fall in
![]() $A_n$
by the previous choice of J. However, for
$A_n$
by the previous choice of J. However, for
![]() $x\in J$
, the transfer function at
$x\in J$
, the transfer function at
![]() $T^i x$
will be of the order of the sum, so that
$T^i x$
will be of the order of the sum, so that
![]() $g(T^i x)$
will be of the order of
$g(T^i x)$
will be of the order of
![]() $a_n$
(or
$a_n$
(or
![]() ${a_n}/{4}$
). This implies
${a_n}/{4}$
). This implies
 $$ \begin{align} \int_{X} | g(x) |^q\,d\mu &\approx \bigg( \frac{a_n}{4} \bigg)^{q} \bigg( \frac{a_n}{a_{n-1}} \bigg) \mu(B_n)\end{align} $$
$$ \begin{align} \int_{X} | g(x) |^q\,d\mu &\approx \bigg( \frac{a_n}{4} \bigg)^{q} \bigg( \frac{a_n}{a_{n-1}} \bigg) \mu(B_n)\end{align} $$
 $$ \begin{align} &= \frac{1}{4^q} \frac{a_n^{q+1}}{a_{n-1} a_n^p n^2}\end{align} $$
$$ \begin{align} &= \frac{1}{4^q} \frac{a_n^{q+1}}{a_{n-1} a_n^p n^2}\end{align} $$
 $$ \begin{align} &= \frac{1}{4^q} \frac{a_n^{q+1-p}}{a_{n-1} n^2}. \end{align} $$
$$ \begin{align} &= \frac{1}{4^q} \frac{a_n^{q+1-p}}{a_{n-1} n^2}. \end{align} $$
However, the last term tends to infinity as
![]() $n\to \infty $
by the definition of
$n\to \infty $
by the definition of
![]() $a_n$
and Lemma 4.2.
$a_n$
and Lemma 4.2.
General proof for case 1: First we prove the following lemma.
Lemma 4.7. Let
![]() $1\leq h < k$
, given in the definition of
$1\leq h < k$
, given in the definition of
![]() $\ell _x$
, satisfy
$\ell _x$
, satisfy
![]() $\lceil (c_n)^h \rceil \leq \ell _x < \lceil (c_n)^{h+1} \rceil $
. If
$\lceil (c_n)^h \rceil \leq \ell _x < \lceil (c_n)^{h+1} \rceil $
. If
then
Proof of lemma
Suppose the lemma is not true. Then
 $$ \begin{align} \bigg| g(x) - \operatorname{\mathrm{sgn}} (\rho_n(x)) \sum_{i\in L_n(x) } f(T^i x) \bigg| &\geq \bigg( \sum_{i\in L_n(x) } f(T^i x) - g(x) \bigg) \end{align} $$
$$ \begin{align} \bigg| g(x) - \operatorname{\mathrm{sgn}} (\rho_n(x)) \sum_{i\in L_n(x) } f(T^i x) \bigg| &\geq \bigg( \sum_{i\in L_n(x) } f(T^i x) - g(x) \bigg) \end{align} $$
 $$ \begin{align} &\geq a_{n-1} \ell_x + \frac{2a_{n-1}^2}{a_n} \ell_x \end{align} $$
$$ \begin{align} &\geq a_{n-1} \ell_x + \frac{2a_{n-1}^2}{a_n} \ell_x \end{align} $$
 $$ \begin{align} &\geq \frac{a_n^h}{a_{n-1}^{h-1}} + 2\frac{a_n^{h-1}}{a_{n-1}^{h-2}}> a_n (c_n)^{h-1}. \end{align} $$
$$ \begin{align} &\geq \frac{a_n^h}{a_{n-1}^{h-1}} + 2\frac{a_n^{h-1}}{a_{n-1}^{h-2}}> a_n (c_n)^{h-1}. \end{align} $$
This contradicts the definition of
![]() $\ell _x$
.
$\ell _x$
.
Resume proof of proposition: The measurable set J was constructed such that truncated orbits of points in J are disjoint. In particular, for
![]() $x,y \in J$
,
$x,y \in J$
,
![]() $x\neq y$
, then
$x\neq y$
, then
![]() $\{ x, Tx, \ldots , T^{\ell _x-1}x \} \cap \{ y, Ty, \ldots , T^{\ell _y-1}y \} = \emptyset $
. Thus,
$\{ x, Tx, \ldots , T^{\ell _x-1}x \} \cap \{ y, Ty, \ldots , T^{\ell _y-1}y \} = \emptyset $
. Thus,
 $$ \begin{align} \int_{X} \bigg| g(x) |^q\,d\mu &\geq \int_{J} \sum_{i \in L_n(x)}\bigg| g(T^i x) |^q\,d\mu. \end{align} $$
$$ \begin{align} \int_{X} \bigg| g(x) |^q\,d\mu &\geq \int_{J} \sum_{i \in L_n(x)}\bigg| g(T^i x) |^q\,d\mu. \end{align} $$
Also, since
![]() $x\in B_n^{\prime }$
, then
$x\in B_n^{\prime }$
, then
![]() $f(x)> a_n$
. As noted previously,
$f(x)> a_n$
. As noted previously,
![]() $T^ix \notin A_n$
for
$T^ix \notin A_n$
for
![]() $0\leq i < c_n^k$
. Thus, by the definition of
$0\leq i < c_n^k$
. Thus, by the definition of
![]() $\ell _x$
,
$\ell _x$
,
Hence, we have the following:
 $$ \begin{align} \int_{X} | g(x) |^q\,d\mu &\geq \int_{J} \sum_{i \in L_n(x)} | g(T^i x) |^q\,d\mu\end{align} $$
$$ \begin{align} \int_{X} | g(x) |^q\,d\mu &\geq \int_{J} \sum_{i \in L_n(x)} | g(T^i x) |^q\,d\mu\end{align} $$
 $$ \begin{align} &= \int_{J} \sum_{i \in L_n(x)} \bigg| g(x) - \sum_{j \in [i] } f(T^j x) \bigg|^q\,d\mu \end{align} $$
$$ \begin{align} &= \int_{J} \sum_{i \in L_n(x)} \bigg| g(x) - \sum_{j \in [i] } f(T^j x) \bigg|^q\,d\mu \end{align} $$
 $$ \begin{align} &\geq \int_{J} \sum_{i \in L_n(x)} \bigg| \frac{a_{n}}{4} \bigg|^q\,d\mu\end{align} $$
$$ \begin{align} &\geq \int_{J} \sum_{i \in L_n(x)} \bigg| \frac{a_{n}}{4} \bigg|^q\,d\mu\end{align} $$
 $$ \begin{align} &= \bigg| \frac{a_n}{4} \bigg|^q \int_J \ell_x\,d\mu\end{align} $$
$$ \begin{align} &= \bigg| \frac{a_n}{4} \bigg|^q \int_J \ell_x\,d\mu\end{align} $$
 $$ \begin{align} &> \bigg| \frac{a_n}{4} \bigg|^q \int_{J} \frac{1}{2} \bigg( \frac{a_n}{a_{n-1}} \bigg) \sum_{i \in L_n(x) } I_{B_n} (T^i x)\,d\mu\end{align} $$
$$ \begin{align} &> \bigg| \frac{a_n}{4} \bigg|^q \int_{J} \frac{1}{2} \bigg( \frac{a_n}{a_{n-1}} \bigg) \sum_{i \in L_n(x) } I_{B_n} (T^i x)\,d\mu\end{align} $$
 $$ \begin{align} &> \bigg| \frac{a_n}{4} \bigg|^q \frac{1}{2} \bigg( \frac{a_n}{a_{n-1}} \bigg) \bigg( \frac{1}{2} \mu (B_{n,1}) \bigg)\end{align} $$
$$ \begin{align} &> \bigg| \frac{a_n}{4} \bigg|^q \frac{1}{2} \bigg( \frac{a_n}{a_{n-1}} \bigg) \bigg( \frac{1}{2} \mu (B_{n,1}) \bigg)\end{align} $$
 $$ \begin{align} &> \bigg( \frac{1}{32} \bigg) \bigg| \frac{a_n}{4} \bigg|^q \bigg( \frac{a_n}{a_{n-1}} \bigg) \mu (B_{n})\end{align} $$
$$ \begin{align} &> \bigg( \frac{1}{32} \bigg) \bigg| \frac{a_n}{4} \bigg|^q \bigg( \frac{a_n}{a_{n-1}} \bigg) \mu (B_{n})\end{align} $$
 $$ \begin{align} &\geq \frac{a_n^{q+1}}{32 (4^q) a_{n-1} a_n^p n^2} \end{align} $$
$$ \begin{align} &\geq \frac{a_n^{q+1}}{32 (4^q) a_{n-1} a_n^p n^2} \end{align} $$
 $$ \begin{align} &= \frac{a_n^{q+1-p}}{32 (4^q) a_{n-1} n^2}. \end{align} $$
$$ \begin{align} &= \frac{a_n^{q+1-p}}{32 (4^q) a_{n-1} n^2}. \end{align} $$
The proof for this case is complete, since, by Lemma 4.2,
 $$ \begin{align*} \lim_{n\to \infty} \frac{a_n^{q+1-p}}{32 (4^q) a_{n-1} n^2} = \infty. \end{align*} $$
$$ \begin{align*} \lim_{n\to \infty} \frac{a_n^{q+1-p}}{32 (4^q) a_{n-1} n^2} = \infty. \end{align*} $$
Proof for case 2:
 $$ \begin{align} \int_{B_n} | g(x) |^q\,d\mu &\geq \int_{J} \sum_{i \in L_n(x)} | g(T^i x) |^q I_{B_n} (T^i x)\,d\mu\end{align} $$
$$ \begin{align} \int_{B_n} | g(x) |^q\,d\mu &\geq \int_{J} \sum_{i \in L_n(x)} | g(T^i x) |^q I_{B_n} (T^i x)\,d\mu\end{align} $$
 $$ \begin{align} &= \int_{J} \sum_{i \in L_n(x)} \bigg| g(x) - \operatorname{\mathrm{sgn}}(x) \sum_{ j \in [-i] } f(T^j x)\bigg|^q I_{B_n} (T^i x)\,d\mu\end{align} $$
$$ \begin{align} &= \int_{J} \sum_{i \in L_n(x)} \bigg| g(x) - \operatorname{\mathrm{sgn}}(x) \sum_{ j \in [-i] } f(T^j x)\bigg|^q I_{B_n} (T^i x)\,d\mu\end{align} $$
 $$ \begin{align} &\geq \int_{J} \sum_{i = c_n^{k-1}}^{c_n^k} \bigg| g(x) - \operatorname{\mathrm{sgn}}(x) \sum_{ j \in [-i] } f(T^j x) \bigg|^q I_{B_n} (T^{-i} x)\,d\mu\end{align} $$
$$ \begin{align} &\geq \int_{J} \sum_{i = c_n^{k-1}}^{c_n^k} \bigg| g(x) - \operatorname{\mathrm{sgn}}(x) \sum_{ j \in [-i] } f(T^j x) \bigg|^q I_{B_n} (T^{-i} x)\,d\mu\end{align} $$
 $$ \begin{align} &\geq \sum_{i = c_n^{k-1}}^{c_n^k} \int_{J} \bigg( \bigg( \frac{a_n}{4} \bigg) \bigg( \frac{a_n}{a_{n-1}} \bigg)^{k-1} \bigg)^q I_{B_n} (T^i x)\,d\mu\end{align} $$
$$ \begin{align} &\geq \sum_{i = c_n^{k-1}}^{c_n^k} \int_{J} \bigg( \bigg( \frac{a_n}{4} \bigg) \bigg( \frac{a_n}{a_{n-1}} \bigg)^{k-1} \bigg)^q I_{B_n} (T^i x)\,d\mu\end{align} $$
 $$ \begin{align} &\geq \bigg( \frac{c_n^k - c_n^{k-1}}{c_n^k} \bigg) \bigg( \frac{1}{16} \bigg) \mu(B_n) \bigg( \bigg( \frac{a_n}{4} \bigg) \bigg( \frac{a_n}{a_{n-1}} \bigg)^{k-1} \bigg)^q\end{align} $$
$$ \begin{align} &\geq \bigg( \frac{c_n^k - c_n^{k-1}}{c_n^k} \bigg) \bigg( \frac{1}{16} \bigg) \mu(B_n) \bigg( \bigg( \frac{a_n}{4} \bigg) \bigg( \frac{a_n}{a_{n-1}} \bigg)^{k-1} \bigg)^q\end{align} $$
 $$ \begin{align} &> \bigg( \frac{1}{32} \bigg) \bigg( \frac{a_n^{kq}}{ a_n^p n^2 4^q a_{n-1}^{q(k-1)} } \bigg)\end{align} $$
$$ \begin{align} &> \bigg( \frac{1}{32} \bigg) \bigg( \frac{a_n^{kq}}{ a_n^p n^2 4^q a_{n-1}^{q(k-1)} } \bigg)\end{align} $$
 $$ \begin{align} &= \bigg( \frac{1}{32} \bigg) \bigg( \frac{a_n^{kq-p}}{ n^2 4^q a_{n-1}^{q(k-1)} } \bigg). \end{align} $$
$$ \begin{align} &= \bigg( \frac{1}{32} \bigg) \bigg( \frac{a_n^{kq-p}}{ n^2 4^q a_{n-1}^{q(k-1)} } \bigg). \end{align} $$
Since
 $$ \begin{align*} \lim_{n\to \infty} \bigg( \frac{1}{32} \bigg) \bigg( \frac{a_n^{kq-p}}{ n^2 4^q a_{n-1}^{q(k-1)} } \bigg) = \infty , \end{align*} $$
$$ \begin{align*} \lim_{n\to \infty} \bigg( \frac{1}{32} \bigg) \bigg( \frac{a_n^{kq-p}}{ n^2 4^q a_{n-1}^{q(k-1)} } \bigg) = \infty , \end{align*} $$
then our result follows for case 2.
Case 3 would be handled in a similar manner as case 1, except we would base our estimate of
![]() $g(x)$
on the inverse of T. Thus, we have the following:
$g(x)$
on the inverse of T. Thus, we have the following:
 $$ \begin{align} \int_{X} | g(x) |^q\,d\mu &\geq \int_{J} \sum_{i \in L_n(x)} | g(T^i x) |^q\,d\mu\end{align} $$
$$ \begin{align} \int_{X} | g(x) |^q\,d\mu &\geq \int_{J} \sum_{i \in L_n(x)} | g(T^i x) |^q\,d\mu\end{align} $$
 $$ \begin{align} &= \int_{J} \sum_{i \in L_n(x)} \bigg| g(x) + \sum_{j \in [i] } f(T^j x) \bigg|^q\,d\mu\end{align} $$
$$ \begin{align} &= \int_{J} \sum_{i \in L_n(x)} \bigg| g(x) + \sum_{j \in [i] } f(T^j x) \bigg|^q\,d\mu\end{align} $$
 $$ \begin{align} &\geq \int_{J} \sum_{i \in L_n(x)}\bigg| \frac{a_{n}}{4} \bigg|^q\, d\mu. \end{align} $$
$$ \begin{align} &\geq \int_{J} \sum_{i \in L_n(x)}\bigg| \frac{a_{n}}{4} \bigg|^q\, d\mu. \end{align} $$
The next steps continue in a similar manner as case 1. Also, case 4 follows in a similar manner as case 2, except by using
![]() $T^{-1}$
instead of T.
$T^{-1}$
instead of T.
Proof of Theorem 1.2
Define
 $$ \begin{align*} \mathcal{G}_p = \bigcap_{n=1}^{\infty} \mathcal{G}^p_n. \end{align*} $$
$$ \begin{align*} \mathcal{G}_p = \bigcap_{n=1}^{\infty} \mathcal{G}^p_n. \end{align*} $$
By Lemmas 4.3 and 4.4, the set
![]() $\mathcal {G}_p$
is a dense
$\mathcal {G}_p$
is a dense
![]() $G_{\delta }$
subset of
$G_{\delta }$
subset of
![]() $L^p_0$
. Also, by Proposition 4.5,
$L^p_0$
. Also, by Proposition 4.5,
![]() $f \in \mathcal {G}_p$
satisfies the conditions of Theorem 1.2.
$f \in \mathcal {G}_p$
satisfies the conditions of Theorem 1.2.
4.3 Not a moment
Let
![]() be a measurable function such that
be a measurable function such that
For
![]() , let
, let
![]() $A, B_i$
be disjoint sets in X, and
$A, B_i$
be disjoint sets in X, and
![]() $b_i> 0$
. Define f as
$b_i> 0$
. Define f as
 $$ \begin{align*} f = I_{A} - \sum_{i=1}^{\infty} b_i I_{B_i}. \end{align*} $$
$$ \begin{align*} f = I_{A} - \sum_{i=1}^{\infty} b_i I_{B_i}. \end{align*} $$
We will give conditions on the fast growth rate of
![]() $b_i$
as well as conditions on the sets
$b_i$
as well as conditions on the sets
![]() $A, B_i$
to guarantee that f is contained in
$A, B_i$
to guarantee that f is contained in
![]() $L^1$
, but such that
$L^1$
, but such that
![]() $\phi \circ \vert g \vert $
is not in
$\phi \circ \vert g \vert $
is not in
![]() $L^1$
for any transfer function g of an ergodic invertible measure-preserving transformation T. Let
$L^1$
for any transfer function g of an ergodic invertible measure-preserving transformation T. Let
![]() $A \subset X$
have measure
$A \subset X$
have measure
![]() $\mu (A) = {1}/{2}$
. Choose
$\mu (A) = {1}/{2}$
. Choose
![]() $b_i> 0$
for
$b_i> 0$
for
![]() such that
such that
![]() $\lim _{i\to \infty } b_i = \infty $
, and such that for all real
$\lim _{i\to \infty } b_i = \infty $
, and such that for all real
![]() $\alpha> 0$
,
$\alpha> 0$
,
 $$ \begin{align} \lim_{i\to \infty} \frac{b_i}{b_{i+1}^{\alpha}} = 0, \end{align} $$
$$ \begin{align} \lim_{i\to \infty} \frac{b_i}{b_{i+1}^{\alpha}} = 0, \end{align} $$
and also for
![]() $y \geq {b_i} / {4}$
,
$y \geq {b_i} / {4}$
,
It is possible to satisfy condition (4.35) by taking a faster growing subsequence for
![]() $b_i$
. Choose disjoint sets
$b_i$
. Choose disjoint sets
![]() $B_i \subset A^c$
such that
$B_i \subset A^c$
such that
Observe that
![]() $f\in L^1$
is mean zero.
$f\in L^1$
is mean zero.
Proposition 4.8. Let
![]() be a measurable function satisfying
be a measurable function satisfying
![]() $\lim _{x\to \infty } \phi (x) = \infty $
. Suppose the mean-zero function
$\lim _{x\to \infty } \phi (x) = \infty $
. Suppose the mean-zero function
![]() $f = I_{A} - \sum _{i=1}^{\infty } b_i I_{B_i}$
satisfies the conditions above, including (4.34), (4.35), and (4.36). If T is an ergodic invertible measure-preserving transformation
$f = I_{A} - \sum _{i=1}^{\infty } b_i I_{B_i}$
satisfies the conditions above, including (4.34), (4.35), and (4.36). If T is an ergodic invertible measure-preserving transformation
![]() $T: X \to X$
and g is a transfer function satisfying
$T: X \to X$
and g is a transfer function satisfying
![]() $f(x) = g(Tx) - g(x)$
for a.e.
$f(x) = g(Tx) - g(x)$
for a.e.
![]() $x\in X$
, then
$x\in X$
, then
Proof. Let
![]() $\operatorname {\mathrm {sgn}}$
be the standard sign function defined as
$\operatorname {\mathrm {sgn}}$
be the standard sign function defined as
![]() $\operatorname {\mathrm {sgn}}(i)=-1$
if
$\operatorname {\mathrm {sgn}}(i)=-1$
if
![]() $i<0$
,
$i<0$
,
![]() $\operatorname {\mathrm {sgn}}(i)=0$
if
$\operatorname {\mathrm {sgn}}(i)=0$
if
![]() $i=0$
, and
$i=0$
, and
![]() $\operatorname {\mathrm {sgn}}(i)=1$
if
$\operatorname {\mathrm {sgn}}(i)=1$
if
![]() $i>0$
. For
$i>0$
. For
![]() $i \in \mathbb {Z}$
, let
$i \in \mathbb {Z}$
, let
![]() $[ i ] = \{ j \in \mathbb {Z} : i \leq j < 0 \}$
if
$[ i ] = \{ j \in \mathbb {Z} : i \leq j < 0 \}$
if
![]() $i < 0$
, and
$i < 0$
, and
![]() $[ i ] = \{ j \in \mathbb {Z} : 0 \leq j < i \}$
if
$[ i ] = \{ j \in \mathbb {Z} : 0 \leq j < i \}$
if
![]() $i \geq 0$
. Note, for
$i \geq 0$
. Note, for
![]() $i \in \mathbb {Z}$
, the coboundary equation expands to the following:
$i \in \mathbb {Z}$
, the coboundary equation expands to the following:
 $$ \begin{align*} g(T^i x) = g(x) + \operatorname{\mathrm{sgn}} (i) \sum_{j \in [i]} f ( T^j x). \end{align*} $$
$$ \begin{align*} g(T^i x) = g(x) + \operatorname{\mathrm{sgn}} (i) \sum_{j \in [i]} f ( T^j x). \end{align*} $$
Define our specialized sign function
![]() $\rho : X \to \{ -1, 1 \}$
based on the following:
$\rho : X \to \{ -1, 1 \}$
based on the following:
-
(1) if
 $g(x) \leq {b_n}/{2}$
, let
$g(x) \leq {b_n}/{2}$
, let
 $\rho (x)=1$
;
$\rho (x)=1$
; -
(2) otherwise if
 $g(x)> {b_n}/{2}$
, then let
$g(x)> {b_n}/{2}$
, then let
 $\rho (x)=-1$
.
$\rho (x)=-1$
.
Assume
![]() $f \in L^1$
. For
$f \in L^1$
. For
![]() $x \in B_n$
, let
$x \in B_n$
, let
 $$ \begin{align*} \ell_x = \min{ \bigg\{ \ell : \ell> 0 , | g( T^{ \rho(x) \ell } x ) | < \frac{ b_n }{4} ( b_n )^{h-1} , \lceil ( b_n )^{h} \rceil \leq \ell < \lceil ( b_n )^{h+1} \rceil \bigg\} }. \end{align*} $$
$$ \begin{align*} \ell_x = \min{ \bigg\{ \ell : \ell> 0 , | g( T^{ \rho(x) \ell } x ) | < \frac{ b_n }{4} ( b_n )^{h-1} , \lceil ( b_n )^{h} \rceil \leq \ell < \lceil ( b_n )^{h+1} \rceil \bigg\} }. \end{align*} $$
Note,
![]() $\ell _x < \infty $
for a.e.
$\ell _x < \infty $
for a.e.
![]() $x \in X$
, otherwise our result follows directly. Thus, exclude points
$x \in X$
, otherwise our result follows directly. Thus, exclude points
![]() $x \in X$
where
$x \in X$
where
![]() $\ell _x = \infty $
. Choose
$\ell _x = \infty $
. Choose
![]() such that
such that
Given
![]() $x \in X$
, define the set
$x \in X$
, define the set
![]() $K_n(x) = [ \tfrac 12 \rho (x) \ell _x ]$
. We do not need to consider all of the cases as in Proposition 4.5, due to the special nature of the counterexamples f in this result. We create tiles in the following way. For
$K_n(x) = [ \tfrac 12 \rho (x) \ell _x ]$
. We do not need to consider all of the cases as in Proposition 4.5, due to the special nature of the counterexamples f in this result. We create tiles in the following way. For
![]() $x \in B_n$
, let
$x \in B_n$
, let
There exists
![]() $J_n$
such that for
$J_n$
such that for
![]() $x \neq y$
,
$x \neq y$
,
![]() $x, y \in J_n$
,
$x, y \in J_n$
,
and
 $$ \begin{align*} \mu \bigg( B_{n} \cap \bigcup_{x \in J_n} B_{n}(x) \bigg)> \frac{1}{4} \mu (B_{n}). \end{align*} $$
$$ \begin{align*} \mu \bigg( B_{n} \cap \bigcup_{x \in J_n} B_{n}(x) \bigg)> \frac{1}{4} \mu (B_{n}). \end{align*} $$
First we prove the following lemma.
Lemma 4.9. Suppose
![]() $\lceil (b_n)^h \rceil \leq \ell _x < \lceil (b_n)^{h+1} \rceil $
for
$\lceil (b_n)^h \rceil \leq \ell _x < \lceil (b_n)^{h+1} \rceil $
for
![]() $1 \leq h < k_n+1$
. If
$1 \leq h < k_n+1$
. If
then
Proof of lemma Suppose the lemma is not true. Then
 $$ \begin{align} \bigg| g(x) + \operatorname{\mathrm{sgn}} (x) \sum_{i\in [\rho(x) \ell_x] } f(T^i x) \bigg| &\geq \ell_0 b_n - ( \ell_x - \ell_0 ) = \ell_0 b_n - \ell_x + \ell_0\end{align} $$
$$ \begin{align} \bigg| g(x) + \operatorname{\mathrm{sgn}} (x) \sum_{i\in [\rho(x) \ell_x] } f(T^i x) \bigg| &\geq \ell_0 b_n - ( \ell_x - \ell_0 ) = \ell_0 b_n - \ell_x + \ell_0\end{align} $$
This contradicts the definition of
![]() $\ell _x$
.
$\ell _x$
.
Resume proof of proposition: Thus, we have the following:
 $$ \begin{align} \int_{X} \phi ( | g(x) | )\,d\mu &\geq \int_{J_n} \sum_{i \in K_n(x)} \phi ( | g(T^i x) | )\,d\mu\end{align} $$
$$ \begin{align} \int_{X} \phi ( | g(x) | )\,d\mu &\geq \int_{J_n} \sum_{i \in K_n(x)} \phi ( | g(T^i x) | )\,d\mu\end{align} $$
 $$ \begin{align} &= \int_{J_n} \sum_{i \in K_n(x)} \phi \bigg( \bigg| g(x) + \sum_{j \in [i] } f(T^j x)\bigg| \bigg)\,d\mu\end{align} $$
$$ \begin{align} &= \int_{J_n} \sum_{i \in K_n(x)} \phi \bigg( \bigg| g(x) + \sum_{j \in [i] } f(T^j x)\bigg| \bigg)\,d\mu\end{align} $$
 $$ \begin{align} &\geq \int_{J_n} n 2^n \ell_x\,d\mu\end{align} $$
$$ \begin{align} &\geq \int_{J_n} n 2^n \ell_x\,d\mu\end{align} $$
 $$ \begin{align} &> n 2^n \int_{J_n} \frac{1}{2} ( b_n ) \sum_{i \in K_n(x) } I_{B_n} (T^i x)\,d\mu \end{align} $$
$$ \begin{align} &> n 2^n \int_{J_n} \frac{1}{2} ( b_n ) \sum_{i \in K_n(x) } I_{B_n} (T^i x)\,d\mu \end{align} $$
5 Category of transformation solutions
This section contains two propositions. The first proposition gives a general condition for the existence of solutions to the coboundary equation when f is not integrable. The second proposition shows the class of transformations with a measurable solution is meager for any measurable function f.
Proposition 5.1. Suppose
![]() is a measurable function. The coboundary equation
is a measurable function. The coboundary equation
![]() $f = g - g \circ T$
has solutions
$f = g - g \circ T$
has solutions
![]() $T \in \mathcal {E}$
,
$T \in \mathcal {E}$
,
![]() $g \in L^0$
, if and only if,
$g \in L^0$
, if and only if,
 $$ \begin{align*} \int_{f> 0} f\,d\mu = \int_{f < 0} ( - f ) \,d \mu \ \ (\infty\textrm{ or finite}). \end{align*} $$
$$ \begin{align*} \int_{f> 0} f\,d\mu = \int_{f < 0} ( - f ) \,d \mu \ \ (\infty\textrm{ or finite}). \end{align*} $$
Proof. The case where both
![]() $\int _{f> 0} f\,d\mu $
and
$\int _{f> 0} f\,d\mu $
and
![]() $\int _{f < 0} ( - f )\,d\mu $
are finite and unequal is already covered by Anosov’s result [Reference Anosov4]. If the integrals are finite and equal, it follows from Theorem 1.1.
$\int _{f < 0} ( - f )\,d\mu $
are finite and unequal is already covered by Anosov’s result [Reference Anosov4]. If the integrals are finite and equal, it follows from Theorem 1.1.
Next, we prove the case where one integral is finite and the other is infinite. Without loss of generality, assume
![]() $\int _{f> 0} f\,d\mu = \infty $
and
$\int _{f> 0} f\,d\mu = \infty $
and
![]() $\int _{f < 0} ( - f )\,d\mu < \infty $
. Choose a measurable subset
$\int _{f < 0} ( - f )\,d\mu < \infty $
. Choose a measurable subset
![]() $A \subset \{ f> 0 \}$
such that
$A \subset \{ f> 0 \}$
such that
 $$ \begin{align*} \int_{A} f\,d\mu + \int_{f < 0} f\,d\mu = 1. \end{align*} $$
$$ \begin{align*} \int_{A} f\,d\mu + \int_{f < 0} f\,d\mu = 1. \end{align*} $$
Let
 $$ \begin{align*} f_0(x)= \begin{cases} f(x) & \textrm{if}\ x \in A \cup \{ f < 0 \} , \\ 0 & \textrm{if}\ x \in \{ f> 0 \} \setminus A. \end{cases} \end{align*} $$
$$ \begin{align*} f_0(x)= \begin{cases} f(x) & \textrm{if}\ x \in A \cup \{ f < 0 \} , \\ 0 & \textrm{if}\ x \in \{ f> 0 \} \setminus A. \end{cases} \end{align*} $$
Thus,
![]() $f_0 \in L^1$
and
$f_0 \in L^1$
and
![]() $\int _X f_0\,d\mu = 1$
. Given
$\int _X f_0\,d\mu = 1$
. Given
![]() $T \in \mathcal {E}$
, by the mean ergodic theorem,
$T \in \mathcal {E}$
, by the mean ergodic theorem,
 $$ \begin{align*} \lim_{n \to \infty} \int_X \bigg| \frac{1}{n} \sum_{i=0}^{n-1} f_0 (T^i x) - \int_X f_0\,d\mu\,\bigg|\,d\mu = 0. \end{align*} $$
$$ \begin{align*} \lim_{n \to \infty} \int_X \bigg| \frac{1}{n} \sum_{i=0}^{n-1} f_0 (T^i x) - \int_X f_0\,d\mu\,\bigg|\,d\mu = 0. \end{align*} $$
Let
![]() $\delta> 0$
. Then
$\delta> 0$
. Then
![]() $\mu \{ x \in X : | {1}/{n} \sum _{i=0}^{n-1} f_0 (T^i x) - 1 | < \delta \} \to 1$
as
$\mu \{ x \in X : | {1}/{n} \sum _{i=0}^{n-1} f_0 (T^i x) - 1 | < \delta \} \to 1$
as
![]() $n \to \infty $
. Hence,
$n \to \infty $
. Hence,
 $$ \begin{align*} \lim_{n \to \infty} \mu \bigg\{ x \in X : \sum_{i=0}^{n-1} f_0 (T^i x)> n ( 1 - \delta ) \bigg\} = 1. \end{align*} $$
$$ \begin{align*} \lim_{n \to \infty} \mu \bigg\{ x \in X : \sum_{i=0}^{n-1} f_0 (T^i x)> n ( 1 - \delta ) \bigg\} = 1. \end{align*} $$
Since
![]() $\sum _{i=0}^{n-1} f (T^i x) \geq \sum _{i=0}^{n-1} f_0 (T^i x)$
for a.e.
$\sum _{i=0}^{n-1} f (T^i x) \geq \sum _{i=0}^{n-1} f_0 (T^i x)$
for a.e.
![]() $x \in X$
, then
$x \in X$
, then
 $$ \begin{align*} \lim_{n \to \infty} \mu \bigg\{ x \in X : \sum_{i=0}^{n-1} f (T^i x)> n ( 1 - \delta ) \bigg\} = 1. \end{align*} $$
$$ \begin{align*} \lim_{n \to \infty} \mu \bigg\{ x \in X : \sum_{i=0}^{n-1} f (T^i x)> n ( 1 - \delta ) \bigg\} = 1. \end{align*} $$
Since the Halász–Schmidt condition (2.1) does not hold, there is no measurable solution g.
The final case to prove is where
![]() $\int _{f> 0} f\,d\mu = \int _{f < 0} ( - f )\,d\mu = \infty $
. It is proved using a construction similar to the one used in Theorem 1.1. Choose disjoint measurable sets
$\int _{f> 0} f\,d\mu = \int _{f < 0} ( - f )\,d\mu = \infty $
. It is proved using a construction similar to the one used in Theorem 1.1. Choose disjoint measurable sets
![]() $X_n \subset ~X$
for
$X_n \subset ~X$
for
![]() such that f is bounded on
such that f is bounded on
![]() $X_n$
,
$X_n$
,
![]() $\int _{X_n} f\,d\mu = 0$
, and
$\int _{X_n} f\,d\mu = 0$
, and
 $$ \begin{align*} \mu \bigg( \bigcup_{i=0}^{\infty} X_i \bigg) = 1. \end{align*} $$
$$ \begin{align*} \mu \bigg( \bigcup_{i=0}^{\infty} X_i \bigg) = 1. \end{align*} $$
Let
![]() $\epsilon _{n,i}> 0$
for
$\epsilon _{n,i}> 0$
for
![]() be such that
be such that
![]() $\sum _{n=1}^{\infty } \sum _{i=1}^{\infty } \epsilon _{n,i} < \infty $
. By Lemma 3.5, for each
$\sum _{n=1}^{\infty } \sum _{i=1}^{\infty } \epsilon _{n,i} < \infty $
. By Lemma 3.5, for each
![]() , there exist invertible measure-preserving
, there exist invertible measure-preserving
![]() $T_n$
, disjoint
$T_n$
, disjoint
![]() $I_{n,i} \subset X_n$
, and
$I_{n,i} \subset X_n$
, and
![]() , such that:
, such that:
-
•
 $X_n = \bigcup _{i=1}^{\infty } \bigcup _{j=0}^{h_{n,i}-1} T_n^j I_{n,i}$
is a disjoint union;
$X_n = \bigcup _{i=1}^{\infty } \bigcup _{j=0}^{h_{n,i}-1} T_n^j I_{n,i}$
is a disjoint union; -
•
 $| \sum _{j=0}^{h_{n,i}-1} f(T_n^j x) | < \epsilon _{n,i}$
;
$| \sum _{j=0}^{h_{n,i}-1} f(T_n^j x) | < \epsilon _{n,i}$
; -
•
 $| \sum _{j=0}^{h_{n,i}-1} f(T_n^j x) | < \|f\|_{\infty } + \epsilon _{n,i}$
.
$| \sum _{j=0}^{h_{n,i}-1} f(T_n^j x) | < \|f\|_{\infty } + \epsilon _{n,i}$
.
Note each
![]() $T_n$
is defined on
$T_n$
is defined on
![]() $X_n$
except for the top levels of each tower. Let T be the join of all
$X_n$
except for the top levels of each tower. Let T be the join of all
![]() $T_n$
:
$T_n$
:
 $$ \begin{align*} Tx = T_n x\quad \textrm{for } x\in X_n\setminus \bigg(\bigcup_{i=1}^{\infty} T_n^{h_{n,i}-1} I_{n,i} \bigg). \end{align*} $$
$$ \begin{align*} Tx = T_n x\quad \textrm{for } x\in X_n\setminus \bigg(\bigcup_{i=1}^{\infty} T_n^{h_{n,i}-1} I_{n,i} \bigg). \end{align*} $$
Define the set
![]() $A\subset X$
as
$A\subset X$
as
 $$ \begin{align*} A = \bigcup_{n=1}^{\infty} \bigcup_{i=1}^{\infty} I_{n,i}. \end{align*} $$
$$ \begin{align*} A = \bigcup_{n=1}^{\infty} \bigcup_{i=1}^{\infty} I_{n,i}. \end{align*} $$
Define
![]() $f_A(x) = \sum _{j=0}^{h_{n,i}-1} f(T^j x)$
for
$f_A(x) = \sum _{j=0}^{h_{n,i}-1} f(T^j x)$
for
![]() $x\in I_{n,i}$
. Note that
$x\in I_{n,i}$
. Note that
![]() $f_A$
is mean zero, since
$f_A$
is mean zero, since
 $$ \begin{align} \int_{A} f_A\,d\mu &= \sum_{n=1}^{\infty} \sum_{i=1}^{\infty} \int_{I_{n,i}} \sum_{j=0}^{h_{n,i}-1} f(T^j x)\,d\mu = \sum_{n=1}^{\infty} \int_{X_n} f\,d\mu = 0. \end{align} $$
$$ \begin{align} \int_{A} f_A\,d\mu &= \sum_{n=1}^{\infty} \sum_{i=1}^{\infty} \int_{I_{n,i}} \sum_{j=0}^{h_{n,i}-1} f(T^j x)\,d\mu = \sum_{n=1}^{\infty} \int_{X_n} f\,d\mu = 0. \end{align} $$
Since
![]() $f_A$
is bounded and mean zero, by Proposition 3.6, there exist
$f_A$
is bounded and mean zero, by Proposition 3.6, there exist
![]() $\tau :A \to A$
and
$\tau :A \to A$
and
![]() $g_A$
such that
$g_A$
such that
![]() $f_A = g_A \circ \tau - g_A$
almost everywhere. There exists a unique extension of
$f_A = g_A \circ \tau - g_A$
almost everywhere. There exists a unique extension of
![]() $T:X\to X$
(up to a set of measure zero) such that the induced transformation
$T:X\to X$
(up to a set of measure zero) such that the induced transformation
![]() $T_A = \tau $
almost everywhere. By Lemma 3.2,
$T_A = \tau $
almost everywhere. By Lemma 3.2,
Also, the explicit transfer function g, defined by Lemma 3.2, is measurable, since
![]() $g_A$
is measurable.
$g_A$
is measurable.
Now we are ready to prove the class of transformations with a measurable solution is a first category set (meager).
Proposition 5.2. Let f be a measurable function such that
![]() $\mu (\{ x : f(x) \neq 0 \})> 0$
. Let
$\mu (\{ x : f(x) \neq 0 \})> 0$
. Let
![]() $\mathcal {T}$
be the set of ergodic invertible measure-preserving transformations T such that
$\mathcal {T}$
be the set of ergodic invertible measure-preserving transformations T such that
![]() $f = g - g\circ T$
has a measurable solution g. The set
$f = g - g\circ T$
has a measurable solution g. The set
![]() $\mathcal {T}$
is a set of first category (meager).
$\mathcal {T}$
is a set of first category (meager).
Proof. Let
![]() such that
such that
![]() $0 < \eta < {1} / {10}$
. For each
$0 < \eta < {1} / {10}$
. For each
![]() , define
, define
 $$ \begin{align*} D_n = \bigg\{ T \in \mathcal{E} : \text{there exists } k> n \textrm{ such that } \mu \bigg( \bigg\{ x: \bigg| \sum_{i=0}^{k-1} f(T^i x) \bigg| > n \bigg\} \bigg) > \eta \bigg\}. \end{align*} $$
$$ \begin{align*} D_n = \bigg\{ T \in \mathcal{E} : \text{there exists } k> n \textrm{ such that } \mu \bigg( \bigg\{ x: \bigg| \sum_{i=0}^{k-1} f(T^i x) \bigg| > n \bigg\} \bigg) > \eta \bigg\}. \end{align*} $$
For each
![]() , the set
, the set
![]() $D_n$
is both open and dense. Establishing open-ness is straightforward. Let
$D_n$
is both open and dense. Establishing open-ness is straightforward. Let
![]() $T \in D_n$
. There exists
$T \in D_n$
. There exists
![]() $\delta _0> 0$
such that
$\delta _0> 0$
such that
 $$ \begin{align*} \mu \bigg( \bigg\{ x: \bigg| \sum_{i=0}^{k-1} f(T^i x) \bigg|> n + \delta_0 \bigg\} \bigg) > \eta. \end{align*} $$
$$ \begin{align*} \mu \bigg( \bigg\{ x: \bigg| \sum_{i=0}^{k-1} f(T^i x) \bigg|> n + \delta_0 \bigg\} \bigg) > \eta. \end{align*} $$
Thus, if
![]() $\eta _0 = \mu ( \{ x: | \sum _{i=0}^{k-1} f(T^i x) |> n + \delta _0 \} )$
, then
$\eta _0 = \mu ( \{ x: | \sum _{i=0}^{k-1} f(T^i x) |> n + \delta _0 \} )$
, then
 $$ \begin{align*} \bigg\{ S \in \mathcal{E} : \int_{X} | f\circ S^i - f\circ T^i |\,d\mu < \frac{\delta_0 ( \eta_0 - \eta )}{k} \bigg\} \end{align*} $$
$$ \begin{align*} \bigg\{ S \in \mathcal{E} : \int_{X} | f\circ S^i - f\circ T^i |\,d\mu < \frac{\delta_0 ( \eta_0 - \eta )}{k} \bigg\} \end{align*} $$
is an open neighborhood containing T and contained in
![]() $D_n$
.
$D_n$
.
To establish that
![]() $D_n$
is dense, it can be accomplished by an application of the ergodic theorem. Let
$D_n$
is dense, it can be accomplished by an application of the ergodic theorem. Let
![]() $S\in \mathcal {E}$
and
$S\in \mathcal {E}$
and
![]() be such that
be such that
![]() ${1} / {20}> \epsilon > 0$
. If
${1} / {20}> \epsilon > 0$
. If
![]() $S \in D_n$
, then we set
$S \in D_n$
, then we set
![]() $T = S$
. Otherwise, assume
$T = S$
. Otherwise, assume
![]() $S \notin D_n$
. Choose
$S \notin D_n$
. Choose
![]() $\alpha> 0$
such that the set
$\alpha> 0$
such that the set
![]() $A = \{ x \in X : f(x)> \alpha \}$
has positive measure. Similarly, choose
$A = \{ x \in X : f(x)> \alpha \}$
has positive measure. Similarly, choose
![]() $\beta> 0$
such that the set
$\beta> 0$
such that the set
![]() $B = \{ x \in X : f(x) < -\beta \}$
has positive measure. Let
$B = \{ x \in X : f(x) < -\beta \}$
has positive measure. Let
![]() be such that
be such that
 $$ \begin{align*} \gamma \geq \max{\!\bigg\{\frac{2n}{\alpha} , \frac{2n}{\beta} \bigg\}}. \end{align*} $$
$$ \begin{align*} \gamma \geq \max{\!\bigg\{\frac{2n}{\alpha} , \frac{2n}{\beta} \bigg\}}. \end{align*} $$
Choose
![]() $\ell _0> n$
such that for
$\ell _0> n$
such that for
![]() $\ell \geq \ell _0$
,
$\ell \geq \ell _0$
,
 $$ \begin{align*} \mu \bigg( \bigg\{ x \in X: \sum_{i=0}^{\ell-1} I_{A} ( S^{i} x )> \gamma \bigg\} \bigg) > 1 - \epsilon \end{align*} $$
$$ \begin{align*} \mu \bigg( \bigg\{ x \in X: \sum_{i=0}^{\ell-1} I_{A} ( S^{i} x )> \gamma \bigg\} \bigg) > 1 - \epsilon \end{align*} $$
and
 $$ \begin{align*} \mu \bigg( \{ x \in X: \sum_{i=0}^{\ell-1} I_{B} ( S^{i} x )> \gamma \} \bigg) > 1 - \epsilon. \end{align*} $$
$$ \begin{align*} \mu \bigg( \{ x \in X: \sum_{i=0}^{\ell-1} I_{B} ( S^{i} x )> \gamma \} \bigg) > 1 - \epsilon. \end{align*} $$
Choose
![]() $h> \ell _0$
such that
$h> \ell _0$
such that
There is a Rohklin tower of height
![]() $4h$
with base I such that
$4h$
with base I such that
 $$ \begin{align*} \mu \bigg( \bigcup_{i=0}^{4h-1} S^i I \bigg)> 1 - \frac{\epsilon}{4h}. \end{align*} $$
$$ \begin{align*} \mu \bigg( \bigcup_{i=0}^{4h-1} S^i I \bigg)> 1 - \frac{\epsilon}{4h}. \end{align*} $$
There exist disjoint sets
![]() $I_1, I_2 \subset I$
such that for each
$I_1, I_2 \subset I$
such that for each
![]() $x \in I_1$
and
$x \in I_1$
and
![]() $y \in I_2$
, there exist
$y \in I_2$
, there exist
![]() $j(x), j(y)$
such that
$j(x), j(y)$
such that
![]() $h \leq j(x) < 2h$
,
$h \leq j(x) < 2h$
,
![]() $h \leq j(y) < 2h$
, and
$h \leq j(y) < 2h$
, and
 $$ \begin{align*} \sum_{i=0}^{\ell_0 - 1} I_A (S^{i + j(x)} x)> \gamma \end{align*} $$
$$ \begin{align*} \sum_{i=0}^{\ell_0 - 1} I_A (S^{i + j(x)} x)> \gamma \end{align*} $$
and
 $$ \begin{align*} \sum_{i=0}^{\ell_0 - 1} I_B (S^{i + j(y)} y)> \gamma. \end{align*} $$
$$ \begin{align*} \sum_{i=0}^{\ell_0 - 1} I_B (S^{i + j(y)} y)> \gamma. \end{align*} $$
By the choice of
![]() $\epsilon < {1} / {20}$
, then
$\epsilon < {1} / {20}$
, then
![]() $I_1, I_2$
may be chosen such that
$I_1, I_2$
may be chosen such that
For each
![]() $x \in I_1$
, let
$x \in I_1$
, let
![]() $i_1(x), i_2(x), \ldots i_{\gamma }(x)$
, be increasing such that
$i_1(x), i_2(x), \ldots i_{\gamma }(x)$
, be increasing such that
and similarly, for each
![]() $y \in I_2$
, let
$y \in I_2$
, let
![]() $i_1(y), i_2(y), \ldots i_{\gamma }(y)$
, be such that
$i_1(y), i_2(y), \ldots i_{\gamma }(y)$
, be such that
and
![]() $h \leq i_j(x) < 2h - 1$
,
$h \leq i_j(x) < 2h - 1$
,
![]() $i_{\gamma }(x) < i_{1}(x) + \ell _0$
, and
$i_{\gamma }(x) < i_{1}(x) + \ell _0$
, and
![]() $h \leq i_j(y) < 2h - 1$
,
$h \leq i_j(y) < 2h - 1$
,
![]() $i_{\gamma }(y) < i_{1}(y) + \ell _0$
. Let
$i_{\gamma }(y) < i_{1}(y) + \ell _0$
. Let
![]() $\phi : I_1 \to I_2$
be an invertible measure-preserving map. The transformation T will be defined in the following manner: for
$\phi : I_1 \to I_2$
be an invertible measure-preserving map. The transformation T will be defined in the following manner: for
![]() $x \in I_1$
, let
$x \in I_1$
, let
![]() $y = \phi (x) \in I_2$
,
$y = \phi (x) \in I_2$
,
and
Otherwise, define T to be identical to S everywhere else on X. Consider
 $$ \begin{align*} \sum_{i=0}^{3h-1} f ( T^i x ) \end{align*} $$
$$ \begin{align*} \sum_{i=0}^{3h-1} f ( T^i x ) \end{align*} $$
for
![]() $x \in \bigcup _{i=0}^{h - 1} T^i (I_1 \cup I_2)$
. Note for such x,
$x \in \bigcup _{i=0}^{h - 1} T^i (I_1 \cup I_2)$
. Note for such x,
 $$ \begin{align*} \bigg| \sum_{i=0}^{3h-1} f ( T^i x ) - \sum_{i=0}^{3h-1} f ( S^i x ) \bigg|> 2n. \end{align*} $$
$$ \begin{align*} \bigg| \sum_{i=0}^{3h-1} f ( T^i x ) - \sum_{i=0}^{3h-1} f ( S^i x ) \bigg|> 2n. \end{align*} $$
Since
 $$ \begin{align*} \mu \bigg( \bigcup_{i=0}^{h - 1} T^i (I_1 \cup I_2) \bigg)> \frac{1}{5} , \end{align*} $$
$$ \begin{align*} \mu \bigg( \bigcup_{i=0}^{h - 1} T^i (I_1 \cup I_2) \bigg)> \frac{1}{5} , \end{align*} $$
then
 $$ \begin{align*} \mu \bigg( \bigg\{ x \in X : \bigg| \sum_{i=0}^{3h-1} f ( T^i x ) \bigg|> n \bigg\} \bigg) > \eta. \end{align*} $$
$$ \begin{align*} \mu \bigg( \bigg\{ x \in X : \bigg| \sum_{i=0}^{3h-1} f ( T^i x ) \bigg|> n \bigg\} \bigg) > \eta. \end{align*} $$
This implies
![]() $T \in D_n$
and
$T \in D_n$
and
![]() $\| T - S \| < \epsilon $
. Thus,
$\| T - S \| < \epsilon $
. Thus,
![]() $\mathcal {T} = \bigcap _{n=1}^{\infty } D_n$
is a dense
$\mathcal {T} = \bigcap _{n=1}^{\infty } D_n$
is a dense
![]() $G_{\delta }$
set. If
$G_{\delta }$
set. If
![]() $T \in \mathcal {T}$
, then the Halász–Schmidt condition (2.1) for a measurable transfer function does not hold, and our result follows.
$T \in \mathcal {T}$
, then the Halász–Schmidt condition (2.1) for a measurable transfer function does not hold, and our result follows.
Acknowledgments
We thank Cesar Silva for feedback on this paper, and in particular for pointing out applications of coboundaries to invariant
![]() $\sigma $
-finite measures of non-singular transformations. Also, we thank El Houcein El Abdalaoui and Matthijs Borst for providing feedback on a previous version. Finally, we thank the referees for providing changes which improved the exposition of this paper.
$\sigma $
-finite measures of non-singular transformations. Also, we thank El Houcein El Abdalaoui and Matthijs Borst for providing feedback on a previous version. Finally, we thank the referees for providing changes which improved the exposition of this paper.
A Appendix. Coboundary existence for bounded measurable functions
In this section, we prove Proposition 3.6 which is our main tool used in §3. Also, we prove Lemma A.2 which is used in §3 as well.
A.1 Balanced partitions
Let A be a measurable subset of X and
![]() in
in
![]() $L_1(A,\mu _A)$
. Let
$L_1(A,\mu _A)$
. Let
![]() $\epsilon> 0$
. We say a finite partition
$\epsilon> 0$
. We say a finite partition
![]() $\Pi $
of A is
$\Pi $
of A is
![]() $\epsilon $
-balanced and uniform, if there exists
$\epsilon $
-balanced and uniform, if there exists
![]() $E\in \Pi $
such that:
$E\in \Pi $
such that:
-
(1)
 $\mu (E) < \epsilon \mu (A)$
;
$\mu (E) < \epsilon \mu (A)$
; -
(2)
 $\int _{A\setminus E} f\,d\mu = {\mu (A\setminus E)}/{\mu (A)} \int _A f\,d\mu $
;
$\int _{A\setminus E} f\,d\mu = {\mu (A\setminus E)}/{\mu (A)} \int _A f\,d\mu $
; -
(3)
 $| f(x) - f(y) | < \epsilon $
for
$| f(x) - f(y) | < \epsilon $
for
 $x,y\in a$
and
$x,y\in a$
and
 $a\in \Pi \setminus \{ E\}$
;
$a\in \Pi \setminus \{ E\}$
; -
(4)
 $\mu (c) = \mu (d)$
for
$\mu (c) = \mu (d)$
for
 $c,d \in \Pi \setminus \{ E\}$
.
$c,d \in \Pi \setminus \{ E\}$
.
We refer to this type of partition as a PUB(
![]() $\epsilon $
) partition for
$\epsilon $
) partition for
![]() $f_{|A}$
. The set E is referred to as the exceptional set of the PUB.
$f_{|A}$
. The set E is referred to as the exceptional set of the PUB.
Lemma A.1. Suppose
![]() $A\subset X$
is measurable and
$A\subset X$
is measurable and
![]() is integrable with mean zero and takes on essentially infinitely many values. Given
is integrable with mean zero and takes on essentially infinitely many values. Given
![]() $\epsilon>0$
, there exists a PUB(
$\epsilon>0$
, there exists a PUB(
![]() $\epsilon $
) partition such that f takes on essentially infinitely many values on both its exceptional set E and its complement
$\epsilon $
) partition such that f takes on essentially infinitely many values on both its exceptional set E and its complement
![]() $A\setminus E$
.
$A\setminus E$
.
Proof. Without loss of generality, it is sufficient to prove the lemma where
![]() $0 < \| f \|_{\infty } < 1$
and
$0 < \| f \|_{\infty } < 1$
and
![]() $\epsilon < 1$
. Let
$\epsilon < 1$
. Let
![]() . Choose
. Choose
![]() such that
such that
For
![]() $i = 0,1,2,\ldots , 2m - 1$
, let
$i = 0,1,2,\ldots , 2m - 1$
, let
 $$ \begin{align} A_i = \bigg\{ x\in A: -1 + \frac{i}{m} \leq f(x) < -1 + \frac{i+1}{m} \bigg\}. \end{align} $$
$$ \begin{align} A_i = \bigg\{ x\in A: -1 + \frac{i}{m} \leq f(x) < -1 + \frac{i+1}{m} \bigg\}. \end{align} $$
Let
![]() $\alpha = \min { \{ \mu (A_i) : \mu (A_i)> 0 \} }$
. There exists
$\alpha = \min { \{ \mu (A_i) : \mu (A_i)> 0 \} }$
. There exists
![]() $i_0$
such that f takes on infinitely many values on
$i_0$
such that f takes on infinitely many values on
![]() $A_{i_0}$
. By the definition of
$A_{i_0}$
. By the definition of
![]() $A_{i_0}$
, there exist disjoint subsets
$A_{i_0}$
, there exist disjoint subsets
![]() $E_0$
and
$E_0$
and
![]() $E_1$
of
$E_1$
of
![]() $A_{i_0}$
with equal measure and such that
$A_{i_0}$
with equal measure and such that
 $$ \begin{align} \frac{1}{\mu (E_0)} \int_{E_0} f\,d\mu < \frac{1}{\mu (A_{i_0})} \int_{A_{i_0}} f\,d\mu , \end{align} $$
$$ \begin{align} \frac{1}{\mu (E_0)} \int_{E_0} f\,d\mu < \frac{1}{\mu (A_{i_0})} \int_{A_{i_0}} f\,d\mu , \end{align} $$
 $$ \begin{align} \frac{1}{\mu (E_1)} \int_{E_1} f\,d\mu> \frac{1}{\mu (A_{i_0})} \int_{A_{i_0}} f\,d\mu , \end{align} $$
$$ \begin{align} \frac{1}{\mu (E_1)} \int_{E_1} f\,d\mu> \frac{1}{\mu (A_{i_0})} \int_{A_{i_0}} f\,d\mu , \end{align} $$
and f takes on infinitely many values on the set
![]() $A_{i_0} \setminus (E_0 \cup E_1)$
and on the set
$A_{i_0} \setminus (E_0 \cup E_1)$
and on the set
![]() $E_0 \cup E_1$
. Let
$E_0 \cup E_1$
. Let
 $$ \begin{align*}d = \min{\bigg\{ \bigg| \frac{1}{\mu (E_i)} \int_{E_i} f\,d\mu - \frac{1}{\mu (A_{i_0})} \int_{A_{i_0}} f\, d\mu \bigg| : i = 0,1 \bigg\}}.\end{align*} $$
$$ \begin{align*}d = \min{\bigg\{ \bigg| \frac{1}{\mu (E_i)} \int_{E_i} f\,d\mu - \frac{1}{\mu (A_{i_0})} \int_{A_{i_0}} f\, d\mu \bigg| : i = 0,1 \bigg\}}.\end{align*} $$
By simultaneous Diophantine approximation [Reference Cassels9, p. 14, Theorem VII], there exist
![]() and
and
![]() such that
such that
 $$ \begin{align} q> \max{\!\bigg\{\frac{2N}{(1 - \epsilon)\mu (A)}, \frac{2\mu (A)}{\,d\mu (E_1)} \bigg\}}, \end{align} $$
$$ \begin{align} q> \max{\!\bigg\{\frac{2N}{(1 - \epsilon)\mu (A)}, \frac{2\mu (A)}{\,d\mu (E_1)} \bigg\}}, \end{align} $$
and for
![]() $i=0, 1, \ldots , 2m - 1$
,
$i=0, 1, \ldots , 2m - 1$
,
 $$ \begin{align} 2mq^{{-1}/{2m}} & < d \bigg( \frac{2\alpha}{3} - q^{{-1}/{2m}} \bigg). \end{align} $$
$$ \begin{align} 2mq^{{-1}/{2m}} & < d \bigg( \frac{2\alpha}{3} - q^{{-1}/{2m}} \bigg). \end{align} $$
Let
![]() $n = q + 1$
. Thus,
$n = q + 1$
. Thus,
 $$ \begin{align} \bigg|\mu (A_i) - \bigg(\frac{p_i}{n} + \frac{\mu (A_i)}{n}\bigg)\bigg| < n^{-1}q^{{-1}/{2m}}. \end{align} $$
$$ \begin{align} \bigg|\mu (A_i) - \bigg(\frac{p_i}{n} + \frac{\mu (A_i)}{n}\bigg)\bigg| < n^{-1}q^{{-1}/{2m}}. \end{align} $$
For
![]() $i=0, 1, \ldots , 2m - 1$
, we can choose subsets
$i=0, 1, \ldots , 2m - 1$
, we can choose subsets
![]() $B_i \subset A_i$
such that
$B_i \subset A_i$
such that
 $$ \begin{align} \frac{1}{\mu (B_i)} \int_{B_i} f\,d\mu & = \frac{1}{\mu (A_i)} \int_{A_i} f\,d\mu. \end{align} $$
$$ \begin{align} \frac{1}{\mu (B_i)} \int_{B_i} f\,d\mu & = \frac{1}{\mu (A_i)} \int_{A_i} f\,d\mu. \end{align} $$
Thus,
 $$ \begin{align} \bigg| \sum\limits_{i=0}^{2m-1} \int_{B_i} f\,d\mu \bigg| & = \bigg| \sum\limits_{i=0}^{2m-1} \frac{\mu (B_i)}{\mu (A_i)} \int_{A_i} f\, d\mu \bigg| = \bigg| \sum\limits_{i=0}^{2m-1} \bigg(\frac{\mu (B_i)}{\mu (A_i)} - \frac{1}{n}\bigg) \int_{A_i} f\, d\mu \bigg|\end{align} $$
$$ \begin{align} \bigg| \sum\limits_{i=0}^{2m-1} \int_{B_i} f\,d\mu \bigg| & = \bigg| \sum\limits_{i=0}^{2m-1} \frac{\mu (B_i)}{\mu (A_i)} \int_{A_i} f\, d\mu \bigg| = \bigg| \sum\limits_{i=0}^{2m-1} \bigg(\frac{\mu (B_i)}{\mu (A_i)} - \frac{1}{n}\bigg) \int_{A_i} f\, d\mu \bigg|\end{align} $$
 $$ \begin{align} & \leq \sum\limits_{i=0}^{2m-1} \bigg| \mu (B_i) - \frac{\mu (A_i)}{n} \bigg| = \sum\limits_{i=0}^{2m-1} \bigg| \mu (A_i) - \frac{p_i + \mu (A_i)}{n} \bigg|\end{align} $$
$$ \begin{align} & \leq \sum\limits_{i=0}^{2m-1} \bigg| \mu (B_i) - \frac{\mu (A_i)}{n} \bigg| = \sum\limits_{i=0}^{2m-1} \bigg| \mu (A_i) - \frac{p_i + \mu (A_i)}{n} \bigg|\end{align} $$
 $$ \begin{align} & < 2m n^{-1} q^{{-1} / {2m}} < \frac{d}{n} \bigg(\frac{2\alpha}{3} - q^{{-1}/{2m}} \bigg). \end{align} $$
$$ \begin{align} & < 2m n^{-1} q^{{-1} / {2m}} < \frac{d}{n} \bigg(\frac{2\alpha}{3} - q^{{-1}/{2m}} \bigg). \end{align} $$
This implies we can choose
![]() $B_{i}$
such that
$B_{i}$
such that
 $$ \begin{align} \sum\limits_{i=0}^{2m-1} \int_{B_i} f\,d\mu = 0. \end{align} $$
$$ \begin{align} \sum\limits_{i=0}^{2m-1} \int_{B_i} f\,d\mu = 0. \end{align} $$
Let
![]() $E = \bigcup _{i=0}^{2m-1} B_i$
and partition each set
$E = \bigcup _{i=0}^{2m-1} B_i$
and partition each set
![]() $A_i\setminus B_i$
into
$A_i\setminus B_i$
into
![]() $p_i$
subsets of measure
$p_i$
subsets of measure
![]() ${1}/{n}$
to form
${1}/{n}$
to form
![]() $\Pi $
. Therefore,
$\Pi $
. Therefore,
![]() $\mu (E) < \epsilon \mu (A)$
and our lemma is proven.
$\mu (E) < \epsilon \mu (A)$
and our lemma is proven.
A.2 Balanced uniform towers
Let A be a measurable subset of X and
![]() a bounded, mean-zero function. Given finite measurable partition Q,
a bounded, mean-zero function. Given finite measurable partition Q,
![]() and
and
![]() $\epsilon> 0$
, an
$\epsilon> 0$
, an
![]() $\epsilon $
-balanced and uniform tower for f is a set of disjoint measurable sets
$\epsilon $
-balanced and uniform tower for f is a set of disjoint measurable sets
![]() $I_i\subset A$
for
$I_i\subset A$
for
![]() $i=1,2,\ldots ,h$
and an invertible measure-preserving map
$i=1,2,\ldots ,h$
and an invertible measure-preserving map
![]() $T: I_i \to I_{i+1}$
for
$T: I_i \to I_{i+1}$
for
![]() $i=1,2,\ldots , h-1$
, such that:
$i=1,2,\ldots , h-1$
, such that:
 $$ \begin{align} \mu \bigg(\bigcup_{i=1}^h I_i\bigg) > \mu (A) - \epsilon , \end{align} $$
$$ \begin{align} \mu \bigg(\bigcup_{i=1}^h I_i\bigg) > \mu (A) - \epsilon , \end{align} $$
 $$ \begin{align} \bigg|\sum\limits_{i=0}^{k} f(T^i x) \bigg| < \| f \|_{\infty} + \epsilon \quad\textrm{for } x\in I_1, k < h , \end{align} $$
$$ \begin{align} \bigg|\sum\limits_{i=0}^{k} f(T^i x) \bigg| < \| f \|_{\infty} + \epsilon \quad\textrm{for } x\in I_1, k < h , \end{align} $$
 $$ \begin{align} \sum_{i=1}^{h} \int_{I_i} f\,d\mu = \int_{A} f\,d\mu \ = \ 0, \end{align} $$
$$ \begin{align} \sum_{i=1}^{h} \int_{I_i} f\,d\mu = \int_{A} f\,d\mu \ = \ 0, \end{align} $$
 $$ \begin{align} \bigg| \sum\limits_{i=0}^{h-1} f(T^i x) \bigg| < \epsilon \quad \textrm{for } x\in I_1 , \end{align} $$
$$ \begin{align} \bigg| \sum\limits_{i=0}^{h-1} f(T^i x) \bigg| < \epsilon \quad \textrm{for } x\in I_1 , \end{align} $$
 $$ \begin{align} \textrm{for each } q\in Q, \text{there exists } \mathcal{I} \subset \{ 1,\ldots ,h \} \textrm{ such that } \mu \bigg( q \triangle \bigg( \bigcup_{i \in \mathcal{I}} I_i \bigg) \bigg) < \epsilon. \end{align} $$
$$ \begin{align} \textrm{for each } q\in Q, \text{there exists } \mathcal{I} \subset \{ 1,\ldots ,h \} \textrm{ such that } \mu \bigg( q \triangle \bigg( \bigcup_{i \in \mathcal{I}} I_i \bigg) \bigg) < \epsilon. \end{align} $$
We refer to this type of tower as a TUB
![]() $(\epsilon , h, Q)$
tower for
$(\epsilon , h, Q)$
tower for
![]() $f_{|A}$
.
$f_{|A}$
.
Lemma A.2. Let
![]() $( X, {\mathcal B} , \mu )$
be a standard probability space and A a measurable subset of X. Suppose
$( X, {\mathcal B} , \mu )$
be a standard probability space and A a measurable subset of X. Suppose
![]() ,
,
![]() $f \in L^{\infty }_0$
, takes on essentially infinitely many values. Given
$f \in L^{\infty }_0$
, takes on essentially infinitely many values. Given
![]() ,
,
![]() $\epsilon> 0$
and finite measurable partition Q, there exists
$\epsilon> 0$
and finite measurable partition Q, there exists
![]() $h> N$
such that f has a TUB
$h> N$
such that f has a TUB
![]() $(\epsilon , h, Q)$
tower.
$(\epsilon , h, Q)$
tower.
Proof. From the construction of PUB(
![]() ${\epsilon } / {3}$
) in the previous lemma, partition
${\epsilon } / {3}$
) in the previous lemma, partition
![]() $A_i \setminus B_i$
into a disjoint union of sets
$A_i \setminus B_i$
into a disjoint union of sets
![]() $A_i(j)$
for
$A_i(j)$
for
![]() $j=1,2,\ldots ,p_i$
, such that
$j=1,2,\ldots ,p_i$
, such that
A.2.1 Greedy stacking
Now we give an inductive procedure for stacking the sets
![]() $A_i(j)$
. Choose arbitrary
$A_i(j)$
. Choose arbitrary
![]() $A_i(j)$
and label the set
$A_i(j)$
and label the set
![]() $I_1$
. Given
$I_1$
. Given
![]() $I_1, I_2, \ldots , I_{k-1}$
, let
$I_1, I_2, \ldots , I_{k-1}$
, let
 $$ \begin{align} \sigma_{k-1} = \sum\limits_{i=1}^{k-1} \int_{I_i} f\,d\mu. \end{align} $$
$$ \begin{align} \sigma_{k-1} = \sum\limits_{i=1}^{k-1} \int_{I_i} f\,d\mu. \end{align} $$
If
![]() $k = h$
, then we are done. If
$k = h$
, then we are done. If
![]() $\sigma _{k-1} \leq 0$
, choose
$\sigma _{k-1} \leq 0$
, choose
 $$ \begin{align*} I_k=A_i(j) \not\subset \bigcup_{i=1}^{k-1} I_i \end{align*} $$
$$ \begin{align*} I_k=A_i(j) \not\subset \bigcup_{i=1}^{k-1} I_i \end{align*} $$
such that
![]() $\int _{I_k} f\,d\mu \geq 0$
. This is possible, since
$\int _{I_k} f\,d\mu \geq 0$
. This is possible, since
![]() $k < h $
and
$k < h $
and
![]() $\sigma _h = \sum \nolimits _{i=0}^{2m-1}\int _{A_i\setminus B_i} f\,d\mu = 0$
. Otherwise, if
$\sigma _h = \sum \nolimits _{i=0}^{2m-1}\int _{A_i\setminus B_i} f\,d\mu = 0$
. Otherwise, if
![]() $\sigma _k> 0$
, then by the construction of
$\sigma _k> 0$
, then by the construction of
![]() $A_i(j)$
, there exists
$A_i(j)$
, there exists
![]() $I_k \not \subset \bigcup _{i=1}^{k-1} I_i$
such that
$I_k \not \subset \bigcup _{i=1}^{k-1} I_i$
such that
![]() $\int _{I_k} f\,d\mu < 0$
. This procedure produces a sequence of sets
$\int _{I_k} f\,d\mu < 0$
. This procedure produces a sequence of sets
![]() $I_i$
for
$I_i$
for
![]() $i=1, 2, \ldots , h$
with the property:
$i=1, 2, \ldots , h$
with the property:
 $$ \begin{align} \sum\limits_{i=1}^{h} \int_{I_i} f\,d\mu & = \sum\limits_{i=0}^{2m-1} \int_{A_i\setminus B_i} f\,d\mu \end{align} $$
$$ \begin{align} \sum\limits_{i=1}^{h} \int_{I_i} f\,d\mu & = \sum\limits_{i=0}^{2m-1} \int_{A_i\setminus B_i} f\,d\mu \end{align} $$
 $$ \begin{align} & = \sum\limits_{i=0}^{2m-1} \int_{B_i} f\,d\mu = 0.\end{align} $$
$$ \begin{align} & = \sum\limits_{i=0}^{2m-1} \int_{B_i} f\,d\mu = 0.\end{align} $$
A.2.2 Level refinement
Our transformation
![]() $\tau $
will map
$\tau $
will map
![]() $I_i$
onto
$I_i$
onto
![]() $I_{i+1}$
for
$I_{i+1}$
for
![]() $i = 1, 2, \ldots , h-1$
. Choose k such that
$i = 1, 2, \ldots , h-1$
. Choose k such that
![]() $k> {3h} / {\epsilon }$
. Partition the range such that for
$k> {3h} / {\epsilon }$
. Partition the range such that for
![]() $\ell =-k, -k + 1, \ldots , -1, 0, 1, \ldots , k - 1$
,
$\ell =-k, -k + 1, \ldots , -1, 0, 1, \ldots , k - 1$
,
 $$ \begin{align*} B_{i, \ell} = \bigg\{ x \in I_i : \frac{\ell}{k} \leq f(x) < \frac{\ell+1}{k} \bigg\}. \end{align*} $$
$$ \begin{align*} B_{i, \ell} = \bigg\{ x \in I_i : \frac{\ell}{k} \leq f(x) < \frac{\ell+1}{k} \bigg\}. \end{align*} $$
Via a measure-space isomorphism, we can take
![]() $X = [0,1]$
(that is, an ordered set). Let
$X = [0,1]$
(that is, an ordered set). Let
![]() $\tau _0$
be any invertible measure-preserving map such that
$\tau _0$
be any invertible measure-preserving map such that
![]() $\tau _0 : I_i \to I_{i+1}$
for
$\tau _0 : I_i \to I_{i+1}$
for
![]() $i \in \{ 1, 2, \ldots , h-1 \}$
. Define the quantized function
$i \in \{ 1, 2, \ldots , h-1 \}$
. Define the quantized function
![]() $f_i ( x ) = { \ell } / { k }$
if
$f_i ( x ) = { \ell } / { k }$
if
![]() $x \in B_{ i, \ell }$
. Define an invertible measure-preserving map
$x \in B_{ i, \ell }$
. Define an invertible measure-preserving map
![]() $\psi _i : I_i \to I_i$
such that
$\psi _i : I_i \to I_i$
such that
![]() $f_i \circ \psi _i$
is non-decreasing for
$f_i \circ \psi _i$
is non-decreasing for
![]() $i \geq 2$
. Let
$i \geq 2$
. Let
![]() $\psi _1$
be the identity map on
$\psi _1$
be the identity map on
![]() $I_1$
. Define an invertible measure-preserving map
$I_1$
. Define an invertible measure-preserving map
![]() $\phi _1 : I_1 \to I_1$
such that
$\phi _1 : I_1 \to I_1$
such that
![]() $f_1 \circ \phi _1$
is non-increasing. The map
$f_1 \circ \phi _1$
is non-increasing. The map
![]() $g_2 = f_1 \circ \phi _1 + f_2 \circ \psi _2 \circ \tau _0$
is a step function on
$g_2 = f_1 \circ \phi _1 + f_2 \circ \psi _2 \circ \tau _0$
is a step function on
![]() $I_1$
. Thus, there exists
$I_1$
. Thus, there exists
![]() $\phi _2 : I_1 \to I_1$
such that
$\phi _2 : I_1 \to I_1$
such that
![]() $g_2 \circ \phi _2$
is non-increasing. Let
$g_2 \circ \phi _2$
is non-increasing. Let
![]() $g_3 = g_2 \circ \phi _2 + f_3 \circ \psi _3 \circ \tau _0^2$
. Continue this process until we have defined
$g_3 = g_2 \circ \phi _2 + f_3 \circ \psi _3 \circ \tau _0^2$
. Continue this process until we have defined
![]() $g_h$
. In particular, by induction,
$g_h$
. In particular, by induction,
![]() $g_{h-1}$
will be a step function on
$g_{h-1}$
will be a step function on
![]() $I_1$
. Thus, we can define
$I_1$
. Thus, we can define
![]() $\phi _{h-1}: I_1 \to I_1$
such that
$\phi _{h-1}: I_1 \to I_1$
such that
![]() $g_{h-1} \circ \phi _{h-1}$
is non-increasing. Let
$g_{h-1} \circ \phi _{h-1}$
is non-increasing. Let
![]() $g_h = g_{h-1} \circ \phi _{h-1} + f_h \circ \psi _h \circ \tau _0^{h-1}$
. A formula for
$g_h = g_{h-1} \circ \phi _{h-1} + f_h \circ \psi _h \circ \tau _0^{h-1}$
. A formula for
![]() $g_h$
is
$g_h$
is
 $$ \begin{align*} g_h = \sum_{\ell =1}^{h} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{j=\ell}^{h-1} \phi_j. \end{align*} $$
$$ \begin{align*} g_h = \sum_{\ell =1}^{h} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{j=\ell}^{h-1} \phi_j. \end{align*} $$
For
![]() $2 \leq \ell \leq h$
, define
$2 \leq \ell \leq h$
, define
Let
![]() $\tau _1$
be the identity map. Each
$\tau _1$
be the identity map. Each
![]() $\tau _{\ell }$
is an invertible measure-preserving mapping from
$\tau _{\ell }$
is an invertible measure-preserving mapping from
![]() $I_1 \to I_{\ell }$
. Define the final mapping
$I_1 \to I_{\ell }$
. Define the final mapping
![]() $\tau $
as
$\tau $
as
![]() $\tau ( x ) = \tau _{\ell +1} \circ \tau _{\ell }^{-1} (x)$
for
$\tau ( x ) = \tau _{\ell +1} \circ \tau _{\ell }^{-1} (x)$
for
![]() $x \in I_{\ell }$
. Because of the greedy algorithm of sorting at each stage and re-ordering so that the next level has
$x \in I_{\ell }$
. Because of the greedy algorithm of sorting at each stage and re-ordering so that the next level has
![]() $f_{\ell }$
monotonic in the opposite direction, then the quantized functions
$f_{\ell }$
monotonic in the opposite direction, then the quantized functions
![]() $f_{\ell }$
do not exhibit much variation as points are iterated through the TUB under
$f_{\ell }$
do not exhibit much variation as points are iterated through the TUB under
![]() $\tau $
.
$\tau $
.
Claim A.3. For
![]() ,
,
![]() $m < h$
and a.e.
$m < h$
and a.e.
![]() $x, y \in I_1$
,
$x, y \in I_1$
,
 $$ \begin{align*} \bigg| \sum_{\ell =1}^{m} f_{\ell} ( \tau^{\ell -1} x ) - \sum_{\ell =1}^{m} f_{\ell} ( \tau^{\ell -1} y ) \bigg| < \frac{\epsilon}{3}, \end{align*} $$
$$ \begin{align*} \bigg| \sum_{\ell =1}^{m} f_{\ell} ( \tau^{\ell -1} x ) - \sum_{\ell =1}^{m} f_{\ell} ( \tau^{\ell -1} y ) \bigg| < \frac{\epsilon}{3}, \end{align*} $$
that is, there does not exist disjoint subsets
![]() $D_1, D_2$
of
$D_1, D_2$
of
![]() $I_1$
with equal positive measure such that for
$I_1$
with equal positive measure such that for
![]() $x \in D_1$
and
$x \in D_1$
and
![]() $y\in D_2$
,
$y\in D_2$
,
 $$ \begin{align*} \bigg| \sum_{\ell =1}^{m} f_{\ell} ( \tau^{\ell -1} x ) - \sum_{\ell =1}^{m} f_{\ell} ( \tau^{\ell -1} y ) \bigg| \geq \frac{\epsilon}{3}. \end{align*} $$
$$ \begin{align*} \bigg| \sum_{\ell =1}^{m} f_{\ell} ( \tau^{\ell -1} x ) - \sum_{\ell =1}^{m} f_{\ell} ( \tau^{\ell -1} y ) \bigg| \geq \frac{\epsilon}{3}. \end{align*} $$
Proof. It is sufficient to prove that for
![]() ,
,
![]() $m < h$
and a.e.
$m < h$
and a.e.
![]() $x, y \in I_1$
,
$x, y \in I_1$
,
 $$ \begin{align*} \bigg( \sum_{\ell =1}^{m} f_{\ell} ( \tau^{\ell -1} x ) - \sum_{\ell =1}^{m} f_{\ell} ( \tau^{\ell -1} y ) \bigg) < \frac{\epsilon}{3}. \end{align*} $$
$$ \begin{align*} \bigg( \sum_{\ell =1}^{m} f_{\ell} ( \tau^{\ell -1} x ) - \sum_{\ell =1}^{m} f_{\ell} ( \tau^{\ell -1} y ) \bigg) < \frac{\epsilon}{3}. \end{align*} $$
By applying the invertible measure-preserving isomorphism,
![]() $\phi _{h-1}^{-1} \phi _{h-2}^{-1} \ldots \phi _{m}^{-1}$
, it is sufficient to prove for
$\phi _{h-1}^{-1} \phi _{h-2}^{-1} \ldots \phi _{m}^{-1}$
, it is sufficient to prove for
![]() ,
,
![]() $m < h$
and a.e.
$m < h$
and a.e.
![]() $x, y \in I_1$
,
$x, y \in I_1$
,
 $$ \begin{align*} \bigg( \sum_{\ell =1}^{m} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m-1} \phi_i (x) - \sum_{\ell =1}^{m} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m-1} \phi_i (y) \bigg) < \frac{\epsilon}{3}. \end{align*} $$
$$ \begin{align*} \bigg( \sum_{\ell =1}^{m} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m-1} \phi_i (x) - \sum_{\ell =1}^{m} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m-1} \phi_i (y) \bigg) < \frac{\epsilon}{3}. \end{align*} $$
We can prove the claim inductively on m. Clearly, it is true for
![]() $m=1$
(by applying the PUB condition on
$m=1$
(by applying the PUB condition on
![]() $I_1$
). Suppose it is true for
$I_1$
). Suppose it is true for
![]() $m < h$
. Let
$m < h$
. Let
![]() $x_0$
and
$x_0$
and
![]() $y_0$
be distinct points in
$y_0$
be distinct points in
![]() $I_1$
. Let
$I_1$
. Let
![]() $x_1 = \phi _m(x_0)$
and
$x_1 = \phi _m(x_0)$
and
![]() $y_1 = \phi _m(y_0)$
. Consider first the case:
$y_1 = \phi _m(y_0)$
. Consider first the case:
 $$ \begin{align*} 0 < \sum_{\ell =1}^{m} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m-1} \phi_i (x_1) - \sum_{\ell =1}^{m} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m-1} \phi_i (y_1) < \frac{\epsilon}{3}. \end{align*} $$
$$ \begin{align*} 0 < \sum_{\ell =1}^{m} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m-1} \phi_i (x_1) - \sum_{\ell =1}^{m} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m-1} \phi_i (y_1) < \frac{\epsilon}{3}. \end{align*} $$
By the construction of
![]() $\phi _m$
,
$\phi _m$
,
![]() $x_0 < y_0$
. This is because the following function is non-increasing in x:
$x_0 < y_0$
. This is because the following function is non-increasing in x:
 $$ \begin{align*} \sum_{\ell =1}^{m} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m} \phi_i (x). \end{align*} $$
$$ \begin{align*} \sum_{\ell =1}^{m} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m} \phi_i (x). \end{align*} $$
Since the function
is non-decreasing in x, then
By combining terms,
 $$ \begin{align*} \sum_{\ell =1}^{m+1} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m} \phi_i (x_0) - \sum_{\ell =1}^{m+1} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m} \phi_i (y_0) < \frac{\epsilon}{3}. \end{align*} $$
$$ \begin{align*} \sum_{\ell =1}^{m+1} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m} \phi_i (x_0) - \sum_{\ell =1}^{m+1} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m} \phi_i (y_0) < \frac{\epsilon}{3}. \end{align*} $$
The case where
 $$ \begin{align*} 0 < \sum_{\ell =1}^{m} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m-1} \phi_i (y_1) - \sum_{\ell =1}^{m} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m-1} \phi_i (x_1) < \frac{\epsilon}{3} \end{align*} $$
$$ \begin{align*} 0 < \sum_{\ell =1}^{m} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m-1} \phi_i (y_1) - \sum_{\ell =1}^{m} f_{\ell} \psi_{\ell} \tau_0^{\ell -1} \Pi_{i=\ell}^{m-1} \phi_i (x_1) < \frac{\epsilon}{3} \end{align*} $$
may be handled in a similar fashion. This completes the proof of the claim.
Now we complete the proof of the lemma. The function f was quantized to
![]() $f_{\ell }$
in such a way that for
$f_{\ell }$
in such a way that for
![]() $x \in I_1$
and
$x \in I_1$
and
![]() $m \in \{ 1, 2, \ldots , h \}$
,
$m \in \{ 1, 2, \ldots , h \}$
,
 $$ \begin{align} \bigg| \sum_{\ell=1}^{m} f ( \tau^{\ell-1} x ) - \sum_{\ell=1}^{m} f_{\ell} ( \tau^{\ell-1} x ) \bigg| &\leq \sum_{\ell=1}^{m} | f ( \tau^{\ell-1} x ) - f_{\ell} ( \tau^{\ell-1} x ) |\end{align} $$
$$ \begin{align} \bigg| \sum_{\ell=1}^{m} f ( \tau^{\ell-1} x ) - \sum_{\ell=1}^{m} f_{\ell} ( \tau^{\ell-1} x ) \bigg| &\leq \sum_{\ell=1}^{m} | f ( \tau^{\ell-1} x ) - f_{\ell} ( \tau^{\ell-1} x ) |\end{align} $$
 $$ \begin{align} &< h \bigg( \frac{\epsilon}{3h} \bigg) = \frac{\epsilon}{3}.\end{align} $$
$$ \begin{align} &< h \bigg( \frac{\epsilon}{3h} \bigg) = \frac{\epsilon}{3}.\end{align} $$
Hence,
 $$ \begin{align} \bigg| \sum_{\ell=1}^{m} f ( \tau^{\ell-1} x ) - \sum_{\ell=1}^{m} f ( \tau^{\ell-1} y ) \bigg| & \leq \bigg| \sum_{\ell=1}^{m} f ( \tau^{\ell-1} x ) - \sum_{\ell=1}^{m} f_{\ell} ( \tau^{\ell-1} x ) \bigg|\end{align} $$
$$ \begin{align} \bigg| \sum_{\ell=1}^{m} f ( \tau^{\ell-1} x ) - \sum_{\ell=1}^{m} f ( \tau^{\ell-1} y ) \bigg| & \leq \bigg| \sum_{\ell=1}^{m} f ( \tau^{\ell-1} x ) - \sum_{\ell=1}^{m} f_{\ell} ( \tau^{\ell-1} x ) \bigg|\end{align} $$
 $$ \begin{align} & \quad+ \bigg| \sum_{\ell=1}^{m} f_{\ell} ( \tau^{\ell-1} x ) - \sum_{\ell=1}^{m} f_{\ell} ( \tau^{\ell-1} y ) \bigg|\end{align} $$
$$ \begin{align} & \quad+ \bigg| \sum_{\ell=1}^{m} f_{\ell} ( \tau^{\ell-1} x ) - \sum_{\ell=1}^{m} f_{\ell} ( \tau^{\ell-1} y ) \bigg|\end{align} $$
 $$ \begin{align} & \quad+ \bigg| \sum_{\ell=1}^{m} f_{\ell} ( \tau^{\ell-1} y ) - \sum_{\ell=1}^{m} f_{\ell} ( \tau^{\ell-1} y ) \bigg|\end{align} $$
$$ \begin{align} & \quad+ \bigg| \sum_{\ell=1}^{m} f_{\ell} ( \tau^{\ell-1} y ) - \sum_{\ell=1}^{m} f_{\ell} ( \tau^{\ell-1} y ) \bigg|\end{align} $$
Therefore, this proves (A.20) of our lemma. Claim (A.18) follows in a similar manner.
Proof of Proposition 3.6
If
![]() is a finite step function, a solution is given in [Reference Adams and Rosenblatt2]. The transfer function g is bounded, since, by [Reference Lin and Sine33],
is a finite step function, a solution is given in [Reference Adams and Rosenblatt2]. The transfer function g is bounded, since, by [Reference Lin and Sine33],
 $$ \begin{align*} g(x) = \lim_{n\to \infty} \frac{1}{n} \sum_{k=1}^{n} \sum_{i=0}^{k-1} f ( T^i x ) \leq \sum_{i=1}^{m} | a_i |. \end{align*} $$
$$ \begin{align*} g(x) = \lim_{n\to \infty} \frac{1}{n} \sum_{k=1}^{n} \sum_{i=0}^{k-1} f ( T^i x ) \leq \sum_{i=1}^{m} | a_i |. \end{align*} $$
Otherwise, f takes on essentially infinitely many values. Let
![]() $\delta _i> 0$
be such that
$\delta _i> 0$
be such that
![]() $\sum _{i=1}^{\infty } \delta _i < \infty $
and
$\sum _{i=1}^{\infty } \delta _i < \infty $
and
![]() $Q_i$
for
$Q_i$
for
![]() a refining sequence of partitions which generate the sigma algebra
a refining sequence of partitions which generate the sigma algebra
![]() $\mathcal {B}$
. Let
$\mathcal {B}$
. Let
![]() $\epsilon _1 = \delta _1$
. Use Lemma A.2 to construct a TUB
$\epsilon _1 = \delta _1$
. Use Lemma A.2 to construct a TUB
![]() $(\epsilon _1, h_1, Q_1)$
tower with decomposition into sets
$(\epsilon _1, h_1, Q_1)$
tower with decomposition into sets
![]() $A_1$
,
$A_1$
,
![]() $B_1$
, and measure-preserving map
$B_1$
, and measure-preserving map
![]() $T_1$
. Also, assume
$T_1$
. Also, assume
![]() $A_1$
is made of levels
$A_1$
is made of levels
![]() $I_{1,i}$
for
$I_{1,i}$
for
![]() $1\leq i \leq h_1$
. Let
$1\leq i \leq h_1$
. Let
![]() $S_1 = T_1$
. Define
$S_1 = T_1$
. Define
![]() by
by
 $$ \begin{align*} f_1(x)= \begin{cases} \displaystyle\sum_{i=0}^{h_1-1} f(S_1^i x) & \textrm{if}\ x\in I_{1,1}, \\ f(x) & \textrm{if}\ x\in B_1. \end{cases} \end{align*} $$
$$ \begin{align*} f_1(x)= \begin{cases} \displaystyle\sum_{i=0}^{h_1-1} f(S_1^i x) & \textrm{if}\ x\in I_{1,1}, \\ f(x) & \textrm{if}\ x\in B_1. \end{cases} \end{align*} $$
Let
![]() $\epsilon _2 = \delta _2 \mu ( I_{1,1} )$
. Since
$\epsilon _2 = \delta _2 \mu ( I_{1,1} )$
. Since
![]() $\int _{B_1 \cup I_{1,1}} f_1\,d\mu = 0$
, then we can apply Lemma A.2 to
$\int _{B_1 \cup I_{1,1}} f_1\,d\mu = 0$
, then we can apply Lemma A.2 to
![]() $f_1$
to obtain a TUB
$f_1$
to obtain a TUB
![]() $(\epsilon _2, h_2, Q_2)$
tower and decompose
$(\epsilon _2, h_2, Q_2)$
tower and decompose
![]() $B_1 \cup I_{1,1}$
into
$B_1 \cup I_{1,1}$
into
![]() $A_2 = \bigcup _{i=1}^{h_2} I_{2,i}$
and
$A_2 = \bigcup _{i=1}^{h_2} I_{2,i}$
and
![]() $B_2$
such that there exists measure-preserving
$B_2$
such that there exists measure-preserving
![]() $T_2: I_{2,i} \to I_{2,i+1}$
for
$T_2: I_{2,i} \to I_{2,i+1}$
for
![]() $i = 1, \ldots , h_2 - 1$
. Define
$i = 1, \ldots , h_2 - 1$
. Define
![]() $S_2$
as
$S_2$
as
 $$ \begin{align*} S_2(x)= \begin{cases} S_1 (x) & \textrm{if}\ x\in S_1^i I_{2,j} \subset S_1^i I_{1,1}, \textrm{for}\ 0\leq i \leq h_1-1\ \textrm{and}\ 1 \leq j \leq h_2, \\ T_2 ( S_1^{ 1 - h_1 } (x) ) & \textrm{if}\ x\in S_1^{h_1 - 1} I_{2,j} \subset S_1^{h_1 - 1} I_{1,1}, \textrm{for}\ 1 \leq j < h_2, \\ T_2(x) & \textrm{if}\ x\in B_1 \cap A_2 \setminus I_{2,h_2}. \end{cases} \end{align*} $$
$$ \begin{align*} S_2(x)= \begin{cases} S_1 (x) & \textrm{if}\ x\in S_1^i I_{2,j} \subset S_1^i I_{1,1}, \textrm{for}\ 0\leq i \leq h_1-1\ \textrm{and}\ 1 \leq j \leq h_2, \\ T_2 ( S_1^{ 1 - h_1 } (x) ) & \textrm{if}\ x\in S_1^{h_1 - 1} I_{2,j} \subset S_1^{h_1 - 1} I_{1,1}, \textrm{for}\ 1 \leq j < h_2, \\ T_2(x) & \textrm{if}\ x\in B_1 \cap A_2 \setminus I_{2,h_2}. \end{cases} \end{align*} $$
Suppose
![]() $T_n$
and
$T_n$
and
![]() $S_n$
have been defined. Proceed in a similar manner to define
$S_n$
have been defined. Proceed in a similar manner to define
![]() $S_{n+1}$
. In particular, for a.e.
$S_{n+1}$
. In particular, for a.e.
![]() $y \in X\setminus B_n$
, there exist a unique
$y \in X\setminus B_n$
, there exist a unique
![]() $x \in I_{n,1}$
and
$x \in I_{n,1}$
and
![]() $j_y \geq 0$
such that
$j_y \geq 0$
such that
![]() $y = S_n^{j_y} x$
. For a.e.
$y = S_n^{j_y} x$
. For a.e.
![]() $x \in I_{n,1}$
, there exists a minimum
$x \in I_{n,1}$
, there exists a minimum
![]() $k_{n,x} \geq 0$
such that
$k_{n,x} \geq 0$
such that
![]() $S_n^{k_{n,x}} x \in I_{n,h_n}$
. Define
$S_n^{k_{n,x}} x \in I_{n,h_n}$
. Define
![]() such that
such that
 $$ \begin{align*} f_n(x)= \begin{cases} \displaystyle\sum_{i=0}^{k_{n,x}} f(S_n^i x) & \textrm{if}\ x\in I_{n,1}, \\ f(x) & \textrm{if}\ x\in B_n. \end{cases} \end{align*} $$
$$ \begin{align*} f_n(x)= \begin{cases} \displaystyle\sum_{i=0}^{k_{n,x}} f(S_n^i x) & \textrm{if}\ x\in I_{n,1}, \\ f(x) & \textrm{if}\ x\in B_n. \end{cases} \end{align*} $$
Let
![]() $\epsilon _{n+1} = \delta _{n+1} \mu ( I_{n,1} )$
. Since
$\epsilon _{n+1} = \delta _{n+1} \mu ( I_{n,1} )$
. Since
![]() $\int _{B_n \cup I_{n,1}} f_n\,d\mu = 0$
, then we can apply Lemma A.2 to
$\int _{B_n \cup I_{n,1}} f_n\,d\mu = 0$
, then we can apply Lemma A.2 to
![]() $f_n$
to obtain a TUB
$f_n$
to obtain a TUB
![]() $(\epsilon _{n+1}, h_{n+1}, Q_{n+1})$
tower and decompose
$(\epsilon _{n+1}, h_{n+1}, Q_{n+1})$
tower and decompose
![]() $B_n \cup I_{n,1}$
into
$B_n \cup I_{n,1}$
into
![]() $A_{n+1} = \bigcup _{i=1}^{h_{n+1}} I_{{n+1},i}$
and
$A_{n+1} = \bigcup _{i=1}^{h_{n+1}} I_{{n+1},i}$
and
![]() $B_{n+1}$
such that there exists measure-preserving
$B_{n+1}$
such that there exists measure-preserving
![]() $T_{n+1}: I_{n+1,i} \to I_{n+1,i+1}$
for
$T_{n+1}: I_{n+1,i} \to I_{n+1,i+1}$
for
![]() $i = 1, \ldots , h_{n+1} - 1$
. Define
$i = 1, \ldots , h_{n+1} - 1$
. Define
![]() $S_{n+1}$
as
$S_{n+1}$
as
 $$ \begin{align*} S_{n+1}(x)= \begin{cases} S_n (x) & \textrm{if}\ x\in S_n^i I_{n+1,j} \subset S_n^i I_{n,1},\\ &\quad\textrm{ for}\ 0\leq i \leq h_n-1\ \textrm{and}\ 1 \leq j \leq h_{n+1}, \\ T_{n+1} ( S_n^{ 1 - h_n } (x) ) & \textrm{if}\ x\in S_n^{h_n - 1} I_{n+1,j} \subset S_n^{h_n - 1} I_{n,1}, \textrm{for}\ 1 \leq j < h_{n+1}, \\ T_{n+1}(x) & \textrm{if}\ x\in B_n \cap A_{n+1} \setminus I_{n+1,h_{n+1}}. \end{cases} \end{align*} $$
$$ \begin{align*} S_{n+1}(x)= \begin{cases} S_n (x) & \textrm{if}\ x\in S_n^i I_{n+1,j} \subset S_n^i I_{n,1},\\ &\quad\textrm{ for}\ 0\leq i \leq h_n-1\ \textrm{and}\ 1 \leq j \leq h_{n+1}, \\ T_{n+1} ( S_n^{ 1 - h_n } (x) ) & \textrm{if}\ x\in S_n^{h_n - 1} I_{n+1,j} \subset S_n^{h_n - 1} I_{n,1}, \textrm{for}\ 1 \leq j < h_{n+1}, \\ T_{n+1}(x) & \textrm{if}\ x\in B_n \cap A_{n+1} \setminus I_{n+1,h_{n+1}}. \end{cases} \end{align*} $$
Note that
![]() $S_{n+1} (x) = S_n (x)$
except for x in a set of measure less than or equal to
$S_{n+1} (x) = S_n (x)$
except for x in a set of measure less than or equal to
Since
![]() $\sum _{n=1}^{\infty } \beta _n < \infty $
, then
$\sum _{n=1}^{\infty } \beta _n < \infty $
, then
![]() $S(x) = \lim _{n\to \infty } S_n (x)$
exists almost everywhere. In particular,
$S(x) = \lim _{n\to \infty } S_n (x)$
exists almost everywhere. In particular,
![]() $S_n(x)$
is eventually constant for a.e.
$S_n(x)$
is eventually constant for a.e.
![]() $x \in X$
. Thus, since each
$x \in X$
. Thus, since each
![]() $S_n$
is invertible and measure preserving, then S is invertible and measure preserving. By a careful choice of
$S_n$
is invertible and measure preserving, then S is invertible and measure preserving. By a careful choice of
![]() $Q_i$
, S will be ergodic.
$Q_i$
, S will be ergodic.
B Appendix. Universal moving averages
Below we prove that if f is a coboundary with an
![]() $L^1$
-transfer function g, then all moving averages converge pointwise.
$L^1$
-transfer function g, then all moving averages converge pointwise.
Theorem B.1. Suppose T is an ergodic invertible measure-preserving transformation on
![]() $(X, {\mathcal B}, \mu )$
. If f is a coboundary with integrable transfer function g, then for all strictly increasing
$(X, {\mathcal B}, \mu )$
. If f is a coboundary with integrable transfer function g, then for all strictly increasing
![]() ,
,
![]() $v_n \in \mathbb {Z}$
, and a.e.
$v_n \in \mathbb {Z}$
, and a.e.
![]() $x \in X$
,
$x \in X$
,
 $$ \begin{align*} \lim_{n\to \infty} \frac{1}{L_n} \sum_{i=1}^{L_n} f(T^{v_n + i} x) = 0. \end{align*} $$
$$ \begin{align*} \lim_{n\to \infty} \frac{1}{L_n} \sum_{i=1}^{L_n} f(T^{v_n + i} x) = 0. \end{align*} $$
Proof. Suppose
![]() $f(x) = g(x) - g(Tx)$
for a.e.
$f(x) = g(x) - g(Tx)$
for a.e.
![]() $x\in X$
. Then
$x\in X$
. Then
 $$ \begin{align*} \sum_{i=1}^{L_n} f(T^{v_n + i} x) = g(T^{v_n+1}x) - g(T^{v_n + L_n + 1}x). \end{align*} $$
$$ \begin{align*} \sum_{i=1}^{L_n} f(T^{v_n + i} x) = g(T^{v_n+1}x) - g(T^{v_n + L_n + 1}x). \end{align*} $$
Since
![]() $L_n \geq n$
is strictly increasing, it is sufficient to show each of the following:
$L_n \geq n$
is strictly increasing, it is sufficient to show each of the following:
-
• for a.e. x,
 $\lim _{n\to \infty } {g(T^{v_n+1}x)}/{n} = 0$
;
$\lim _{n\to \infty } {g(T^{v_n+1}x)}/{n} = 0$
; -
• for a.e. x,
 $\lim _{n\to \infty } {g(T^{v_n+L_n+1}x)}/{n} = 0$
.
$\lim _{n\to \infty } {g(T^{v_n+L_n+1}x)}/{n} = 0$
.
Here, we show the first term converges to zero. A similar argument will show the second term converges.
For
![]() , define
, define
Since
![]() $g \in L^1(\mu )$
, then
$g \in L^1(\mu )$
, then
 $$ \begin{align*} \sum_{n=1}^{\infty} (n-1) \mu (E_{n,1}) \leq \int_X | g(T^{v_1+1}x) |\,d\mu < \infty. \end{align*} $$
$$ \begin{align*} \sum_{n=1}^{\infty} (n-1) \mu (E_{n,1}) \leq \int_X | g(T^{v_1+1}x) |\,d\mu < \infty. \end{align*} $$
There exists non-decreasing
![]() such that
such that
![]() $\lim _{n\to \infty } {n}/{K_n} = 0$
and
$\lim _{n\to \infty } {n}/{K_n} = 0$
and
 $$ \begin{align*} \sum_{n=1}^{\infty} K_n \mu (E_{n,1}) < \infty. \end{align*} $$
$$ \begin{align*} \sum_{n=1}^{\infty} K_n \mu (E_{n,1}) < \infty. \end{align*} $$
Define
 $$ \begin{align*} E = \bigcap_{m=1}^{\infty} \bigcup_{n=m}^{\infty} \bigcup_{k=1}^{K_n} E_{n,k}. \end{align*} $$
$$ \begin{align*} E = \bigcap_{m=1}^{\infty} \bigcup_{n=m}^{\infty} \bigcup_{k=1}^{K_n} E_{n,k}. \end{align*} $$
First, since T is measure preserving, we have
 $$ \begin{align*} \mu(E) \leq \sum_{n=m}^{\infty} \sum_{k=1}^{K_n} \mu(E_{n,k}) &= \sum_{n=m}^{\infty} \sum_{k=1}^{K_n} \mu(E_{n,1}) \\ &= \sum_{n=m}^{\infty} K_n \mu(E_{n,1}) \to 0\quad\textrm{as } m\to \infty. \end{align*} $$
$$ \begin{align*} \mu(E) \leq \sum_{n=m}^{\infty} \sum_{k=1}^{K_n} \mu(E_{n,k}) &= \sum_{n=m}^{\infty} \sum_{k=1}^{K_n} \mu(E_{n,1}) \\ &= \sum_{n=m}^{\infty} K_n \mu(E_{n,1}) \to 0\quad\textrm{as } m\to \infty. \end{align*} $$
Thus,
![]() $\mu (E)=0$
. Second, we show for
$\mu (E)=0$
. Second, we show for
![]() $x\notin E$
, we have almost everywhere convergence. If
$x\notin E$
, we have almost everywhere convergence. If
![]() $x\notin E$
, there exists m sufficiently large such that
$x\notin E$
, there exists m sufficiently large such that
 $$ \begin{align*} x \notin \bigcup_{k=1}^{K_n} E_{n,k} \quad\textrm{for } n\geq m. \end{align*} $$
$$ \begin{align*} x \notin \bigcup_{k=1}^{K_n} E_{n,k} \quad\textrm{for } n\geq m. \end{align*} $$
Hence, if
![]() $n> |g(T^{v_k+1}x)| \geq n-1$
, then
$n> |g(T^{v_k+1}x)| \geq n-1$
, then
![]() $k> K_n$
. Therefore,
$k> K_n$
. Therefore,
 $$ \begin{align*} \frac{|g(T^{v_k+1}x)|}{k} < \frac{n}{K_n} \to 0\quad\textrm{as } n\to \infty .\\[-34pt] \end{align*} $$
$$ \begin{align*} \frac{|g(T^{v_k+1}x)|}{k} < \frac{n}{K_n} \to 0\quad\textrm{as } n\to \infty .\\[-34pt] \end{align*} $$
Corollary B.2. Suppose
![]() $(X,{\mathcal B},\mu )$
is a standard probability space and
$(X,{\mathcal B},\mu )$
is a standard probability space and
![]() $f \in L^2_0(\mu )$
. There exists an ergodic invertible measure-preserving transformation T on
$f \in L^2_0(\mu )$
. There exists an ergodic invertible measure-preserving transformation T on
![]() $(X,{\mathcal B},\mu )$
such that all moving averages converge for f. In particular, for all strictly increasing
$(X,{\mathcal B},\mu )$
such that all moving averages converge for f. In particular, for all strictly increasing
![]() ,
,
![]() $v_n \in \mathbb {Z}$
, and a.e.
$v_n \in \mathbb {Z}$
, and a.e.
![]() $x\in X$
,
$x\in X$
,
 $$ \begin{align*} \lim_{n\to \infty} \frac{1}{L_n} \sum_{i=1}^{L_n} f(T^{v_n+i}x) = 0. \end{align*} $$
$$ \begin{align*} \lim_{n\to \infty} \frac{1}{L_n} \sum_{i=1}^{L_n} f(T^{v_n+i}x) = 0. \end{align*} $$