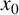QUASICONTINUITY, NONATTRACTING POINTS, DISTRIBUTIVE CHAOS AND RESISTANCE TO DISRUPTIONS

Abstract

We prove that any continuous function can be locally approximated at a fixed point $x_{0}$ by an uncountable family resistant to disruptions by the family of continuous functions for which $x_{0}$ is a fixed point. In that context, we also consider the property of quasicontinuity.

1 Introduction and preliminaries

Many papers examine disruptions of functions and dynamical systems with respect to certain properties (see, for example, [7, 10, 11]). The basis of our considerations is an observation articulated in Proposition 2.4, which states that distributional chaos for continuous functions at an arbitrary point may be disrupted by the family of all continuous functions. This prompts a natural question about the possibility of approximating a continuous function by a ‘big’ family of functions resistant to disruptions. Whereas approximating functions cannot be continuous, a natural requirement is to determine a property ‘close’ to continuity. Taking into account research conducted in [1, 3, 9], the chosen property is quasicontinuity (which is widely examined in real analysis).

Throughout the paper, we will use standard definitions and notation. Unless otherwise stated, we will consider functions mapping the unit interval $[0,1]$ into itself. Therefore, we will write $(a,b), [a,b]$ and so on, instead of the intersections $(a,b)\cap [0,1], [a,b]\cap [0,1]$ . By $\mathbb {N}$ , we denote the positive integers. The symbol $\operatorname {\mathrm {\#}} (A)$ means the cardinality of A. The symbol $f|_{A}$ denotes a restriction of the function f to A. We denote the set of all fixed points of f by $\textrm {Fix}(f)$ (that is, $x\in \textrm {Fix}(f)$ , if $f(x)=x$ ).

We employ the concept of a (discrete) dynamical system, following [2, 12]. Let X be a compact space. A topological dynamical system $(X,f)$ (denoted by $(f)$ ) is given by a map $f:X\rightarrow X$ . The evolution of the system is given by the successive iterations of the map, that is, $f^{0}(x)=x$ and $f^{n}(x)=(f\circ f^{n-1})(x)$ for $x\in X$ and $n\in \mathbb {N}$ . For $x\in X$ , the set $O_{f}(x)=\{f^{i}(x): i=0,1,2,\ldots \}$ is called an orbit.

Let $(f)$ be a dynamical system and let $Y\subset X$ . For $n \in \mathbb {N}$ and $\varepsilon> 0$ , we say that a set $E\subset Y$ is an $(n,Y,\varepsilon )$ -separated set if for all distinct points $x,y\in E$ , there exists $k\in [0,n-1]\cap \mathbb {N}$ such that $|f^{k}(x)-f^{k}(y)|> \varepsilon $ . Let $s_{n}(f,Y,\varepsilon )$ denote the maximal cardinality of an $(n,\varepsilon )$ -separated set in Y. The entropy of a dynamical system $(f)$ on $Y\subset X$ is the number

$$ \begin{align*}h(f,Y) = \lim\limits_{ \varepsilon\rightarrow 0}\limsup\limits_{ n\rightarrow \infty} \frac{1}{n}\log s_{n}(f,Y,\varepsilon).\end{align*} $$

If $Y=X$ , then we will omit the symbol X.

Let $f:I\rightarrow I$ , where I is a nondegenerate compact interval, be a continuous function and let $n\in \{2,3,\ldots \}$ . If $J_{1},J_{2},\ldots ,J_{n}$ are nondegenerate, closed and pairwise disjoint intervals such that $J_{1}\cup J_{2}\cup \cdots \cup J_{n}\subset f(J_{i})$ for all $i\in \{ 1,2,\ldots ,n\}$ , then $(J_{1}, J_{2},\ldots , J_{n})$ is called an n-horseshoe for f.

Following [5], we say that a function f is quasicontinuous at a point $x_{0}\in [0,1]$ if for any neighbourhood V of $x_{0}$ and for any neighbourhood W of $f(x_{0})$ , there exists a nonempty open set $U\subset V$ such that $f(U)\subset W$ . The function f is called quasicontinuous if it is quasicontinuous at every point $x\in [0,1]$ . From now on, by DQc, we denote the family of all Darboux and quasicontinuous functions.

Quasicontinuous dynamical systems (that is, systems such that $f^{n}$ is quasicontinuous for $n\in \mathbb {N}$ ) have been considered in [1, 3]. To ensure that all iterations of a quasicontinuous dynamical system have this property, in [9], quasicontinuity was connected with the Darboux property for the first time. The following lemma refers to these considerations in a more general situation.

Lemma 1.1. If $f,g\in \mathrm {DQc}$ , then $g\circ f\in \mathrm {DQc}$ .

Proof. Assume that $f,g\in \mathrm {DQc}$ . Obviously, $g\circ f$ is Darboux. For simplicity of notation, we will use symbols $(a,b), [a,b]$ and so on even in the case where $a>b$ .

Now, let $x_{0}\in [0,1]$ , $V=(x_{0}-\delta ,x_{0}+\delta )$ and $W=(g(f(x_{0}))-{\varepsilon },g(f(x_{0}))+{\varepsilon })$ . Of course, it is sufficient to consider only the case when there is $\delta _{1}>0$ such that $f([x_{0}-\delta _{1},x_{0}+\delta _{1}])\neq \{f(x_{0})\}$ .

Put $A_{0}=[x_{0}-\delta ,x_{0}+\delta ]$ . Then $f(A_{0})\ni f(x_{0})$ is a nondegenerate interval. Obviously, there exists $p_{1}\in (f(x_{0})-{\varepsilon },f(x_{0})+{\varepsilon })\cap (f(A_{0})\backslash \{f(x_{0})\})$ .

Now, let $A_{1}=[f(x_{0}),p_{1}]$ . Then $A_{1}\subset f(A_{0})$ . In the case where $g(A_{1})=\{g(f(x_{0}))\}$ , the proof is straightforward. So let us consider the situation $g(A_{1})\neq \{g(f(x_{0}))\}$ . The Darboux property of g implies that there is $p_{2}\in (g(f(x_{0}))-{\varepsilon },g(f(x_{0}))+{\varepsilon })\cap (g(A_{1})\backslash \{g(f(x_{0}))\})$ . Put $A_{2}=[g(f(x_{0})),p_{2}]\subset g(A_{1})$ . It is immediate that there exists $y_{2}\in A_{2}\backslash \{g(f(x_{0})), g(f(x_{0}-\delta )),g(f(x_{0}+\delta )),g(p_{1}),p_{2}\}\subset (g(f(x_{0})),p_{2})$ . Consequently, there is $y_{1}\in A_{1}$ such that $y_{2}=g(y_{1})$ . Obviously, $y_{1}\in (f(x_{0}),p_{1})\backslash \{f(x_{0}-\delta ),f(x_{0}+\delta )\}$ . By quasicontinuity of g at $y_{1}$ , there is an open set $U_{1}\subset (f(x_{0}),p_{1})\backslash \{f(x_{0}-\delta ),f(x_{0}+\delta )\}$ such that $g(U_{1})\subset (g(f(x_{0})),p_{2})\subset A_{2}\subset W$ .

Now, choose $z_{1}\in U_{1}$ . One can find $y_{0}\in A_{0}$ such that $z_{1}=f(y_{0})$ . Then $y_{0}\in V\backslash \{x_{0}\}$ . By quasicontinuity of f at $y_{0}$ , we infer that there is an open set $U_{0}\subset V\backslash \{x_{0}\}$ such that $f(U_{0})\subset U_{1}$ . It is easy to check that $g(f(U_{0}))\subset W$ .

In connection with distributional chaos, we will use the notation and concepts introduced in [13]. Let $(f)$ be a dynamical system and $x,y\in [0,1]$ . For $t>0$ , the functions,

$$ \begin{align*} \Phi^{*(f)}_{x,y} (t) &=\limsup\limits_{n\longrightarrow \infty}\frac{1}{n}\operatorname{\mathrm{\#}}(\{\kern1.5pt j\in [0,n-1]\cap\mathbb{N}:|f^{\,j}(x)-f^{\,j}(y)|<t\}),\\ \Phi^{(f)}_{x,y} (t) &=\liminf\limits_{n\longrightarrow \infty}\frac{1}{n}\operatorname{\mathrm{\#}}(\{\kern1.5pt j\in[0,n-1]\cap\mathbb{N}:|f^{\,j}(x)-f^{\,j}(y)|<t\}), \end{align*} $$

are respectively called upper and lower distribution function of x and y for the dynamical system $(f)$ .

Let $x,y\in [0,1]$ . We shall say that a pair $(x,y)$ is a DC-pair for a dynamical system $(f)$ if $\Phi ^{*(f)}_{x,y} (t)=1$ for any $t>0$ and there exists $t_{0}>0$ such that $\Phi ^{(f)}_{x,y} (t_{0})=0.$ A set $S\subset [0,1]$ is called a distributionally scrambled set (DS-set for brevity) for a dynamical system $(f)$ if $\operatorname {\mathrm {\#}} (S)>1$ and each pair of distinct points $x,y\in S$ forms a DC-pair. A dynamical system $(f)$ is distributionally chaotic if there exists an uncountable DS-set for this system.

The concept of the S-DC1 point is a slight modification of the DC1 point introduced in [7]. We say that $x_{0}$ is an S-DC1 point (point focusing distributional chaos) for a dynamical system $(f)$ (briefly, $x_{0}$ is an S-DC1 point for f) if for any ${\varepsilon }>0$ , there exist an uncountable DS-set S for the dynamical system $(f)$ and a strictly increasing sequence $\{n_{k}\}_{k\in \mathbb {N}}\subset \mathbb {N}$ such that $f^{ n_{k}}(S) \subset (x_{0}-{\varepsilon },x_{0}+{\varepsilon })$ .

In our considerations a special role is played by the ‘local approximation of functions’, which we obtain by means of equivalence classes considered in [6, 11]. Let $f,g$ be some functions, $x_{0}\in [0,1]$ and ${\varepsilon }>0$ . Then one can consider the equivalence relation , where . By the symbol $[f]_{x_{0}}^{{\varepsilon }}$ , we denote the equivalence class of f under relation ${{\varepsilon }}/{x_{0}}$ .

Many papers treat disruptions of dynamical systems (for example, [7, 10, 11]). We will adapt this term to the situation which we consider.

Let f be a function, $\mathcal {F}$ a family of functions and let ${\varepsilon }>0$ , $x_{0}\in [0,1]$ . We define the family $D_{{\varepsilon }}(f,\mathcal {F},x_{0})=\{g\circ f\in [f]_{x_{0}}^{{\varepsilon }}:g\in \mathcal {F}\}$ . Each function belonging to $D_{{\varepsilon }}(f,\mathcal {F},x_{0})$ is called an ${\varepsilon }$ -disruption of f at $x_{0}$ .

By C, we mean the family of all continuous functions $f:[0,1]\rightarrow [0,1]$ and we put $\textrm {FIX} (C,x_{0})=\{f\in C:x_{0}\in \textrm {Fix}(f)\}$ .

Let us observe that if we consider any function $f\in \textrm {FIX}(C,x_{0})$ , then for all ${\varepsilon }>0$ , it is easy to find a function $g\in D_{{\varepsilon }}(f,\textrm {FIX}(C,x_{0}),x_{0})$ such that $x_{0}$ is not an S-DC1 point of g. Therefore, our attention will be focused on a situation when $x_{0}\in \textrm {S-DC1}$ cannot be removed by disruptions. It is why we adopt the following definition.

Let $P(x_{0})$ be some property of functions at $x_{0}\in [0,1]$ . We will say that a family of functions $\mathcal {K}$ is resistant to disruptions by a family of functions $\mathcal {F}$ with respect to the property $P(x_{0})$ if each function $f\in \mathcal {K}$ has the property $P(x_{0})$ and there exists $\mu>0$ such that for any function $g\in \mathcal {K}$ , each function $\xi \in D_{\mu }(g,\mathcal {F},x_{0})$ has the property $P(x_{0})$ . If $\mathcal {K}=\{f\}$ , we will say that the function f is resistant to disruptions by a family $\mathcal {F}$ with respect to the property $P(x_{0})$ . Otherwise, we will say that f is not resistant to disruptions by $\mathcal {F}$ with respect to $P(x_{0})$ .

2 Various kinds of points and resistance to disruptions

In the theory of dynamical systems, a special role is played by attraction (for example, the well-known Lorenz attractor), including attracting points [4]. Usually, this relates to fixed points, even for noncontinuous functions [8]. In our considerations, we examine points with the opposite property, although they will also be associated with fixed points.

We say that $x_{0}\in [0,1]$ is a nonattracting point of a function f if for any $\alpha>0$ , there exists $\sigma \in (0,\alpha )$ such that

$$ \begin{align*}f([x_{0}-\sigma,x_{0}+\sigma])=[x_{0}-\sigma,x_{0}+\sigma]=f^{-1}([x_{0}-\sigma,x_{0}+\sigma]).\end{align*} $$

Proposition 2.1. If $x_{0}\in [0,1]$ is a nonattracting point of f, then $x_{0}\in \mathrm {Fix}(f)$ .

Proof. Assume that f and $x_{0}$ satisfy the assumptions of the proposition. Fix ${\varepsilon }>0$ and let $\{\delta _{n}\}_{n\in \mathbb {N}}\subset (0,{\varepsilon })$ be a strictly decreasing sequence converging to $0$ such that $f([x_{0}-\delta _{n},x_{0}+\delta _{n}])= [x_{0}-\delta _{n},x_{0}+\delta _{n}] \;\mathrm { for }\; n\in \mathbb {N}$ . Obviously, for any $n\in \mathbb {N}$ , we have $f(x_{0})\in f([x_{0}-\delta _{n},x_{0}+\delta _{n}])$ . Hence, $f(x_{0})\in \bigcap _{n=1}^{\infty }f([x_{0}-\delta _{n},x_{0}+\delta _{n}])=\bigcap _{n=1}^{\infty }[x_{0}-\delta _{n},x_{0}+\delta _{n}]=\{x_{0}\}$ .

The following proposition explains the name ‘nonattracting point’.

Proposition 2.2. If $x_{0}\in [0,1]$ is a nonattracting point of f, then for any $x\neq x_{0}$ , there exists $\delta _{x}>0$ such that $O_{f}(x)\cap [x_{0}-\delta _{x},x_{0}+\delta _{x}]=\emptyset $ .

Proof. Let us adopt the notation used in the proposition. Without loss of generality, we can assume that $x_{0}\in (0,1)$ . Let $x\in [0,1]\backslash \{x_{0}\}$ and let $\alpha =|x-x_{0}|$ . It follows that there exists $\delta _{x}\in (0,\alpha )$ such that $f([x_{0}-\delta _{x},x_{0}+\delta _{x}])=[x_{0}-\delta _{x},x_{0}+\delta _{x}]=f^{-1}([x_{0}-\delta _{x},x_{0}+\delta _{x}])$ .

Now we will proceed by induction to show that $f^{n}(x)\notin [x_{0}-\delta _{x},x_{0}+\delta _{x}]$ for $n=0,1,2,\ldots .$ Put $n=0$ . Since $\delta _{x}\in (0,\alpha )$ , it it easy to see that $f^{0}(x)=x\notin [x_{0}-\delta _{x},x_{0}+\delta _{x}]$ .

Now put $n=1$ . Suppose, contrary to our claim, that $f(x)\in [x_{0}-\delta _{x},x_{0}+\delta _{x}]$ . This clearly forces $x\in f^{-1}([x_{0}-\delta _{x},x_{0}+\delta _{x}])=[x_{0}-\delta _{x},x_{0}+\delta _{x}]$ . However, $x\notin [x_{0}-\delta _{x},x_{0}+\delta _{x}]$ , which is a contradiction.

Finally, let us assume that $f^{i}(x)\notin [x_{0}-\delta _{x},x_{0}+\delta _{x}]$ for some $i\in \mathbb {N}$ and suppose that $f^{i+1}(x)\in [x_{0}-\delta _{x},x_{0}+\delta _{x}]$ . Then, $f^{i}(x)\in f^{-1}([x_{0}-\delta _{x},x_{0}+\delta _{x}])=[x_{0}-\delta _{x},x_{0}+\delta _{x}]$ , which leads us to a contradiction.

Let us now note a useful lemma.

Lemma 2.3. Let f be a function. If there is $x_{0}\in [0,1]$ such that $f([x_{0}-\delta ,x_{0}+\delta ])=[x_{0}-\delta ,x_{0}+\delta ]=f^{-1}([x_{0}-\delta ,x_{0}+\delta ])$ and $x_{0}-\delta \in (0,1)$ for some $\delta>0$ (respectively $x_{0}+\delta \in (0,1)$ ) is a continuity point of f, then $f(x_{0}-\delta )\in \{x_{0}-\delta ,x_{0}+\delta \}$ (respectively $f(x_{0}+\delta )\in \{x_{0}-\delta ,x_{0}+\delta \}$ ).

Proof. Consider $f, \delta , x_{0}$ and $x_{0}-\delta $ satisfying the assumptions of the lemma. There is no loss of generality in assuming that $x_{0}\in (0,1)$ . Thus, $f(x_{0}-\delta )\in [x_{0}-\delta ,x_{0}+\delta ]$ . Suppose, contrary to our claim, that $f(x_{0}-\delta )\in (x_{0}-\delta ,x_{0}+\delta )$ .

Fix ${\varepsilon }>0$ such that $f(x_{0}-\delta )-{\varepsilon },f(x_{0}-\delta )+{\varepsilon }\in (x_{0}-\delta ,x_{0}+\delta )$ . Since f is continuous at $x_{0}-\delta $ , it follows that there is $r\in (0,{\varepsilon })$ such that $f((x_{0}-\delta -r,x_{0}-\delta ))\subset (f(x_{0}-\delta )-{\varepsilon },f(x_{0}-\delta )+{\varepsilon })$ .

Now, choose $y\in (x_{0}-\delta -r,x_{0}-\delta )$ so that $f(y)\in (f(x_{0}-\delta )-{\varepsilon },f(x_{0}-\delta )+{\varepsilon })\subset [x_{0}-\delta ,x_{0}+\delta ]$ . According to our assumption $f^{-1}([x_{0}-\delta ,x_{0}+\delta ])=[x_{0}-\delta ,x_{0}+\delta ]$ and $f(y)\in [x_{0}-\delta ,x_{0}+\delta ]$ , we have $f^{-1}(f(y))\subset [x_{0}-\delta ,x_{0}+\delta ]$ . In particular, $y\in f^{-1}(f(y))$ , so that $y\in [x_{0}-\delta ,x_{0}+\delta ]$ , which contradicts the fact that $y\in (x_{0}-\delta -r,x_{0}-\delta )$ .

The proof of $f(x_{0}+\delta )\in \{x_{0}-\delta ,x_{0}+\delta \}$ runs in a similar way.

The following statement shows the purposefulness of the considerations in Theorem 2.8.

Proposition 2.4. Let f be a continuous function such that $x_{0}\in (0,1)$ is a nonattracting point of f. Then f is not resistant to disruptions by $\mathrm {FIX}(C,x_{0})$ with respect to the property ‘ $x_{0}$ is an S-DC1 point’.

Proof. Let us adopt the notation used in the proposition. Fix $\mu>0$ . Since $x_{0}$ is a nonattracting point of f, it follows that there is $\gamma \in (0,\mu )$ such that $f([x_{0}-\gamma ,x_{0}+\gamma ])=[x_{0}-\gamma ,x_{0}+\gamma ]=f^{-1}([x_{0}-\gamma ,x_{0}+\gamma ])$ . Again, let us fix $\delta \in (0,\gamma )$ such that $f([x_{0}-\delta ,x_{0}+\delta ])=[x_{0}-\delta ,x_{0}+\delta ]=f^{-1}([x_{0}-\delta ,x_{0}+\delta ])$ . Now, consider the function g given by the formula

$$ \begin{align*} g(x)= \begin{cases} x & \mathrm{for}\ x\in [0,x_{0}-\gamma]\cup [x_{0}+\gamma,1],\\ x_{0}& \mathrm{for}\ x\in [x_{0}-\delta,x_{0}+\delta],\\ \mathrm{linear}& \mathrm{on\; the\; intervals}\ [x_{0}-\gamma,x_{0}-\delta],[x_{0}+\delta,x_{0}+\gamma]. \end{cases} \end{align*} $$

It is easy to see that g is continuous and $g\circ f\in [f]_{x_{0}}^{\mu }$ . Furthermore, $x_{0}$ is not an S-DC1 point for $g\circ f$ , since this function is constant on $[x_{0}-\delta ,x_{0}+\delta ]$ .

For later reference, we note a known fact.

Lemma 2.5 [12]

If $f:I\rightarrow I$ is a continuous function, where I is a nondegenerate compact interval, then $(f)$ is distributionally chaotic if and only if there exists an integer $n\geq 1$ such that $f^{n}$ has a 2-horseshoe.

Note that the term ‘S-DC1 point’ has been introduced for self maps. In the next theorem, we do not have that situation, so we refer to the relevant properties without using this notion.

Theorem 2.6. Let $p'\leq p<q\leq q'$ and let $f:[p,q]\rightarrow [p',q']$ be a continuous function, for which there exist points $p<a<b<c<d<q$ such that $f(a)=a=f(d)$ and $f(b)=d=f(c)$ . Then there exists an uncountable set S such that

$$ \begin{align*}O_{f}(s)\subset [a,b]\cup[c,d]\quad \text{for } s\in S,\end{align*} $$

and if $s,z\in S$ are distinct points, then $\Phi _{s,z}^{*(f)}(t)=1$ for all $t>0$ , and there is some $t_{0}>0$ such that $\Phi _{s,z}^{(f)}(t_{0})=0$ .

Proof. Let us adopt the notation in the lemma. Set $a_{1}\kern1.5pt{=}\kern1.5pt \sup \{x\kern1.5pt{\in}\kern1.5pt [a,b]:f(x)\kern1.5pt{=}\kern1.5pt a\}$ , $b_{1}\kern1.5pt{=}\kern1.5pt \inf \{x\in [a,b]:f(x)\kern1.5pt{=}\kern1.5pt d,x>a_{1}\}$ , $c_{1}\kern1.5pt{=}\kern1.5pt \sup \{x\kern1.5pt{\in}\kern1.5pt [c,d]:f(x)\kern1.5pt{=}\kern1.5pt d\}$ , $d_{1}\kern1.5pt{=}\kern1.5pt \inf \{x\kern1.5pt{\in}\kern1.5pt [c,d]: f(x)=a,x>c_{1}\}$ . We see at once that $f(a_{1})=a=f(d_{1})$ , $f(b_{1})=d=f(c_{1})$ and $p<a_{1}<b_{1}<c_{1}<d_{1}<q$ .

Now, let us consider the function $f_{0}:[p,q]\rightarrow [p,q]$ defined by

$$ \begin{align*} f_{0}(x)= \begin{cases} a&\mathrm{ for }\ x\in [p,a_{1}]\cup [d_{1},q], \\ f(x)&\mathrm{ for }\ x\in [a_{1},b_{1}]\cup [c_{1},d_{1}], \\ d&\mathrm{ for }\ x\in [b_{1},c_{1}]. \end{cases} \end{align*} $$

One can easily see that $f_{0}$ is a continuous function with the property $f_{0}([a_{1},b_{1}])=[a,d]=f_{0}([c_{1},d_{1}])$ .

We will show that there exists a DS-set S for $(f_{0})$ . Clearly, $([a_{1},b_{1}],[c_{1},d_{1}])$ forms a horseshoe for $f_{0}$ . By Lemma 2.5, there exists an uncountable DS-set $S_{0}$ for $(f_{0})$ .

Now, we will prove that

(2.1) $$ \begin{align} \operatorname{\mathrm{\#}} (\{x\in S_{0}: O_{f_{0}}(x)\not\subset [a_{1},b_{1}]\cup [c_{1},d_{1}]\})\leq 1. \end{align} $$

Suppose, contrary to our claim, that there exist two distinct points $x_{1}$ , $x_{2}\in S_{0}$ and $n_{1},n_{2}\in \mathbb {N}$ such that $(f_{0})^{n_{i}}(x_{i})\notin [a_{1},b_{1}]\cup [c_{1},d_{1}]$ for $i\in \{1,2\}$ . Therefore, $(f_{0})^{n_{i}+1}(x_{i})\in \{a,d\}$ . Let us note that if $(f_{0})^{n_{i}+1}(x_{i})=a$ (for some $i\in \{1,2\}$ ), then $(f_{0})^{m}(x_{i})=a$ for $m\geq n_{i}+1$ and likewise, if $(f_{0})^{n_{i}+1}(x_{i})=d$ , then $(f_{0})^{n_{i}+2}(x_{i})=a$ . Put $n_{0}=\max \{n_{1}+ 2, n_{2}+2\}$ . It follows that $(f_{0})^{n}(x_{i})=a\;\mathrm { for }\; n\geq n_{0}\;\mathrm { and }\; i\in \{1,2\}$ . Recall that $x_{1},x_{2}\in S_{0}$ , and hence for some $t_{0}>0$ ,

(2.2) $$ \begin{align} \Phi_{x_{1},x_{2}}^{(f_{0})}(t_{0})=\liminf\limits_{n\longrightarrow\infty}\frac{1}{n}\operatorname{\mathrm{\#}}(\{\,j\in[0,n-1]\cap\mathbb{N} : |(f_{0})^{\,j}(x_{1})-(f_{0})^{\,j}(x_{2})|<t_{0}\})=0. \end{align} $$

However, one can infer that

$$ \begin{align*}\frac{1}{n}\operatorname{\mathrm{\#}}(\{\kern1.5pt j\in[0,n-1]\cap\mathbb{N}: |(f_{0})^{\,j}(x_{1})-(f_{0})^{\,j}(x_{2})|<t_{0}\})\geq \frac{n-n_{0}}{n}\quad \mathrm{for\; any }\; n>n_{0},\end{align*} $$

which obviously leads us to a contradiction with (2.2). This proves (2.1).

Put $S=S_{0}\backslash \{x\in S_{0}: O_{f_{0}}(x)\not \subset [a_{1},b_{1}]\cup [c_{1},d_{1}]\}$ . From (2.1), we conclude that S is an uncountable set. Of course, if $x\in S$ , then $O_{f_{0}}(x)\subset [a_{1},b_{1}]\cup [c_{1},d_{1}]$ , which leads to the conclusion that $S\subset [a_{1},b_{1}]\cup [c_{1},d_{1}]$ .

What is left is to show that S is a DS-set for $(f)$ . It is easy to prove, by induction, that $(f_{0})^{n}(x)=f^{n}(x)$ for all $x\in S$ and all $n\in \mathbb {N}\cup \{0\}$ . Hence, for any $z_{1},z_{2}\in S$ and any $t>0$ , we have $1=\Phi _{z_{1},z_{2}}^{*(f_{0})}(t)=\Phi _{z_{1},z_{2}}^{*(f)}(t)$ . Now, fix $t_{1}>0$ such that $\Phi _{z_{1},z_{2}}^{(f_{0})}(t_{1})=0$ . Thus, by (2.2), $0=\Phi _{z_{1},z_{2}}^{(f_{0})}(t_{1})= \Phi _{z_{1},z_{2}}^{(f)}(t_{1})$ . This finishes the proof of the theorem.

Building the intuition related to the concept of a nonattracting point, let us note the following statement.

Proposition 2.7. Let f be a Darboux function and $x_{0}\in (0,1)$ be a nonattracting point of f. Then, for any $\delta>0$ such that $f([x_{0}-\delta ,x_{0}+\delta ])=[x_{0}-\delta ,x_{0}+\delta ]=f^{-1}([x_{0}-\delta ,x_{0}+\delta ])$ , there are two possibilities:

$$ \begin{align*} f([x_{0}-\delta,x_{0}))= [x_{0}-\delta,x_{0})\quad\text{and}\quad f((x_{0},x_{0}+\delta])= (x_{0},x_{0}+\delta] \end{align*} $$

or

$$ \begin{align*} f([x_{0}-\delta,x_{0}))= (x_{0},x_{0}+\delta] \quad\text{and}\quad f((x_{0},x_{0}+\delta])=[x_{0}-\delta,x_{0}). \end{align*} $$

Proof. Let us adopt the notation used in the proposition. Clearly, Proposition 2.1 implies that $x_{0}\in \mathrm {Fix}(f)$ . First, let us prove that

(2.3) $$ \begin{align} x_{0}\notin f([x_{0}-\delta,x_{0}+\delta]\backslash\{x_{0}\}). \end{align} $$

Suppose, contrary to our claim, that $x_{0}\in f([x_{0}-\delta ,x_{0}+\delta ]\backslash \{x_{0}\})$ . Assume, for example, that $x_{0}\in f([x_{0}-\delta ,x_{0}))$ . Then there is $t\in [x_{0}-\delta ,x_{0})$ such that $f(t)=x_{0}$ . By the definition of a nonattracting point, we conclude that there exists $\sigma \in (0,x_{0}-t)$ such that

(2.4) $$ \begin{align} f([x_{0}-\sigma,x_{0}+\sigma])=[x_{0}-\sigma,x_{0}+\sigma]=f^{-1}([x_{0}-\sigma,x_{0}+\sigma]). \end{align} $$

Of course, $t\in f^{-1}([x_{0}-\sigma ,x_{0}+\sigma ])$ . Moreover, we have $t\notin [x_{0}-\sigma ,x_{0}+\sigma ]$ (because $t<x_{0}-\sigma $ ), and hence $f^{-1}([x_{0}-\sigma ,x_{0}+\sigma ])\backslash [x_{0}-\sigma ,x_{0}+\sigma ]\neq \emptyset $ , contrary to (2.4). This contradiction proves that $x_{0}\notin f([x_{0}-\delta ,x_{0}))$ .

In the case where $x_{0}\in f((x_{0},x_{0}+\delta ])$ , the proof runs in a similar way. This finishes the proof of (2.3).

Now, let $\alpha \in f([x_{0}-\delta ,x_{0}))$ . Then there exists $a\in [x_{0}-\delta ,x_{0}) $ such that $f(a)=\alpha $ . According to (2.3), we obtain $\alpha \neq x_{0}$ . Consequently, we have two possibilities. First, let us suppose that $\alpha \in [x_{0}-\delta ,x_{0})$ . We start by showing that in this case,

(2.5) $$ \begin{align} f([x_{0}-\delta,x_{0}))\subset [x_{0}-\delta,x_{0}). \end{align} $$

Suppose, contrary to (2.5), that there is a point $b\in [x_{0}-\delta ,x_{0})$ such that $f(b)>x_{0}$ . Then, $a,b\in [x_{0}-\delta ,x_{0})$ and $f(a)=\alpha <x_{0}<f(b)$ . Since f is Darboux, it follows that there exists $c\in [x_{0}-\delta ,x_{0})$ satisfying $f(c)=x_{0}$ , and in consequence, $x_{0}\in f([x_{0}-\delta ,x_{0}))$ , contrary to (2.3). Thus, $f([x_{0}-\delta ,x_{0}))\subset (-\infty ,x_{0})$ . According to our assumption $f([x_{0}-\delta ,x_{0}))\subset [x_{0}-\delta ,x_{0}+\delta ]$ , it follows that $f([x_{0}-\delta ,x_{0}))\subset [x_{0}-\delta ,x_{0}]$ . This combined with (2.3) proves (2.5).

Now, we will show that in this case,

(2.6) $$ \begin{align} f((x_{0},x_{0}+\delta])= (x_{0},x_{0}+\delta]. \end{align} $$

From the considerations above and the equality $f([x_{0}-\delta ,x_{0}+\delta ])=[x_{0}-\delta ,x_{0}+\delta ]$ , it follows that

(2.7) $$ \begin{align} (x_{0},x_{0}+\delta]\subset f((x_{0},x_{0}+\delta]). \end{align} $$

Now we will show the opposite inclusion. Let $e\in (x_{0},x_{0}+\delta ]$ . From (2.7), there exists $o\in (x_{0},x_{0}+\delta ]$ such that $e=f(o)$ . Suppose, contrary to our claim, that there is $h\in (x_{0},x_{0}+\delta ]$ such that $f(h)<x_{0}$ . We have $o,h\in (x_{0},x_{0}+\delta ]$ and $f(h)<x_{0}<e=f(o)$ . Since f is Darboux, there exists $z\in (x_{0},x_{0}+\delta ]$ satisfying $f(z)=x_{0}$ , that is, $x_{0}\in f((x_{0},x_{0}+\delta ])$ , contrary to (2.3). Thus, $f((x_{0},x_{0}+\delta ])\subset (x_{0},\infty )$ . Moreover, $f((x_{0},x_{0}+\delta ])\subset [x_{0}-\delta ,x_{0}+\delta ]\cap (x_{0},\infty )=(x_{0},x_{0}+\delta ]$ . Thus from (2.7), we obtain $f((x_{0},x_{0}+\delta ])=(x_{0},x_{0}+\delta ]$ , which proves (2.6).

From (2.3), (2.5), (2.6) and the assumed equality ${f([x_{0}\kern1.5pt{-}\kern1.5pt\delta ,x_{0}\kern1.5pt{+}\kern1.5pt\delta ])\kern1.5pt{=}\kern1.5pt[x_{0}\kern1.5pt{-}\kern1.5pt\delta ,x_{0}\kern1.5pt{+}\kern1.5pt\delta ]}$ , we have $f([x_{0}-\delta ,x_{0}))=[x_{0}-\delta ,x_{0})$ .

For other cases, the proof runs in a similar way.

As pointed out in the Section 1, Proposition 2.4 suggests a question about the possibility of approximating the continuous functions by a family resistant to disruptions with respect to the property connected with an S-DC1 point. The answer is given in the next theorem.

Let $\mathrm {Qc}(x_{0})$ (respectively S-DC1 $(x_{0})$ ) denote the property of functions such that $x_{0}$ is a quasicontinuity point of a function (respectively $x_{0}$ is an S-DC1 point of a function). Now we will state and prove the main result of our paper.

Theorem 2.8. Let f be a continuous function such that $x_{0}\in [0,1]$ is a nonattracting point of f. Then for any ${\varepsilon }>0$ , there exists an uncountable family $\mathcal {K}\subset D_{{\varepsilon }}(f,\mathrm {DQc},x_{0})$ , which is resistant to disruptions by the family $\mathrm {FIX} (C,x_{0})$ with respect to the following properties:

1. (a) the property $\mathrm {Qc}(x_{0})$ ;

2. (b) the property S-DC1 $(x_{0})$ .

Proof. Without loss of generality, we can assume that $x_{0}\in (0,1)$ (if $x_{0}\in \{0,1\}$ , the proof is analogous). By Proposition 2.1, it is obvious that $x_{0}\in \mathrm {Fix}(f)$ . We shall examine this proof for fixed ${\varepsilon }>0$ . Obviously, there exists $\delta \in (0,{\varepsilon })$ , which is a number such that $f([x_{0}-\delta ,x_{0}+\delta ])=[x_{0}-\delta ,x_{0}+\delta ]=f^{-1}([x_{0}-\delta ,x_{0}+\delta ])$ .

By Proposition 2.7, we can find a strictly monotone sequence $\{x_{n}\}_{n\in \mathbb {N}}\subset (x_{0}-\delta ,x_{0})$ converging to $x_{0}$ such that $\{f(x_{n})\}_{n\in \mathbb {N}}$ is strictly monotone and $f(x_{n})\neq x_{0}$ for all $n\in \mathbb {N}$ .

We will consider the case when $\{f(x_{n})\}_{n\in \mathbb {N}}$ is strictly decreasing. This implies that $\{f(x_{n})\}_{n\in \mathbb {N}}\subset (x_{0},x_{0}+\delta ]$ . Let us put $s_{n}=\min \{x\in [x_{n},x_{n+1}]:f(x)=f(x_{n+1})\}$ for $n\in \mathbb {N}$ . Continuity of f implies that

$$ \begin{align*} f(x_{n+1})=f(s_{n})<f(x_{n}),\quad f([x_{n},s_{n}))\subset (f(x_{n+1}),1]\quad \mathrm{and}\quad x_{0}\notin f([x_{n},s_{n}]). \end{align*} $$

For each $\alpha \in (x_{0}-x_{2},x_{0}-x_{1})$ , let $\beta _{\alpha }=x_{0}+\alpha \in (x_{0},x_{0}+\delta )$ . Let us choose $z_{n}\in [x_{n},s_{n}]$ such that $f(z_{n})=f(s_{n})+\tfrac 12(f(x_{n})-f(s_{n}))$ for $n\in \mathbb {N}$ . Then, we define the functions $g_{\alpha }:[0,1]\rightarrow [0,1]$ for $\alpha \in (x_{0}-x_{2},x_{0}-x_{1})$ by

$$ \begin{align*} g_{\alpha}(x)= \begin{cases} x & \mathrm{for} \ x\in [0,x_{0}]\cup [x_{0}+\delta,1]\mathrm{,}\\ x_{1}& \mathrm{for} \ x\in \{f(x_{n}):n\in\mathbb{N}\}\textrm{,} \\ \beta_{\alpha}& \mathrm{for} \ x\in \{f(z_{n}):n\in\mathbb{N}\}\textrm{,}\\ \mathrm{linear} & \mathrm{on\; the\; intervals} \ [f(x_{n+1}),f(z_{n})], [f(z_{n}),f(x_{n})], \,[f(x_{1}),x_{0}+\delta] \\ & \quad \mathrm{ for }\; n\in\mathbb{N}\textrm{.} \end{cases} \end{align*} $$

Put $f_{\alpha }=g_{\alpha }\circ f$ for $\alpha \in (x_{0}-x_{2},x_{0}-x_{1})$ and let $\mathcal {K}=\{f_{\alpha }:\alpha \in (x_{0}-x_{2},x_{0}-x_{1})\}$ . An easy computation shows that $\mathcal {K}\subset [f]_{x_{0}}^{{\varepsilon }}$ .

We are now in a position to show that for each $\alpha \in (x_{0}-x_{2},x_{0}-x_{1})$ , the function $f_{\alpha }$ is resistant to disruptions by the family $\mathrm {FIX} (C,x_{0})$ with respect to each of the properties (a) and (b). Fix $\alpha _{0}\in (x_{0}-x_{2},x_{0}-x_{1})$ and put $\mu ={(x_{0}-x_{3})}/{2}$ . Obviously, $f_{\alpha _{0}}\in \mathrm {DQc}$ . Fix $\xi \in D_{\mu }(f_{\alpha _{0}},\mathrm {FIX} (C,x_{0}),x_{0})$ , that is, $\xi =\tau \circ f_{\alpha _{0}}$ , where $\tau \in \mathrm {FIX} (C,x_{0})$ . By Lemma 1.1, the function $\xi \in \mathrm {DQc}$ .

Now we will prove that $x_{0}$ is an S-DC1 point for the dynamical system $(\xi )$ . To do so, fix $\sigma \in (0,\mu )$ . Recall that $\xi =\tau \circ f_{\alpha _{0}}=\tau \circ g_{\alpha _{0}}\circ f$ , where $\tau \in \mathrm {FIX} (C,x_{0})$ and, moreover, $\xi \in [f_{\alpha _{0}}]_{x_{0}}^{\mu }$ . Obviously, there exists $m\in \mathbb {N}$ such that $x_{m}\in (x_{0}-\sigma ,x_{0})$ . Note that $f_{\alpha _{0}}(x_{m})=x_{1}=f_{\alpha _{0}}(x_{m+1})$ and $f_{\alpha _{0}}(z_{m})=\beta _{\alpha _{0}}$ . Moreover,

(2.8) $$ \begin{align} \xi(x_{m+1})=\xi(x_{m})<x_{0}-\frac{\alpha_{0}}{2}<x_{0}+\frac{\alpha_{0}}{2}<\xi(z_{m}). \end{align} $$

The first inequality of (2.8) is obtained by showing that $\xi (x_{m})<x_{1}+\mu $ . Indeed, we show that $\xi (x_{m})=x_{1}$ . Conversely, suppose that $\xi (x_{m})\neq x_{1}$ . Since $\xi \in [f_{\alpha _{0}}]_{x_{0}}^{\mu }$ , we conclude by our supposition that $x_{m},f_{\alpha _{0}}(x_{m})\in (x_{0}-\mu ,x_{0}+\mu )$ . However, $f_{\alpha _{0}}(x_{m})=x_{1}\notin (x_{0}-\mu ,x_{0}+\mu )\subset (x_{1},x_{0}+\alpha _{0})$ , which is a contradiction. Thus, $\xi (x_{m})=f_{\alpha _{0}}(x_{m})=x_{1}<x_{1}+\mu <x_{1}+\tfrac 12\alpha _{0}<x_{0}-\tfrac 12\alpha _{0}$ .

To prove the last inequality of (2.8), it is sufficient to observe that $\xi (z_{m})=\beta _{\alpha _{0}}$ . Indeed, suppose contrary to our claim that $\xi (z_{m})\neq \beta _{\alpha _{0}}$ . Since $\xi \in [f_{\alpha _{0}}]_{x_{0}}^{\mu }$ , we have $z_{m},f_{\alpha _{0}}(z_{m})\kern1.5pt{\in}\kern1.5pt (x_{0}-\mu ,x_{0}+\mu )$ . However, $f_{\alpha _{0}}(z_{m})\kern1.5pt{=}\kern1.5pt\beta _{\alpha _{0}}\kern1.5pt{\notin}\kern1.5pt (x_{0}-\mu ,x_{0}+\mu )\kern1.5pt{\subset}\kern1.5pt (x_{1},x_{0}+\alpha _{0})$ , which is a contradiction. Therefore, $\xi (z_{m})>\beta _{\alpha _{0}}-\mu >\beta _{\alpha _{0}}-\tfrac 12\alpha _{0}=x_{0}+\tfrac 12\alpha _{0}$ . Since $\xi |_{[x_{m},s_{m}]}$ is a continuous function, (2.8) shows that there is $a\in (x_{m},z_{m})$ such that $\xi (a)=a$ . Moreover, there exists $d\in (z_{m},s_{m})$ such that $\xi (d)=a$ . We shall also conclude from (2.8) that $\xi (z_{m})>x_{0}+\tfrac 12\alpha _{0}>d$ and $\xi (d)=a<d$ . By continuity of $\xi |_{[x_{m},s_{m}]}$ , it follows that there exists $c\in (z_{m},d)$ such that $\xi (c)=d$ . Furthermore, $\xi (a)=a<d<\xi (z_{m})$ and $\xi |_{[x_{m},s_{m}]}$ is Darboux, and hence there is a point $b\in (a,z_{m})$ such that $\xi (b)=d$ .

Finally, $x_{m}< a<b<c<d< s_{m}$ . The above considerations show that $\xi |_{[x_{m},s_{m}]}:[x_{m},s_{m}]\rightarrow [0,1]$ satisfies the assumptions of Theorem 2.6 (with $p'=0,p=x_{m},q=s_{m},q'=1$ ). Consequently, there exists an uncountable DS-set S for $(\xi )$ such that $O_{\xi }(s)\subset [a,b]\cup [c,d]$ for all $s\in S$ .

What is left is to show that there is a sequence $\{n_{k}\}_{k\in \mathbb {N}}$ such that $S\subset \xi ^{n_{k}}(S)\subset (x_{0}-\sigma ,x_{0}+\sigma )$ . For this purpose, let us put $\{n_{k}\}_{k\in \mathbb {N}}=\{1,2,\ldots \}$ . Fix $s\in S$ . Then, by Theorem 2.6, we have $\xi ^{n_{k}}(s)\in O_{\xi }(s)\subset [a,b]\cup [c,d] \subset (x_{0}-\sigma ,x_{0}+\sigma )$ for any $n\in \mathbb {N}$ .