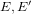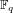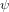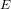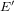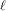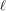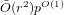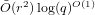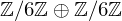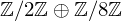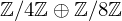Consider two ordinary elliptic curves $E,E^{\prime }$ defined over a finite field $\mathbb{F}_{q}$, and suppose that there exists an isogeny $\unicode[STIX]{x1D713}$ between $E$ and $E^{\prime }$. We propose an algorithm that determines $\unicode[STIX]{x1D713}$ from the knowledge of $E$, $E^{\prime }$ and of its degree $r$, by using the structure of the $\ell$-torsion of the curves (where $\ell$ is a prime different from the characteristic $p$ of the base field). Our approach is inspired by a previous algorithm due to Couveignes, which involved computations using the $p$-torsion on the curves. The most refined version of that algorithm, due to De Feo, has a complexity of $\tilde{O} (r^{2})p^{O(1)}$ base field operations. On the other hand, the cost of our algorithm is $\tilde{O} (r^{2})\log (q)^{O(1)}$, for a large class of inputs; this makes it an interesting alternative for the medium- and large-characteristic cases.

We present JKL-ECM, an implementation of the elliptic curve method of integer factorization which uses certain twisted Hessian curves in a family studied by Jeon, Kim and Lee. This implementation takes advantage of torsion subgroup injection for families of elliptic curves over a quartic number field, in addition to the ‘small parameter’ speedup. We produced thousands of curves with torsion 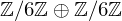$\mathbb{Z}/6\mathbb{Z}\oplus \mathbb{Z}/6\mathbb{Z}$ and small parameters in twisted Hessian form, which admit curve arithmetic that is ‘almost’ as fast as that of twisted Edwards form. This allows JKL-ECM to compete with GMP-ECM for finding large prime factors. Also, JKL-ECM, based on GMP, accepts integers of arbitrary size. We classify the torsion subgroups of Hessian curves over 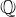$\mathbb{Q}$ and further examine torsion properties of the curves described by Jeon, Kim and Lee. In addition, the high-performance curves with torsion 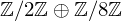$\mathbb{Z}/2\mathbb{Z}\oplus \mathbb{Z}/8\mathbb{Z}$ of Bernstein et al. are completely recovered by the 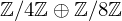$\mathbb{Z}/4\mathbb{Z}\oplus \mathbb{Z}/8\mathbb{Z}$ family of Jeon, Kim and Lee, and hundreds more curves are produced besides, all with small parameters and base points.

Given an elliptic curve E over a field of positive characteristic p, we consider how to efficiently determine whether E is ordinary or supersingular. We analyze the complexity of several existing algorithms and then present a new approach that exploits structural differences between ordinary and supersingular isogeny graphs. This yields a simple algorithm that, given E and a suitable non-residue in 𝔽p2, determines the supersingularity of E in O(n3log 2n) time and O(n) space, where n=O(log p) . Both these complexity bounds are significant improvements over existing methods, as we demonstrate with some practical computations.

Given a prime q and a negative discriminant D, the CM method constructs an elliptic curve E/Fq by obtaining a root of the Hilbert class polynomial HD(X) modulo q. We consider an approach based on a decomposition of the ring class field defined by HD, which we adapt to a CRT setting. This yields two algorithms, each of which obtains a root of HD mod q without necessarily computing any of its coefficients. Heuristically, our approach uses asymptotically less time and space than the standard CM method for almost all D. Under the GRH, and reasonable assumptions about the size of log q relative to ∣D∣, we achieve a space complexity of O((m+n)log q) bits, where mn=h(D) , which may be as small as O(∣D∣1/4 log q) . The practical efficiency of the algorithms is demonstrated using ∣D∣>1016 and q≈2256, and also ∣D∣>1015 and q≈233220. These examples are both an order of magnitude larger than the best previous results obtained with the CM method.

In his celebrated memoir, Morgan Ward's definition of elliptic divisibility sequences has the remarkable feature that it does not become at all clear until deep into the paper that there exist nontrivial examples of such sequences. Even then, Ward's proof of the coherence of his definition relies on displaying a sequence of values of quotients of Weierstraß $\sigma$-functions. We give a direct proof of coherence and show, rather more generally, that a sequence defined by a so-called Somos relation of width 4 is always also given by three-term Somos relations of all larger widths $5, 6, 7, \ldots.$