Refine search
Actions for selected content:
1 results
The volume of a random simplex in an n-ball is asymptotically normal
-
- Journal:
- Journal of Applied Probability / Volume 14 / Issue 3 / September 1977
- Published online by Cambridge University Press:
- 14 July 2016, pp. 647-653
- Print publication:
- September 1977
-
- Article
- Export citation
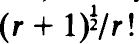 and
and  , respectively.
, respectively.