Let 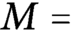 $M=$
diag
$M=$
diag 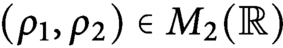 $(\rho _1,\rho _2)\in M_{2}({\mathbb R})$
be an expanding matrix and Let
$(\rho _1,\rho _2)\in M_{2}({\mathbb R})$
be an expanding matrix and Let  $\{D_n\}_{n=1}^{\infty }$
be a sequence of digit sets with
$\{D_n\}_{n=1}^{\infty }$
be a sequence of digit sets with 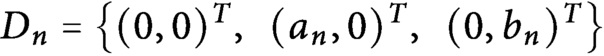 $D_n=\left \{(0, 0)^T,\,\,\,(a_n, 0 )^T, \,\,\, (0, b_n )^T \right \}$
, where
$D_n=\left \{(0, 0)^T,\,\,\,(a_n, 0 )^T, \,\,\, (0, b_n )^T \right \}$
, where 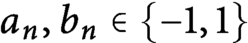 $a_n, b_n\in \{-1,1\}$
. The associated Borel probability measure
$a_n, b_n\in \{-1,1\}$
. The associated Borel probability measure 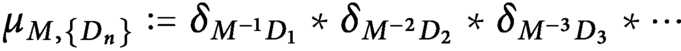 $$ \begin{align*} \mu_{M,\{D_n\}}:=\delta_{M^{-1}D_1}\ast \delta_{M^{-2}D_2}\ast \delta_{M^{-3}D_3}\ast \cdots \end{align*} $$
$$ \begin{align*} \mu_{M,\{D_n\}}:=\delta_{M^{-1}D_1}\ast \delta_{M^{-2}D_2}\ast \delta_{M^{-3}D_3}\ast \cdots \end{align*} $$
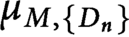 $\mu _{M, \{D_n\}}$
is a spectral measure if and only if
$\mu _{M, \{D_n\}}$
is a spectral measure if and only if  $3\mid \rho _i$
for each
$3\mid \rho _i$
for each  $i=1, 2$
. The special case is the Sierpinski-type measure with
$i=1, 2$
. The special case is the Sierpinski-type measure with 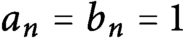 $a_n=b_n=1$
for all
$a_n=b_n=1$
for all 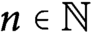 $n\in {\mathbb N}$
, which is proved by Dai et al. [Appl. Comput. Harmon. Anal. (2020), https://doi.org/10.1016/j.acha.2019.12.001].
$n\in {\mathbb N}$
, which is proved by Dai et al. [Appl. Comput. Harmon. Anal. (2020), https://doi.org/10.1016/j.acha.2019.12.001].
 ${\mathbb R}^2$
${\mathbb R}^2$