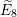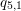A log symplectic manifold is a complex manifold equipped with a complex symplectic form that has simple poles on a hypersurface. The possible singularities of such a hypersurface are heavily constrained. We introduce the notion of an elliptic point of a log symplectic structure, which is a singular point at which a natural transversality condition involving the modular vector field is satisfied, and we prove a local normal form for such points that involves the simple elliptic surface singularities $\widetilde{E}_{6},\widetilde{E}_{7}$ and $\widetilde{E}_{8}$. Our main application is to the classification of Poisson brackets on Fano fourfolds. For example, we show that Feigin and Odesskii’s Poisson structures of type $q_{5,1}$ are the only log symplectic structures on projective four-space whose singular points are all elliptic.

In this paper we consider the dynamical system involved by the Ricci operator on the space of Kähler metrics of a Fano manifold. Nadel has defined an iteration scheme given by the Ricci operator and asked whether it has some non-trivial periodic points. First, we prove that no such periodic points can exist. We define the inverse of the Ricci operator and consider the dynamical behaviour of its iterates for a Fano Kähler–Einstein manifold. Then we define a finite-dimensional procedure to give an approximation of Kähler–Einstein metrics using this iterative procedure and apply it on ℂℙ2 blown up in three points.

Let X be a complex-projective contact manifold with $b_2(X)=1$. It has long been conjectured that X should then be rational-homogeneous, or equivalently, that there exists an embedding $X \to {\mathbb P}^n$ whose image contains lines.

We show that X is covered by a compact family of rational curves, called ‘contact lines’, that behave very much like the lines on the rational-homogeneous examples: if $x \in X$ is a general point, then all contact lines through x are smooth, no two of them share a common tangent direction at x, and the union of all contact lines through x forms a cone over an irreducible, smooth base. As a corollary, we obtain that the tangent bundle of X is stable.