The infinitely divisible distributions on ${{\mathbb{R}}^{+}}$ of random variables 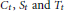${{C}_{t}},\,{{S}_{t}}\,\text{and}\,{{T}_{t}}$ with Laplace transforms

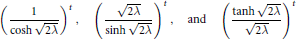$${{\left( \frac{1}{\cosh \sqrt{2\lambda }} \right)}^{t}},{{\left( \frac{\sqrt{2\lambda }}{\sinh \sqrt{2\lambda }} \right)}^{t}},\,\text{and}\,{{\left( \frac{\tanh \sqrt{2\lambda }}{\sqrt{2\lambda }} \right)}^{t}}$$

respectively are characterized for various 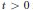$t\,>\,0$ in a number of different ways: by simple relations between their moments and cumulants, by corresponding relations between the distributions and their Lévy measures, by recursions for their Mellin transforms, and by differential equations satisfied by their Laplace transforms. Some of these results are interpreted probabilistically via known appearances of these distributions for 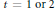$t\,=\,1\,\,\text{or}\,2$ in the description of the laws of various functionals of Brownian motion and Bessel processes, such as the heights and lengths of excursions of a one-dimensional Brownian motion. The distributions of 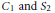${{C}_{1}}\,\text{and}\,{{S}_{2}}$ are also known to appear in the Mellin representations of two important functions in analytic number theory, the Riemann zeta function and the Dirichlet 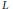$L$-function associated with the quadratic character modulo 4. Related families of infinitely divisible laws, including the gamma, logistic and generalized hyperbolic secant distributions, are derived from 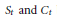${{S}_{t}}\,\text{and}\,{{C}_{t}}$ by operations such as Brownian subordination, exponential tilting, and weak limits, and characterized in various ways.