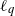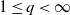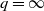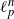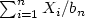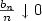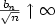Refine search
Actions for selected content:
19 results
Moderate deviations for weight-dependent random connection models
- Part of
-
- Journal:
- Journal of Applied Probability , First View
- Published online by Cambridge University Press:
- 04 June 2025, pp. 1-23
-
- Article
- Export citation
Sample path moderate deviations for non-stationary power-law shot noise processes
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 62 / Issue 3 / September 2025
- Published online by Cambridge University Press:
- 28 March 2025, pp. 1105-1131
- Print publication:
- September 2025
-
- Article
- Export citation
Gaussian approximation and moderate deviations of Poisson shot noises with application to compound generalized Hawkes processes
- Part of
-
- Journal:
- Advances in Applied Probability / Volume 57 / Issue 1 / March 2025
- Published online by Cambridge University Press:
- 02 December 2024, pp. 305-345
- Print publication:
- March 2025
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Asymptotic results for sums and extremes
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 61 / Issue 4 / December 2024
- Published online by Cambridge University Press:
- 13 March 2024, pp. 1153-1171
- Print publication:
- December 2024
-
- Article
- Export citation
Moderate deviations inequalities for Gaussian process regression
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 61 / Issue 1 / March 2024
- Published online by Cambridge University Press:
- 05 June 2023, pp. 172-197
- Print publication:
- March 2024
-
- Article
- Export citation
Limit theorems for random points in a simplex
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 59 / Issue 3 / September 2022
- Published online by Cambridge University Press:
- 21 June 2022, pp. 685-701
- Print publication:
- September 2022
-
- Article
- Export citation
Asymptotic behavior of the weak approximation to a class of Gaussian processes
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 58 / Issue 3 / September 2021
- Published online by Cambridge University Press:
- 16 September 2021, pp. 693-707
- Print publication:
- September 2021
-
- Article
- Export citation
Small-time moderate deviations for the randomised Heston model
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 57 / Issue 1 / March 2020
- Published online by Cambridge University Press:
- 04 May 2020, pp. 19-28
- Print publication:
- March 2020
-
- Article
- Export citation
Moderate deviations-based importance sampling for stochastic recursive equations
- Part of
-
- Journal:
- Advances in Applied Probability / Volume 49 / Issue 4 / December 2017
- Published online by Cambridge University Press:
- 17 November 2017, pp. 981-1010
- Print publication:
- December 2017
-
- Article
- Export citation
Moderate deviations for a Curie–Weiss model with dynamical external field∗
-
- Journal:
- ESAIM: Probability and Statistics / Volume 17 / 2013
- Published online by Cambridge University Press:
- 04 November 2013, pp. 725-739
- Print publication:
- 2013
-
- Article
- Export citation
Tail approximations for samples from a finite population withapplications to permutation tests
-
- Journal:
- ESAIM: Probability and Statistics / Volume 16 / 2012
- Published online by Cambridge University Press:
- 31 August 2012, pp. 425-435
- Print publication:
- 2012
-
- Article
- Export citation
Moderate Deviations for Word Counts in Biological Sequences
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 46 / Issue 4 / December 2009
- Published online by Cambridge University Press:
- 14 July 2016, pp. 1020-1037
- Print publication:
- December 2009
-
- Article
-
- You have access
- Export citation
Capacity bounds for the CDMA system and a neural network:a moderate deviations approach
-
- Journal:
- ESAIM: Probability and Statistics / Volume 13 / July 2009
- Published online by Cambridge University Press:
- 21 July 2009, pp. 343-362
- Print publication:
- July 2009
-
- Article
- Export citation
Process level moderate deviations for stabilizing functionals
-
- Journal:
- ESAIM: Probability and Statistics / Volume 14 / 2010
- Published online by Cambridge University Press:
- 07 October 2008, pp. 1-15
- Print publication:
- 2010
-
- Article
- Export citation
Functional Large Deviations and Moderate Deviations for Markov-Modulated Risk Models with Reinsurance
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 45 / Issue 3 / September 2008
- Published online by Cambridge University Press:
- 14 July 2016, pp. 800-817
- Print publication:
- September 2008
-
- Article
-
- You have access
- Export citation
Minimum variance importance sampling via Population Monte Carlo
-
- Journal:
- ESAIM: Probability and Statistics / Volume 11 / June 2007
- Published online by Cambridge University Press:
- 17 August 2007, pp. 427-447
- Print publication:
- June 2007
-
- Article
- Export citation
Large deviation properties of constant rate data streams sharing a buffer with long-range dependent traffic in critical loading
- Part of
-
- Journal:
- Advances in Applied Probability / Volume 39 / Issue 2 / June 2007
- Published online by Cambridge University Press:
- 01 July 2016, pp. 407-428
- Print publication:
- June 2007
-
- Article
-
- You have access
- Export citation
Moderate Deviations for I.I.D. Random Variables
-
- Journal:
- ESAIM: Probability and Statistics / Volume 7 / March 2003
- Published online by Cambridge University Press:
- 15 May 2003, pp. 209-218
- Print publication:
- March 2003
-
- Article
- Export citation
Bayesian inference for Markov chains
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 39 / Issue 1 / March 2002
- Published online by Cambridge University Press:
- 14 July 2016, pp. 91-99
- Print publication:
- March 2002
-
- Article
- Export citation