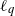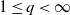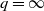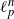Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Sonnleitner, Mathias
and
Thäle, Christoph
2025.
A note on critical intersections of classical and Schatten p-balls.
Random Matrices: Theory and Applications,
Vol. 14,
Issue. 02,
Kabluchko, Zakhar
Prochno, Joscha
and
Sonnleitner, Mathias
2025.
A probabilistic approach to Lorentz balls ℓq,1n.
Journal of Functional Analysis,
Vol. 288,
Issue. 1,
p.
110682.