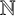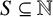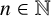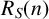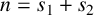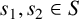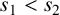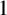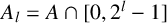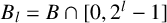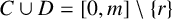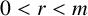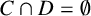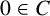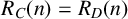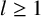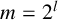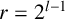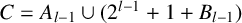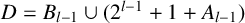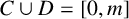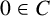Let
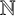 $\mathbb {N}$
be the set of all nonnegative integers. For
$\mathbb {N}$
be the set of all nonnegative integers. For
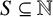 $S\subseteq \mathbb {N}$
and
$S\subseteq \mathbb {N}$
and
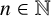 $n\in \mathbb {N}$
, let
$n\in \mathbb {N}$
, let
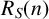 $R_S(n)$
denote the number of solutions of the equation
$R_S(n)$
denote the number of solutions of the equation
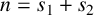 $n=s_1+s_2$
,
$n=s_1+s_2$
,
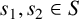 $s_1,s_2\in S$
and
$s_1,s_2\in S$
and
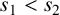 $s_1<s_2$
. Let A be the set of all nonnegative integers which contain an even number of digits
$s_1<s_2$
. Let A be the set of all nonnegative integers which contain an even number of digits
 $1$
in their binary representations and
$1$
in their binary representations and
 $B=\mathbb {N}\setminus A$
. Put
$B=\mathbb {N}\setminus A$
. Put
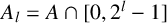 $A_l=A\cap [0,2^l-1]$
and
$A_l=A\cap [0,2^l-1]$
and
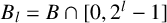 $B_l=B\cap [0,2^l-1]$
. We prove that if
$B_l=B\cap [0,2^l-1]$
. We prove that if
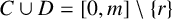 $C \cup D=[0, m]\setminus \{r\}$
with
$C \cup D=[0, m]\setminus \{r\}$
with
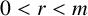 $0<r<m$
,
$0<r<m$
,
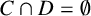 $C \cap D=\emptyset $
and
$C \cap D=\emptyset $
and
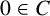 $0 \in C$
, then
$0 \in C$
, then
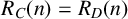 $R_{C}(n)=R_{D}(n)$
for any nonnegative integer n if and only if there exists an integer
$R_{C}(n)=R_{D}(n)$
for any nonnegative integer n if and only if there exists an integer
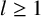 $l \geq 1$
such that
$l \geq 1$
such that
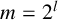 $m=2^{l}$
,
$m=2^{l}$
,
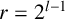 $r=2^{l-1}$
,
$r=2^{l-1}$
,
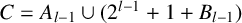 $C=A_{l-1} \cup (2^{l-1}+1+B_{l-1})$
and
$C=A_{l-1} \cup (2^{l-1}+1+B_{l-1})$
and
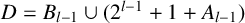 $D=B_{l-1} \cup (2^{l-1}+1+A_{l-1})$
. Kiss and Sándor [‘Partitions of the set of nonnegative integers with the same representation functions’, Discrete Math. 340 (2017), 1154–1161] proved an analogous result when
$D=B_{l-1} \cup (2^{l-1}+1+A_{l-1})$
. Kiss and Sándor [‘Partitions of the set of nonnegative integers with the same representation functions’, Discrete Math. 340 (2017), 1154–1161] proved an analogous result when
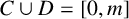 $C\cup D=[0,m]$
,
$C\cup D=[0,m]$
,
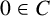 $0\in C$
and
$0\in C$
and
 $C\cap D=\{r\}$
.
$C\cap D=\{r\}$
.