We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We introduce “braided” versions of self-similar groups and Röver–Nekrashevych groups, and study their finiteness properties. This generalizes work of Aroca and Cumplido, and the first author and Wu, who considered the case when the self-similar groups are what we call “self-identical.” In particular, we use a braided version of the Grigorchuk group to construct a new group called the “braided Röver group,” which we prove is of type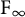 
$\operatorname {\mathrm {F}}_\infty $. Our techniques involve using so-called d-ary cloning systems to construct the groups, and analyzing certain complexes of embedded disks in a surface to understand their finiteness properties.
