We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
In this paper, we study both the existence and uniqueness of nonnegative solutions for the nonlocal 
$p$-Laplace equation with singular term where 
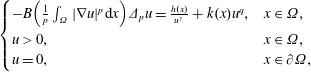$$\begin{eqnarray}\left\{\begin{array}{@{}ll@{}}-B\Bigl(\frac{1}{p}\int _{\unicode[STIX]{x1D6FA}}|\unicode[STIX]{x1D6FB}u|^{p}\text{d}x\Bigr)\unicode[STIX]{x1D6E5}_{p}u=\frac{h(x)}{u^{\unicode[STIX]{x1D6FE}}}+k(x)u^{q},\quad & x\in \unicode[STIX]{x1D6FA},\\ u>0,\quad & x\in \unicode[STIX]{x1D6FA},\\ u=0,\quad & x\in \unicode[STIX]{x2202}\unicode[STIX]{x1D6FA},\end{array}\right.\end{eqnarray}$$
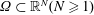$\unicode[STIX]{x1D6FA}\subset \mathbb{R}^{N}(N\geqslant 1)$ is a bounded domain with smooth boundary 
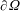$\unicode[STIX]{x2202}\unicode[STIX]{x1D6FA}$, 
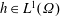$h\in L^{1}(\unicode[STIX]{x1D6FA})$, 
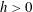$h>0$ almost everywhere in 
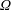$\unicode[STIX]{x1D6FA}$, 
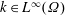$k\in L^{\infty }(\unicode[STIX]{x1D6FA})$ is a non-negative function, 
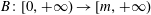$B:[0,+\infty )\rightarrow [m,+\infty )$ is continuous for some positive constant 
$m$, 
$p>1$,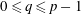 
$0\leqslant q\leqslant p-1$, and 
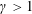$\unicode[STIX]{x1D6FE}>1$. A “compatibility condition” on the couple 
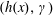$(h(x),\unicode[STIX]{x1D6FE})$ will be given for the problem to admit at least one solution. To be a little more precise, it is shown that the problem admits at least one solution if and only if there exists a 
$u_{0}\in W_{0}^{1,p}(\unicode[STIX]{x1D6FA})$ such that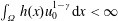 
$\int _{\unicode[STIX]{x1D6FA}}h(x)u_{0}^{1-\unicode[STIX]{x1D6FE}}\text{d}x<\infty$. When 
$k(x)\equiv 0$, the weak solution is unique.
