1 results
Normalized solutions for nonlinear Schrödinger systems
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 148 / Issue 2 / April 2018
- Published online by Cambridge University Press:
- 20 November 2017, pp. 225-242
- Print publication:
- April 2018
-
- Article
- Export citation
-
We consider the existence of normalized solutions in H1(ℝN) × H1(ℝN) for systems of nonlinear Schr¨odinger equations, which appear in models for binary mixtures of ultracold quantum gases. Making a solitary wave ansatz, one is led to coupled systems of elliptic equations of the form
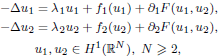
and we are looking for solutions satisfying
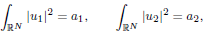
where a1> 0 and a2> 0 are prescribed. In the system, λ1 and λ2 are unknown and will appear as Lagrange multipliers. We treat the case of homogeneous nonlinearities, i.e.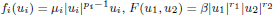
, with positive constants β, μi, pi, ri. The exponents are Sobolev subcritical but may be L2-supercritical. Our main result deals with the case in which 
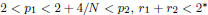in dimensions 2 ≤ N ≤ 4. We also consider the cases in which all of these numbers are less than 2 + 4/N or all are bigger than 2 + 4/N.
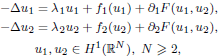
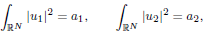
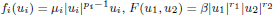, with positive constants 
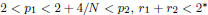in dimensions 2 ≤ 