Hostname: page-component-78c5997874-xbtfd Total loading time: 0 Render date: 2024-11-10T13:34:58.257Z Has data issue: false hasContentIssue false
  • English
  • Français

Normalized solutions for nonlinear Schrödinger systems

Part of: Elliptic equations and systems Equations and inequalities involving nonlinear operators Spectral theory and eigenvalue problems Partial differential equations Variational problems in infinite-dimensional spaces Equations of mathematical physics and other areas of application

Published online by Cambridge University Press:  20 November 2017

Thomas Bartsch  and
Louis Jeanjean
Thomas Bartsch
Affiliation:
Mathematisches Institut, Universität Giessen, Arndtstraße 2, 35392 Giessen, Germany (thomas.bartsch@math.uni-giessen.de)
Louis Jeanjean
Affiliation:
Laboratoire de Mathématiques (UMR 6623), Université Bourgogne Franche-Comté, 16 Route de Gray, 25030 Besançon Cedex, France (louis.jeanjean@univ-fcomte.fr)
Get access Rights & Permissions [Opens in a new window]

Abstract

We consider the existence of normalized solutions in H1(ℝN) × H1(ℝN) for systems of nonlinear Schr¨odinger equations, which appear in models for binary mixtures of ultracold quantum gases. Making a solitary wave ansatz, one is led to coupled systems of elliptic equations of the form

and we are looking for solutions satisfying

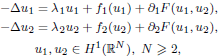
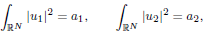
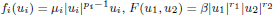where a1> 0 and a2> 0 are prescribed. In the system, λ1 and λ2 are unknown and will appear as Lagrange multipliers. We treat the case of homogeneous nonlinearities, i.e. , with positive constants β, μi, pi, ri. The exponents are Sobolev subcritical but may be L2-supercritical. Our main result deals with the case in which 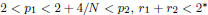in dimensions 2 ≤ N ≤ 4. We also consider the cases in which all of these numbers are less than 2 + 4/N or all are bigger than 2 + 4/N.

Keywords

nonlinear Schrödinger systemssolitary wavesnormalized solutionsvariational methodsconstrained linking

MSC classification

Secondary: 35J50: Variational methods for elliptic systems 35B08: Entire solutions 35J47: Second-order elliptic systems 35P30: Nonlinear eigenvalue problems, nonlinear spectral theory 35Q55: NLS-like equations (nonlinear Schrödinger) 47J30: Variational methods 58E05: Abstract critical point theory (Morse theory, Ljusternik-Schnirelman (Lyusternik-Shnirel'man) theory, etc.)
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 148 , Issue 2 , April 2018 , pp. 225 - 242
Copyright
Copyright © Royal Society of Edinburgh 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)