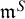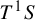Recall that two geodesics in a negatively curved surface S are of the same type if their free homotopy classes differ by a homeomorphism of the surface. In this note we study the distribution in the unit tangent bundle of the geodesics of fixed type, proving that they are asymptotically equidistributed with respect to a certain measure ${\mathfrak {m}}^S$ on $T^1S$ . We study a few properties of this measure, showing for example that it distinguishes between hyperbolic surfaces.