1 results
Représentations irréductibles bornées des groupes de Lie exponentiels
-
- Journal:
- Canadian Journal of Mathematics / Volume 53 / Issue 5 / 01 October 2001
- Published online by Cambridge University Press:
- 20 November 2018, pp. 944-978
- Print publication:
- 01 October 2001
-
- Article
-
- You have access
- Export citation
-
Let
$G$ be a solvable exponential Lie group. We characterize all the continuous topologically irreducible bounded representations
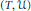$(T,\mathcal{U})$
of $G$ on a Banach space
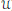$\mathcal{U}$
by giving a $G$-orbit i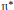n
${{n}^{*}}$
(
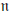$\mathfrak{n}$ being the nilradical of 
$\mathfrak{g}$), a topologically irreducible representation of
${{L}^{1}}({{\mathbb{R}}^{n}},\,\,\omega )$
, for a certain weight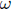 
$\omega $ and a certain 
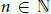$n\,\in \,\mathbb{N}$, and a topologically simple extension norm. If $G$ is not symmetric, i.e., if the weight 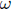$\omega $ is exponential, we get a new type of representations which are fundamentally different from the induced representations.
