Search

Let 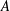$A$ be the inductive limit of a sequence

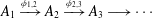$$\begin{eqnarray}A_{1}\xrightarrow[{}]{\unicode[STIX]{x1D719}_{1,2}}A_{2}\xrightarrow[{}]{\unicode[STIX]{x1D719}_{2,3}}A_{3}\longrightarrow \cdots\end{eqnarray}$$

with

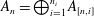$A_{n}=\bigoplus _{i=1}^{n_{i}}A_{[n,i]}$, where all the

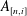$A_{[n,i]}$ are Elliott–Thomsen algebras and

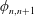$\unicode[STIX]{x1D719}_{n,n+1}$ are homomorphisms. In this paper, we will prove that

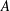$A$ can be written as another inductive limit

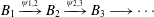$$\begin{eqnarray}B_{1}\xrightarrow[{}]{\unicode[STIX]{x1D713}_{1,2}}B_{2}\xrightarrow[{}]{\unicode[STIX]{x1D713}_{2,3}}B_{3}\longrightarrow \cdots\end{eqnarray}$$

with

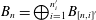$B_{n}=\bigoplus _{i=1}^{n_{i}^{\prime }}B_{[n,i]^{\prime }}$, where all the

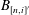$B_{[n,i]^{\prime }}$ are Elliott–Thomsen algebras and with the extra condition that all the

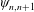$\unicode[STIX]{x1D713}_{n,n+1}$ are injective.

For two modules M and N, iM (N) stands for the largest submodule of N relative to which M is injective. For any module M, iM : Mod-R → Mod-R thus defines a left exact preradical, and iM (M) is quasi-injective. Classes of ring including strongly prime, semi-Artinian rings and those with no middle class are characterized using this functor: a ring R is semi-simple or right strongly prime if and only if for any right R-module M, iM (R) = R or 0, extending a result of Rubin; R is a right QI-ring if and only if R has the ascending chain condition (a.c.c.) on essential right ideals and iM is a radical for each M ∈ Mod-R (the a.c.c. is not redundant), extending a partial answer of Dauns and Zhou to a long-standing open problem. Also discussed are rings close to those with no middle class.

Search Results

Refine search

Refine search

Actions for selected content:

2 results

Injectivity of the Connecting Homomorphisms in Inductive Limits of Elliott–Thomsen Algebras

Rings Characterized via a Class of Left Exact Preradicals

Search Results

Refine search

Refine search

Actions for selected content:

Save Search

2 results

Injectivity of the Connecting Homomorphisms in Inductive Limits of Elliott–Thomsen Algebras

Rings Characterized via a Class of Left Exact Preradicals