We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
Tachikawa's second conjecture for symmetric algebras is shown to be equivalent to indecomposable symmetric algebras not having any nontrivial stratifying ideals. The conjecture is also shown to be equivalent to the supremum of stratified ratios being less than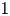 
$1$, when taken over all indecomposable symmetric algebras. An explicit construction provides a series of counterexamples to Tachikawa's second conjecture from each (potentially existing) gendo-symmetric algebra that is a counterexample to Nakayama's conjecture. The results are based on establishing recollements of derived categories and on constructing new series of algebras.
