Let $\mathcal{E}$ be an ample vector bundle of rank $r$ on a projective variety $X$ with only log-terminal singularities. We consider the nefness of adjoint divisors ${{K}_{X}}\,+\,\left( t-r \right)\,\det \,\mathcal{E}$ when $t\,\ge \,\dim\,X$ and $t\,>\,r$. As an application, we classify pairs $\left( X,\,\mathcal{E} \right)$ with ${{c}_{r}}$-sectional genus zero.

Let $X$ be a smooth projective surface over the complex number field and let $L$ be a nef-big divisor on $X$. Here we consider the following conjecture; If the Kodaira dimension 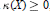$\kappa (X)\ge 0$, then 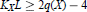${{K}_{X}}L\,\ge \,2q(X)\,-\,4$, where 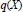$q\left( X \right)$ is the irregularity of $X$. In this paper, we prove that this conjecture is true if (1) the case in which 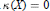$\kappa (X)=0$ or 1, (2) the case in which $\kappa (X)=2$ and ${{h}^{0}}(L)\,\ge \,2$ , or (3) the case in which 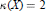$\kappa (X)=2$, $X$ is minimal, 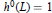${{h}^{0}}(L)\,=\,1$ , and $L$ satisfies some conditions.