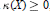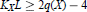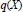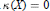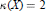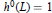Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Fukuma, Yoshiaki
and
Ishihara, Hironobu
2000.
A generalization of curve genus for ample vector bundles, II.
Pacific Journal of Mathematics,
Vol. 193,
Issue. 2,
p.
307.
Fukuma, Yoshiaki
2000.
On complexn-folds polarized by an ample line bundle L with ..
Communications in Algebra,
Vol. 28,
Issue. 12,
p.
5769.
Fukuma, Yoshiaki
2000.
On sectional genus of quasi-polarized manifolds with non-negative Kodaira dimension. II..
Kodai Mathematical Journal,
Vol. 23,
Issue. 1,
Fukuma, Yoshiaki
2001.
A Lower Bound for (KX+tL)Ln−1 of Quasi-Polarized Manifolds (X,L) with κ(KX+tL)≥0.
Journal of Algebra,
Vol. 239,
Issue. 2,
p.
624.
FUKUMA, Yoshiaki
2002.
A LOWER BOUND FOR KXL2 OF POLARIZED 3-FOLDS (X,L) OF GENERAL TYPE.
Kyushu Journal of Mathematics,
Vol. 56,
Issue. 1,
p.
203.