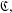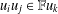Refine search
Actions for selected content:
2 results
An uncountable Furstenberg–Zimmer structure theory
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 43 / Issue 7 / July 2023
- Published online by Cambridge University Press:
- 21 June 2022, pp. 2404-2436
- Print publication:
- July 2023
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
(ANTI)COMMUTATIVE ALGEBRAS WITH A MULTIPLICATIVE BASIS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 91 / Issue 2 / April 2015
- Published online by Cambridge University Press:
- 15 December 2014, pp. 211-218
- Print publication:
- April 2015
-
- Article
-
- You have access
- Export citation