In this paper, we introduce the concept of A-proper topological degree in Menger PN-space and study some of its properties. Utilising its properties, we obtain a new fixed point theorem.

In the paper “The topological degree of A-proper mapping in the Menger PN-space (I)”, the new concept of A-proper topological degree has been given. Now, utilising the new concept, we give the corresponding definitions of convex A-proper, P*-compact and Pγ-compact in Menger PN-space. As an application of these new concepts, we prove the existence of solution for some equations.

In this paper, we study the existence of positive periodic solutions to the equation x″ = f (t, x). It is proved that such a equation has more than one positive periodic solution when the nonlinearity changes sign. The proof relies on a fixed point theorem in cones.

In this paper we give a complete classification of -invariant or Hopf pseudo-Einstein real hypersurfaces in complex two-plane Grassmannians G2(ℂm+2).

In the paper the asymptotic distribution of (|ζ(s)|,ζ(s)), where ζ(s) is the Riemann zeta - function, in the sense of weak convergence of probability measures is considered. For this, the continuity theorems for probability measures on ℝ × ℂ are used. Some aspects of the dependence of |ζ(s)| and ζ(s) are also discussed.

In 1996 Poland and Rhemtulla proved that the number v (G) of conjugacy classes of non-normal subgroups of a non-Hamiltonian nilpotent group G is at least c − 1, where c is the nilpotency class of G. In this paper we consider the map that associates to every conjugacy class of subgroups of a finite p-group the conjugacy class of the normaliser of any of its representatives. In spite of the fact that this map need not be injective, we prove that, for p odd, the number of conjugacy classes of normalisers in a finite p-group is at least c (taking into account the normaliser of the normal subgroups). In the case of p-groups of maximal class we can find a better lower bound that depends also on the prime p.

In this article using techniques which appeared in Witt's proof of Wedderburn's theorem, an arithmetical characterisation of groups acting on a set whose orbits are all singleton is given. This can then be used to obtain a new proof of Wedderburn's theorem.

We shall discuss boundedness and compactness of the products of composition and differentiation between Hardy spaces.

The purpose of this article is to construct families of elliptic curves E over finite fields F so that the groups of F-rational points of E are cyclic, by using a representation of the modular invariant function by a generator of a modular function field associated with the modular group Γ0(N), where N = 5, 7 or 13.

Some elementary inequalities providing upper bounds for the difference of the norm and the numerical radius of a bounded linear operator on Hilbert spaces under appropriate conditions are given.

We introduce a class of β − v-unfavourable spaces, which contains some known classes of β-unfavourable spaces for topological games of Choquet type. It is proved that every β − v-unfavourable space X is a Namioka space, that is for any compact space Y and any separately continuous function f : x × Y → ℝ there exists a dense in XGδ-set A ⊆ X such that f is jointly continuous at each point of A × Y.

We construct a family of representations of an arbitrary variant Sa of a semigroup S, induced by a given representation of S, and investigate properties of such representations and their kernels.

We use exponential sums to obtain new lower bounds on the number of distinct distances defined by all pairs of points (a, b) ∈ A × B for two given sets where is a finite field of q elements and n ≥ 1 is an integer.

In this paper, we refine a theorem of F. Wang and G. Tang concerning freeness of reflexive modules over coherent GCD-domains.

It is shown that if a retarded delay differential equation has a global attractor 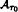in the space C ([—τ0, ], ℝd) for a given nonzero constant delay τ0, then the equation has an attractor Aτ in the space C ([—τ, 0], ℝd) for nearby constant delays τ. Moreover the attractors Aτ converge upper semi continuously to 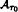in C ([—τ0, 0], ℝd) in the sense that they are identified through corresponding segments of entire trajectories in ℝd with nonempty compact subsets 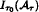of C ([—τ0, 0], ℝd) which converge upper semi continuously to 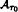in C ([—τ0, 0], ℝd).

In this paper, we introduce a new concept of generalised f-projection operator which extends the generalised projection operator πK : B* → K, where B is a reflexive Banach space with dual space B* and K is a nonempty, closed and convex subset of B. Some properties of the generalised f-projection operator are given. As an application, we study the existence of solution for a class of variational inequalities in Banach spaces.