In this paper we generalise the concept of a Steinberg cross section to non-connected affine Kac–Moody groups. This Steinberg cross section is a section to the restriction of the adjoint quotient map to a given exterior connected component of the affine Kac–Moody group. (The adjoint quotient is only defined on a certain submonoid of the entire group, however, the intersection of this submonoid with each connected component is non-void.) The image of the Steinberg cross section consists of a “twisted Coxeter cell”, a transversal slice to a twisted Coxeter element. A crucial point in the proof of the main result is that the image of the cross section can be endowed with a ${{\mathbb{C}}^{*}}$-action.

The first eigenvalue of the Laplacian on a surface can be viewed as a functional on the space of Riemannian metrics of a given area. Critical points of this functional are called extremal metrics. The only known extremal metrics are a round sphere, a standard projective plane, a Clifford torus and an equilateral torus. We construct an extremal metric on a Klein bottle. It is a metric of revolution, admitting a minimal isometric embedding into a sphere ${{\mathbb{S}}^{4}}$ by the first eigenfunctions. Also, this Klein bottle is a bipolar surface for Lawson's ${{\tau }_{3,1}}$-torus. We conjecture that an extremal metric for the first eigenvalue on a Klein bottle is unique, and hence it provides a sharp upper bound for ${{\lambda }_{1}}$ on a Klein bottle of a given area. We present numerical evidence and prove the first results towards this conjecture.

The aim of this paper is to extend the structure theory for infinitely generated modules over tame hereditary algebras to the more general case of modules over concealed canonical algebras. Using tilting, we may assume that we deal with canonical algebras. The investigation is centered around the generic and the Prüfer modules, and how other modules are determined by these modules.

Selick and Wu gave a functorial decomposition of $\Omega \Sigma X$ for path-connected, $p$-local $CW$-complexes $X$ which obtained the smallest nontrivial functorial retract ${{A}^{\min }}\left( X \right)$ of $\Omega \Sigma X$. This paper uses methods developed by the second author in order to extend such functorial decompositions to the loops on coassociative co-$H$ spaces.

The equality of the centralizer and twisted centralizer is proved based on a case-by-case analysis when the unipotent radical of a maximal parabolic subgroup is abelian. Then this result is used to determine the poles of intertwining operators.

The following problem has been suggested by Paul Turán. Let $\Omega $ be a symmetric convex body in the Euclidean space 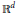${{\mathbb{R}}^{d}}$ or in the torus 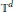${{\mathbb{T}}^{d}}$. Then, what is the largest possible value of the integral of positive definite functions that are supported in $\Omega $ and normalized with the value 1 at the origin? From this, Arestov, Berdysheva and Berens arrived at the analogous pointwise extremal problem for intervals in 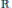$\mathbb{R}$. That is, under the same conditions and normalizations, the supremum of possible function values at $z$ is to be found for any given point 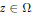$z\,\in \,\Omega $. However, it turns out that the problem for the real line has already been solved by Boas and Kac, who gave several proofs and also mentioned possible extensions to 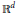${{\mathbb{R}}^{d}}$ and to non-convex domains as well.

Here we present another approach to the problem, giving the solution in 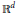${{\mathbb{R}}^{d}}$ and for several cases in 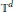${{\mathbb{T}}^{d}}$. Actually, we elaborate on the fact that the problem is essentially one-dimensional and investigate non-convex open domains as well. We show that the extremal problems are equivalent to some more familiar ones concerning trigonometric polynomials, and thus find the extremal values for a few cases. An analysis of the relationship between the problem for 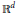${{\mathbb{R}}^{d}}$ and that for 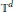${{\mathbb{T}}^{d}}$ is given, showing that the former case is just the limiting case of the latter. Thus the hierarchy of difficulty is established, so that extremal problems for trigonometric polynomials gain renewed recognition.

We introduce a new conjecture concerning the construction of elements in the annihilator ideal associated to a Galois action on the higher-dimensional algebraic $K$-groups of rings of integers in number fields. Our conjecture ismotivic in the sense that it involves the (transcendental) Borel regulator as well as being related to $l$–adic étale cohomology. In addition, the conjecture generalises the wellknown Coates–Sinnott conjecture. For example, for a totally real extension when $r\,=\,-2,\,-4,\,-6,\,\ldots $ the Coates–Sinnott conjecture merely predicts that zero annihilates ${{K}_{-2r}}$ of the ring of $S$–integers while our conjecture predicts a non-trivial annihilator. By way of supporting evidence, we prove the corresponding (conjecturally equivalent) conjecture for the Galois action on the étale cohomology of the cyclotomic extensions of the rationals.