Hostname: page-component-78c5997874-s2hrs Total loading time: 0 Render date: 2024-11-14T08:09:41.645Z Has data issue: false hasContentIssue false
  • English
  • Français

Large discrete parts of the E-tree

Published online by Cambridge University Press:  12 March 2014

Harold Simmons
Harold Simmons*
Affiliation:
Department of Mathematics, University of Aberdeen, Aberdeen, Scotland
Get access Rights & Permissions [Opens in a new window]

Extract

Let PA be first order Peano arithmetic, let Λ be the lattice of Π1 sentences modulo PA, and let S be the poset of prime filters of Λ ordered by reverse inclusion. We show there are large convex discrete parts of S; in particular there are convex parts which form a completed Baire tree or an Aronszajn tree.

The elements of S, which we call nodes, correspond to the extensions of PA which are complete for 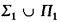sentences. Equivalently, for each model of PA the Π1-theory ∀() of is a node, and every node occurs in this form. Note that the Π1-theory ∀(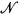) of the standard model 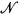(i.e. the filter of true Π1 sentences) is the unique root of S.

This poset S, which is sometimes called the E-tree, was first studied in [1] where it is shown that:

(1) The poset is tree-like, i.e. the set of predecessors of any node is linearly ordered.

(2) The poset has branches, each of which is closed under unions and intersections; in particular each branch has a maximum member.

(3) There are branches on which ∀(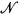) does not have an immediate successor. Further properties of the E-tree are given in [2]−[7]. In particular in [4] Misercque shows that:

(4) There are branches on which ∀(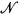) does have an immediate successor.

(5) There are nodes with both an immediate predecessor and an immediate successor.

The two results (3) and (4) show that there are fundamentally different branches of S, and (5) shows that parts of branches may be discrete.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 53 , Issue 3 , September 1988 , pp. 980 - 984
Copyright
Copyright © Association for Symbolic Logic 1988

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Jensen, D. and Ehrenfeucht, A., Some problem in elementary arithmetics, Fundamenta Mathematkae, vol. 92 (1976), pp. 223–245.CrossRefGoogle Scholar
[2]Misercque, D., Solution de deux problèmes posés par H. Simmons, Bulletin de la Société Mathématique de Belgique, Série B, vol. 33 (1981), pp. 65–72.Google Scholar
[3]Misercque, D., The nonhomogeneity of the E-tree—answer to a problem raised by D. Jensen and A. Ehrenfeucht, Proceedings of the American Mathematical Society, vol. 84 (1982), pp. 573–575.Google Scholar
[4]Misercque, D., Branches of the E-tree which are not isomorphic, Bulletin of the London Mathematical Society, vol. 17 (1985), pp. 513–517.CrossRefGoogle Scholar
[5]Misercque, D., Sur le treillis de formules fermes universelles de l'arithmétique de Peano, Thesis, Université Libre de Bruxelles, Brussels, 1985.Google Scholar
[6]Simmons, H., Existentially closed models of basic number theory. Logic Colloquium '76, North-Holland, Amsterdam, 1977, pp. 325–369.Google Scholar
[7]Simmons, H., The lattice of universal sentences modulo Peano arithmetic, Rapport du Séminaire de Mathématique Pure, no. 93, Université Catholique de Louvain, Louvain-La-Neuve, 1980.Google Scholar
[8]Smoryński, C., Self-reference and modal logic, Springer-Verlag, Berlin, 1985.CrossRefGoogle Scholar