In (1) the authors introduced the idea of a syntopogenous pre-ordered space and, in (2), discussed the more general notion of a convex syntopogenous space. The former generalizes Nachbin's ‘uniform preordered space’ (4) and Singal and Lal's ‘proximity preordered space’ (7); the latter does the same for Nachbin's ‘convex topological space’ (4) and Redfield's ‘nearly uniform ordered space’ (5).

Consider an ideal A of a commutative ring R; denote by Ai the integral closure of A in R.

This paper is concerned with the notion of independence relating sets of elements in a ring A to a proper ideal a of A. A set of elements a1, …, an ∈A is called a-independent if every form in A[X1, …, Xn] vanishing at a1,…, an has all its coefficients in a. This notion leads to many questions (cf. (2) and (12)), which are of some interest in their own right, several of which are considered here. On the other hand, this independence is related to the structure of the graded ring associated to the ideal generated by the set of elements, hence is often relevant to some problems concerning regular sequences and complex.

An important problem in geometric topology is to find a homology 3-sphere M with Rohlin invariant μ(M) = ½ such that M # M bounds an acyclic 4-manifold. If such an M exists then, for instance, all closed topological manifolds of dimension ≥ 5 support a polyhedral structure(3, 4). One way of producing such examples is to find a homology 3-sphere M with μ(M) = ½ such that M admits an orientation-reversing diffeomorphism h, for then M # M would be diffeomorphic to M # − M which bounds an acyclic manifold. In this paper we observe by elementary means that if h is further assumed to be an involution, then this is not possible, namely:

Theorem. Let M be a homology 3-sphere which admits an orientation reversing involution. Then M bounds a parallelizable rational 4-ball. In particular, μ(M) = 0.

We establish integral representations for quotients of Tricomi ψ functions and of several quotients of modified Bessel functions and of linear combinations of them. These integral representations are used to prove the complete monotonicity of the functions considered and to prove the infinite divisibility of a three parameter probability distribution. Limiting cases of this distribution are the hitting time distributions considered recently by Kent and Wendel. We also derive explicit formulas for the Kent–Wendel probability density functions.

Let H(μ, θ) be the hyperplane in Rn (n ≥ 2) that is perpendicular to the unit vector 6 and perpendicular distance μ from the origin; that is, H(μ, θ) = (x ∈ Rn: x. θ = μ). (Note that H(μ, θ) and H(−μ, −θ) are the same hyperplanes.) Let X be a proper compact convex subset of Rm. If f(x) ∈ L1(X) we will denote by F(μ, θ) the projection of f perpendicular to θ; that is, the integral of f(x) over H(μ, θ) with respect to (n − 1)-dimensional Lebesgue measure. By Fubini's Theorem, if f(x) ∈ L1(X), F(μ, θ) exists for almost all μ for every θ. Our aim in this paper is, given a finite collection of unit vectors θ1, …, θN, to characterize the F(μ, θi) that are the projections of some function f(x) with support in X for 1 ≤ i ≤ N.

Let AB be the algebra of all bounded continuous functions on the closed unit ball B of c0, analytic on the open unit ball, with sup norm, and let AU be the sub-algebra of AB of those functions which are uniformly continuous on B. Call a set S ⊂ B a boundary of AB (AU) if

for every f ∈ AB (f ∈AU, respectively). In the paper we study the boundaries of AB and AU. We give a complete description of the boundaries of AU and present some necessary and some sufficient conditions for a set to be a boundary of AB. We also give some examples of boundaries.

Throughout this paper α denotes a number larger than 1 and C denotes the complex plane. Pó1ya(15),(16) (see also Hardy(10) and Landau(13)) proved the following results.

1. There has been much interest recently in ‘instantons’. These correspond, in differential geometric terms, to connexions in principal bundles over Euclidean 4-space whose curvature satisfies the Yang-Mills equations. The connexion is also required to approach the trivial connexion at infinity. The self-dual solutions have been classified by converting the problem into one of algebraic geometry using the ideas of R. Penrose and R. Ward

Let X be a Hausdorff topological space. We consider various locally convex spaces of continuous real valued functions on X and give necessary and sufficient conditions in order that (i) they contain an absolutely convex weakly compact total subset and (ii) they contain an absolutely convex total subset which is an Eberlein compact, when given the weak topology.

Background. Riesz products are very useful for the construction of singular measures on compact, Abelian groups. Under some circumstances, two Riesz products are either equivalent or singular in the measure-theoretic sense. Exact knowledge of these circumstances has been of major interest ever since the 1930s, when Riesz's famous example (8) was recognized as a fertile source of examples of singular continuous measures. Zygmund(11) showed that any Riesz product over a Hadamard dissociate subset of ℕ is either a square integrable function or singular with respect to Lebesgue measure. Hewitt–Zuckerman(4) generalized these products to all compact, Abelian groups, introducing the notion of a dissociate subset. They extended Zygmund's result in certain cases. The next major step was taken by Brown–Moran(3) and Peyrière(6), (7), who showed that two Riesz products

are mutually singular if

The author (9) has improved another result of Brown–Moran (3) by showing that µa and µb are equivalent if

By a dynamical system we mean one of several related objects: measure preserving transformations on probability spaces (processes), self homeomorphisms of compact metric spaces (compact systems), or a combination of these, namely compact systems provided with invariant Borel probability measures. It is the latter, which we call compact processes, which will be of most interest in this paper. In particular, we will study the dynamical properties of the product of two processes with respect to compatible measures – those measures which project to the given measures on the component spaces. This leads to the notion of disjointness of two processes – the only compatible measure is the product measure. As an application we obtain a theorem, a special case of which gives rise to a class of transformations which preserve normal sequences. Finally, we study a topological analog (topological disjointness) and briefly consider the relation between the two notions of disjointness.

Let S be a semigroup with a compact topology in which multiplication is continuous on the left (i.e. xi→x implies xiy→xy for each y in S). Then S has a minimal left ideal L which is compact; each idempotent e in L is a right identity for L (xe = xfor each x ∈ L)and L = Se; Ge = eL is a group and L is the union of all such groups; and if f is a second idempotent in L, the canonical map x ↦ fx of Ge to Gf is an algebraic isomorphism (see Ruppert(2) for these facts). Baker and Milnes(1), §4(A), have observed that, in the case in which S is the Stone–Cech compactification of a discrete abelian group, the canonical map from Ge to Gf may not be a homeomorphism. (This contrasts with the situation in compact semigroups with separately continuous multiplication.) We present a simple proof of a more definitive result.

A JB-algebra A is a real Jordan algebra, which is also a Banach space, the norm in which satisfies the conditions that

and

for all elements a and b in A. It follows from (1.1) and (l.2) that

for all elements a and b in A. When the JB-algebra A possesses an identity element then A is said to be a unital JB-algebra and (1.2) is equivalent to the condition that

for all elements a and b in A. For the general theory of JB-algebras the reader is referred to (2), (3), (7) and (10).

Approximate analytical expressions for the wave reflexion amplitude for transmission above one-dimensional real smooth and slowly varying potentials in the absence of classical turning points are obtained in two different ways by means of the phase-integral method developed by N. Fröman and P. O. Fröman. The wave reflexion is shown to be governed by the complex transition points for the problem. The great accuracy of the formulae obtained, as well as their respective ranges of applicability, is illustrated by applying them, up to the eleventh order phase-integral approximation, to an exactly solvable model, for which the potential represents a monotonic step.

In (13), Quillen defines a higher algebraic K-theory by taking homotopy groups of the classifying spaces of certain categories. Certain questions in K-theory then become questions such as when do functors induce a homotopy equivalence of classifying spaces, or when is a square of categories homotopy cartesian? Quillen has given some techniques for answering such questions. F. Waldhausen has extended these ideas in (19), and broadened the range of applications to include geometric topology (20).

In his paper (2), Ripley has shown that some of the basic results of point process theory can be established without making any use of topological assumptions concerning the phase space. For the construction of distributions of point processes he needs pseudo-topological assumptions. In the present paper, another sufficient condition is given for the fact that a distribution of a random measure can be constructed by help of finite-dimensional distributions. This condition is expressed by purely measure-theoretic notions. Roughly speaking, it is supposed that concerning the existence of conditional probabilities the phase space behaves as if it were a Polish space. Measureable spaces with such a property are called ‘full measurable spaces’; they are identical with the measurable spaces of type (B) in (1).

A circular disc of radius a, made of homogeneous, isotropic, linearly elastic material, contains a radial edge crack of length b(0 < b < 2a). The disc is in equilibrium in a state of generalized plane stress under various loadings, which are motivated by the fact that this geometry is to become a standard test specimen configuration in the fracture testing of materials.

The first cases considered are those in which the disc is loaded by either (i) opposing point forces P normal to the crack, or (ii) opposing point couples M, in each case acting at the crack mouth. The problem of determining the resulting stress field throughout the disc is solved analytically in closed form in each case, and the respective stress intensity factors 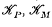are given exactly by

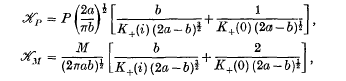
where K+(0), K+(i) are constants whose values are

K+(0) = 0.966528…,

K+(i) = 0.355715…,

correct to 6 decimal places.

Let T be a linear operator on a complex normed space X. Its spatial numerical range V(T) is denned as

Here we describe our results and their background: terminology (mostly standard) is denned in Section 2. Throughout, F is a separable Banach space, 1 ≤ p < ∞ and Lp(F) is the space of measurable functions [0,1] → F with P-integrable norms. Given a ‘nice’ property P for Banach spaces, we may formulate the conjecture: Lp(F) satisfies P if and only if both F and Lp (= LP(ℝ)) satisfy P. This conjecture is known to be true for various specific properties, for example the Radon–Nikodym property ((4), section 5·4); reflexivity ((4), corollary 4·1·2); super-refiexivity ((12), proposition 1·2); B-convexity ((14), p. 200); and the properties of not containing copies of c0 (6) and l1 (13). The object of this paper is to demonstrate that the conjecture is false for the property of having an unconditional basis – this answers a question in (4).