A random environment is modelled by an arbitrary stochastic process, the future of which is described by a σ-algebra. Renewal processes and alternating renewal processes are defined in this environment by considering the conditional distributions of random variables generated by the processes with respect to the σ-algebra. Generalizations of several of the standard limit theorems of renewal theory are derived.

This paper is a supplement to [2]. There, solutions ψ., (μ) of certain free-boundary problems, depending on a small parameter μ, are related to the Green function G., (a(μ)) of a second-order, elliptic, partial differential equation defined on a bounded set Ω ⊂ ℝ2. The free boundary is the boundary of a set A(μ) ⊂ Ω that is unknown a priori and corresponds to the cross-section of a steady vortex ring or of a confined plasma in equilibrium, for example.

Let Σ be a closed surface of genus ≥ 1, G a compact Lie group, not necessarily connected with Lie algebra g, ξ,: P → Σ a principal G-bundle, and suppose Σ equipped with a Riemannian metric and g with an invariant scalar product so that the Yang—Mills equations on ξ are defined. Further, let

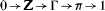
be the universal central extension of the fundamental group π of Σ and ΓR the group obtained from Γ when its centre Z is extended to the additive group R of the reals. We show that there are bijective correspondences between various spaces of classes of Yang—Mills connections on ξ and spaces of representations of Γ and ΓR (as appropriate) in G. In particular, we show that the holonomy establishes a homeomorphism between the moduli space N(ξ) of central Yang–Mills connections on ξ and the space Repξ(Γ, G) of representations of Γ in G determined by ξ. Our results rely on a detailed study of the holonomy of a central Yang–Mills connection and extend corresponding ones of Atiyah and Bott for the case where G is connected.

K. Motegi has kindly pointed out that the Lemma in Section 4 of the paper is incorrect. (The assertion in Case 1 on p. 95 that the sewing map of E(K) is periodic is false.) Hence Theorem 6 is unproven. However, we have a weaker result.

Some self-dual local rings of integers not free over their associated orders

By N. P. Byott

(Volume 110 (1991), 5–10)

Department of Mathematics, University of Exeter, Exeter EX4 4QE

(Received 19 August 1993; Revised 21 March 1994)