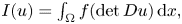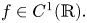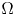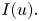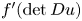On a class of special Euler–Lagrange equations

Abstract

We make some remarks on the Euler–Lagrange equation of energy functional $I(u)=\int _\Omega f(\det Du)\,{\rm d}x,$ where $f\in C^1(\mathbb {R}).$ For certain weak solutions $u$ we show that the function $f'(\det Du)$ must be a constant over the domain $\Omega$ and thus, when $f$ is convex, all such solutions are an energy minimizer of $I(u).$ However, other weak solutions exist such that $f'(\det Du)$ is not constant on $\Omega.$ We also prove some results concerning the homeomorphism solutions, non-quasimonotonicity and radial solutions, and finally we prove some stability results and discuss some related questions concerning certain approximate solutions in the 2-Dimensional cases.

1. Introduction

Let $n\ge 2$ be an integer. We denote by $\mathbb {M}^{n\times n}$ the standard space of real $n\times n$ matrices with inner product

\[ A:B =\sum_{i,j=1}^n A^i_j B^i_j \quad \forall\ A=(A^i_j), \ B=(B^i_j)\in \mathbb{M}^{n\times n}. \]

Also denote by $A^T$, $\det A$ and $\operatorname {cof} A$ the transpose, determinant, and the cofactor matrix of $A\in \mathbb {M}^{n\times n},$ respectively, so that the identity $A^T\operatorname {cof} A=(\operatorname {cof} A)A^T=(\det A)I$ holds for all $A\in \mathbb {M}^{n\times n}.$

Let $f\colon \mathbb {R}\to \mathbb {R}$ be a $C^1$ function; i.e., $f\in C^1(\mathbb {R}),$ and $\Omega$ be a bounded domain in $\mathbb {R}^n.$ We study the special energy functional

(1.1)\begin{equation} I_\Omega(u)=\int_\Omega f(\det Du){\rm d}x \end{equation}

for functions $u\colon \Omega \to \mathbb {R}^n,$ where $Du$ is the Jacobian matrix of $u$; that is, $(Du)^i_j=\partial u^i/\partial x_j$ if $u =(u^1,\, \dots,\,u^n)$ and $x=(x_1,\, \dots,\,x_n)\in \Omega.$ Such a functional $I_\Omega$ and its Euler–Lagrange equations have been studied in [3, 5, 8, 17] concerning the energy minimizers, weak solutions and the gradient flows. Our motivation to further study the functional $I_\Omega$ is closely related to the counterexamples on existence and regularity given in [13, 16, 20]. From the identity

\[ \partial \det A/\partial A^i_j=(\operatorname{cof} A)^i_j; \ \mbox{i.e.,} \ D(\det A) = \operatorname{cof} A \quad \forall\ A=(A^i_j) \in \mathbb{M}^{n\times n}, \]

it follows that the Euler–Lagrange equation of $I_\Omega$ is given by

(1.2)\begin{equation} \operatorname{div} (f'(\det Du)\operatorname{cof} Du)=0 \quad\mbox{in} \ \Omega. \end{equation}

By a weak solution of equation (1.2) we mean a function $u\in W^{1,1}_{loc}(\Omega ;\mathbb {R}^n)$ such that $f'(\det Du)\operatorname {cof} Du\in L^1_{loc}(\Omega ;\mathbb {M}^{n\times n})$ and

(1.3)\begin{equation} \int_\Omega f'(\det Du)\operatorname{cof} Du : D\zeta \,{\rm d}x =0 \quad \forall\ \zeta\in C_c^1(\Omega;\mathbb{R}^n). \end{equation}

This is a very weak sense of solutions. Usually, under suitable growth conditions on $f,$ the weak solutions are studied in the space $u\in W^{1,p}_{loc}(\Omega ;\mathbb {R}^n)$ for some $1\le p\le \infty$ with $f'(\det Du)\operatorname {cof} Du\in L^{p'}_{loc}(\Omega ;\mathbb {M}^{n\times n})$, where $p'= {p}/{(p-1)}$ is the Hölder conjugate of $p.$ However, in this paper, we will not specify the growth conditions on $f,$ but may assume some higher integrability of $f'(\det Du)\operatorname {cof} Du.$ For example, if a weak solution $u$ satisfies, in addition, $f'(\det Du)\operatorname {cof} Du\in L^q(\Omega ;\mathbb {M}^{n\times n})$ for some $q\ge 1$, then (1.3) holds for all $\zeta \in W^{1,q'}_0(\Omega ;\mathbb {R}^n).$

It is well-known that $\operatorname {div}(\operatorname {cof} Du)=0$ for all $u\in C^2(\Omega ;\mathbb {R}^n)$ and thus by approximation it holds that

(1.4)\begin{equation} \int_\Omega \operatorname{cof} Du : D\zeta \,{\rm d}x =0 \quad \forall\ u\in W^{1,n-1}_{loc}(\Omega;\mathbb{R}^n),\; \zeta\in C_c^1(\Omega;\mathbb{R}^n). \end{equation}

Consequently, any functions $u\in W^{1,n-1}_{loc}(\Omega ;\mathbb {R}^n)$ with $f'(\det Du)$ being constant almost everywhere on $\Omega$ are automatically a weak solution of equation (1.2); however, the converse is false, at least when $n=2$ (see example 3.4). Some formal calculations based on the identity $\operatorname {div}(\operatorname {cof} Du)=0$ show that $f'(\det Du)$ must be constant on $\Omega$ if $u\in C^2(\Omega ;\mathbb {R}^n)$ is a classical solution of (1.2) (see [5, 17]). We prove that the same result holds for all weak solutions $u\in C^1(\Omega ;\mathbb {R}^n)$ (see also [8]) or $u\in W^{1,p}_{loc}(\Omega ;\mathbb {R}^n)$ with $f'(\det Du)\in W_{loc}^{1,\bar p}(\Omega )$ for some $p\ge n-1$ and $\bar p=p/(p+1-n).$ If, in addition, $f$ is convex, then all weak solutions $u\in W^{1,n}_{loc}(\Omega ;\mathbb {R}^n)$ of (1.2) with $f'(\det Du)$ being constant almost everywhere on $\Omega$ must be an energy minimizer of the energy $I_G$ among all $W^{1,n}(G;\mathbb {R}^n)$ functions having the same boundary value as $u$ on $\partial G$ for all subdomains $G$ of $\Omega.$

We study further conditions under which a weak solution $u$ of (1.2) must have constant $f'(\det Du)$ almost everywhere on $\Omega.$ In addition, we prove that $f'(\det Du)$ must be constant in the case of homeomorphism weak solutions $u\in W^{1,p}(\Omega ;\mathbb {R}^n)$ for $p>n$ (see theorem 2.8). When the domain $\Omega$ is a ball, we study the radially symmetric weak solutions and establish a specific characterization about certain weak solutions (see § 3). Finally, in the 2-Dimensional cases, equation (1.2) is reformulated as a first-order partial differential relation [8, 13] and we prove some stability results for certain approximate solutions of this relation (see § 4).

2. Some general results for all dimensions

In what follows, unless otherwise specified, we assume $h\in C(\mathbb {R})$ is a given function. Recall that $u\in W^{1,1}_{loc}(\Omega ;\mathbb {R}^n)$ is a weak solution of equation

(2.1)\begin{equation} \operatorname{div} (h(\det Du)\operatorname{cof} Du)=0 \quad\mbox{in} \ \Omega, \end{equation}

provided that $h(\det Du)\operatorname {cof} Du\in L^1_{loc}(\Omega ;\mathbb {M}^{n\times n})$ and

(2.2)\begin{equation} \int_\Omega h(\det Du)\operatorname{cof} Du : D\zeta \,{\rm d}x =0 \quad \forall\, \zeta\in C_c^1(\Omega;\mathbb{R}^n). \end{equation}

If, in addition, $h(\det Du)\operatorname {cof} Du\in L_{loc}^q(\Omega ;\mathbb {M}^{n\times n})$ for some $q\ge 1,$ then equation (2.2) holds for all $\zeta \in W^{1,q'}(\Omega ;\mathbb {R}^n)$ with compact support in $\Omega,$ where $q'={q}/({q-1}).$ Since equation (2.1) and the related energy functional highly lack the ellipticity and coercivity, there is no feasible theory of existence and regularity for (2.1)

2.1. Formal calculations and some implications

It is well-known that $\operatorname {div}(\operatorname {cof} Dw)=0$ holds in $\Omega$ for all $w\in C^2(\Omega ;\mathbb {R}^n).$ From this, we easily have the following point-wise identity:

\[ \operatorname{div}(a \operatorname{cof} Dw )=(\operatorname{cof} Dw) Da \quad \forall\ a\in C^1(\Omega),\ w\in C^2(\Omega;\mathbb{R}^n). \]

Thus, for all $a\in C^1(\Omega ),\,\;w\in C^2(\Omega ;\mathbb {R}^n)$ and $\zeta \in C^1_c(\Omega ;\mathbb {R}^n),$ it follows that

(2.3)\begin{equation} \int_\Omega a \operatorname{cof} Dw :D\zeta\,{\rm d}x ={-}\int_\Omega (\operatorname{cof} Dw) Da\cdot \zeta\,{\rm d}x. \end{equation}

Lemma 2.1 Identity (2.3) holds for all functions

\[ w\in W^{1, p}_{loc}(\Omega;\mathbb{R}^n),\quad a\in W^{1,\bar p}_{loc}(\Omega;\mathbb{R}^n), \quad \zeta\in C_c^1(\Omega;\mathbb{R}^n), \]

where $n-1\le p\le \infty$ and $\bar p={p}/({p+1-n})$ are given numbers.

Proof. Note that if $w\in W^{1, p}_{loc}(\Omega ;\mathbb {R}^n)$ then $\operatorname {cof} Dw\in L^{{p}/({n-1})}_{loc}(\Omega ;\mathbb {M}^{n\times n}).$ Since $\bar p$ and ${p}/({n-1})$ are Hölder conjugate numbers, it follows, by the standard approximation arguments, that identity (2.3) holds for the given functions.

Lemma 2.2 Let $H(t)=th(t)-\int _0^t h(s)\,{\rm d}s.$ Then

(2.4)\begin{equation} \int_\Omega h(\det Dw) \operatorname{cof} Dw :D((Dw) \phi) = \int_\Omega H(\det Dw) \operatorname{div}\phi \end{equation}

holds for all $w\in C^2(\Omega ;\mathbb {R}^n)$ and $\phi \in C_c^1(\Omega ;\mathbb {R}^n).$

Proof. First we assume $h\in C^1(\mathbb {R})$ and let $a (x)=h(\det Dw(x)).$ Then $a\in C^1(\Omega )$ and $Da=h'(\det Dw ) D(\det Dw ).$ Take $\zeta =(Dw)\phi \in C_c^1(\Omega ;\mathbb {R}^n)$ in (2.3) and, from $Dw^T \operatorname {cof} Dw=(\det Dw)I$ and $H'(t)=th'(t),$ we have

\begin{align*} & \int_\Omega h(\det Dw) \operatorname{cof} Dw :D((Dw) \phi) \\ & \quad ={-}\int_\Omega h'(\det Dw ) (\operatorname{cof} Dw )D(\det Dw ) \cdot (Dw)\phi\\ & \quad ={-}\int_\Omega h'(\det Dw) (\det Dw) D(\det Dw ) \cdot \phi \\ & \quad ={-}\int_\Omega H'(\det Dw) D(\det Dw ) \cdot \phi \\ & \quad ={-}\int_\Omega D(H(\det Dw) ) \cdot \phi = \int_\Omega H(\det Dw) \operatorname{div} \phi. \end{align*}

This proves identity (2.4) for $h\in C^1(\mathbb {R}).$ The proof of (2.4) for $h\in C(\mathbb {R})$ follows by approximating $h$ by $C^1$ functions.

Proposition 2.3 Let $u\in C^2(\Omega ;\mathbb {R}^n)$ be a weak solution of (2.1). Then $h(\det Du)$ is constant on $\Omega.$

Proof. Let $d(x)=\det Du(x);$ then $d\in C^1(\Omega ).$ By (2.2) and (2.4), it follows that $\int _\Omega H(d(x))\operatorname {div}\phi \,{\rm d}x=0$ for all $\phi \in C_c^1(\Omega ;\mathbb {R}^n);$ hence $H(d(x))=C$ is a constant on $\Omega.$ Suppose, on the contrary, that $h(d(x_1))\ne h(d(x_2))$ for some $x_1,\,x_2\in \Omega.$ Then $d_1=d(x_1)\ne d_2=d(x_2),$ say $d_1< d_2.$ Since $d(x)$ is continuous, by the intermediate value theorem, it follows that $H(t)=C$ for all $t\in [d_1,\,d_2].$ Solving for $h(t)$ from the integral equation $th(t)-\int _0^t h(s)\,{\rm d}s=C$ on $(d_1,\,d_2)$, we obtain that $h(t)$ is constant on $(d_1,\,d_2),$ contradicting $h(d_1)\ne h(d_2).$

Proposition 2.4 Let $p\ge n-1$, $\bar p={p}/({p+1-n}),$ and $u\in W^{1, p}_{loc}(\Omega ;\mathbb {R}^n)$ be a weak solution of (2.1) such that $h(\det Du)\in W^{1,\bar p}_{loc}(\Omega ).$ Then $h(\det Du)$ is constant almost everywhere on $\Omega.$

Proof. With $a=h(\det Du)\in W^{1,\bar p}_{loc}(\Omega )$ and $w=u\in W^{1, p}_{loc}(\Omega ;\mathbb {R}^n)$ in lemma 2.1 and by (2.2), we have

\[ 0= \int_\Omega h(\det Du)\operatorname{cof} Du:D \zeta ={-}\int_\Omega (\operatorname{cof} Du)D(h(\det Du)) \cdot \zeta \]

for all $\zeta \in C_c^1(\Omega ;\mathbb {R}^n).$ As a result, we have $(\operatorname {cof} Du)D(h(\det Du ))=0$ a.e. on $\Omega.$ Thus $D(h(\det Du ))=0$ a.e. on the set $\Omega _0=\{x\in \Omega :\det Du(x)\ne 0\}.$ Clearly, $D(h(\det Du ))=0$ a.e. on the set $E=\{x\in \Omega :\det Du(x) = 0\}$ because $h(\det Du)=h(0)$ a.e. on $E.$ Therefore, $D(h(\det Du ))=0$ a.e. on the whole domain $\Omega ;$ this proves that $h(\det Du)$ is constant a.e. on $\Omega.$

2.2. The change of variables

The formal calculations leading to proposition 2.3 do not work for the $C^1$ weak solutions of equation (2.1); however, the same conclusion still holds, as has been discussed in [8] without proof. We give a proof by choosing suitable test functions based on change of variables.

Let us first recall the change of variables for general Sobolev functions; see, e.g., [14]. Let $p>n$ and $w\in W^{1,p}(\Omega ;\mathbb {R}^n);$ then $w\in C_{loc}^\alpha (\Omega ;\mathbb {R}^n)$ with $\alpha =1-({n}/{p}).$ Let $E$ be a measurable subset of $\Omega.$ Denote by $N(w|E;y)$ the cardinality of the set $\{x\in E: w(x)=y\}.$ Then, for all measurable functions $g\colon w(\Omega )\to \mathbb {R},$ the change of variable formula:

(2.5)\begin{equation} \int_E g(w(x)) |\det Dw(x)|\, {\rm d}x =\int_{w(E)} g(y) N(w|E;y) \,{\rm d}y \end{equation}

is valid, whenever one of the two sides is meaningful (see [14, Theorem 2]).

Theorem 2.5 Let $u\in C^1(\Omega ;\mathbb {R}^2)$ be a weak solution of equation (2.1). Then $h(\det Du)$ is constant on $\Omega.$

Proof. Let $E=\{x\in \Omega :\det Du(x)=0\},$ which is relatively closed in $\Omega.$ There is nothing to prove if $E=\Omega ;$ thus we assume $\Omega _0=\Omega \setminus E\ne \emptyset.$ Let $C$ be a component of the open set $\Omega _0.$ Without loss of generality, we assume $\det Du>0$ on $C.$ Let $x_0\in C$ and $y_0=u(x_0).$ Since $y_0$ is a regular value of $u$, by the inverse function theorem, there exists an open disc $D=B_\epsilon (y_0)$ such that

\[ \begin{cases} U=u^{{-}1}(D)\ \mbox{is a subdomain of }C;\\ \det Du>0\ \mbox{on} \ U;\\ u:U\to D\ \mbox{is bijective with inverse} \ v=u^{{-}1}:D\to U;\\ v\in C^1(D;\mathbb{R}^n). \end{cases} \]

Given any $\phi \in C^1_c(D;\mathbb {R}^n),$ the function $\zeta (x)=\phi (u(x))\in C^1_c(U;\mathbb {R}^n)$ is a test function for (2.2) with $D\zeta (x)=D\phi (u(x))Du(x);$ thus, by the change of variables, we obtain that

\begin{align*} 0& =\int_\Omega h(\det Du)\operatorname{cof} Du : D\zeta \,{\rm d}x\\ & =\int_U h(\det Du(x))\operatorname{cof} Du(x) : D\phi(u(x))Du(x) \, {\rm d}x \\ & =\int_U h(\det Du(x))\det Du(x) \operatorname{tr}(D\phi(u(x))) \, {\rm d}x \\ & =\int_D h(\det Du(v(y))\operatorname{div} \phi(y) \,{\rm d}y. \end{align*}

This holding for all $\phi \in C^1_c(D;\mathbb {R}^n)$ proves that $h(\det Du(v(y))$ is constant on $D;$ hence $h(\det Du)$ is constant on $U.$ Since $C$ is connected and $h$ is continuous, it follows that $h(\det Du)$ is constant on the relative closure $\bar C$ of $C$ in $\Omega.$ If $E=\emptyset,$ we have $C=\Omega ;$ hence, $h(\det Du)$ is constant on $\Omega.$ If $E\ne \emptyset,$ we have $\bar C\cap E\ne \emptyset$ and thus $h(\det Du)=h(0)$ on $\bar C,$ which proves that $h(\det Du) =h(0)$ on $\Omega _0;$ hence $h(\det Du) =h(0)$ on the whole $\Omega.$

Corollary 2.6 Let $u$ be a weak solution of equation (2.1). Then $\det Du$ is constant almost everywhere on $\Omega$ if one of the following assumptions holds:

1. (a) $u \in C^1(\Omega ;\mathbb {R}^n)$ and $h$ is not constant on any intervals.

2. (b) $u\in W^{1,p}_{loc}(\Omega ;\mathbb {R}^n)$, $h(\det Du)\in W^{1,\bar p}_{loc}(\Omega )$, where $n-1\le p\le \infty$ and $\bar p={p}/({p+1-n})$ are given numbers, and $h$ is one-to-one.

Proof. Assuming (a), by theorem 2.5, we have that $h(\det Du)=c$ is constant in $\Omega$ and thus $\det Du(x)\in h^{-1}(c).$ Since $\Omega$ is connected, $\det Du$ is continuous in $\Omega$ and $h^{-1}(c)$ contains no intervals, it follows that $\det Du$ is constant in $\Omega.$ Assuming (b), by proposition 2.4, we have that $h(\det Du)$ is constant a.e. on $\Omega.$ Since $h$ is one-to-one, it follows that $\det Du$ is constant a.e. on $\Omega.$

The following result applies to the weak solutions $u$ of (2.1) such that $u\in C^1(\Omega ;\mathbb {R}^n)$ or $u\in W^{1,p}_{loc}(\Omega ;\mathbb {R}^n)$ with $h(\det Du)\in W^{1,\bar p}_{loc}(\Omega )$ for some $p\ge n$ and $\bar p={p}/({p+1-n}).$

Proposition 2.7 Assume $h$ is nondecreasing and let $f(t)=\int _0^t h(s)\,{\rm d}s.$ Suppose that $u\in W^{1,n}_{loc}(\Omega ;\mathbb {R}^n)$ is a weak solution of (2.1) such that $h(\det Du)$ is constant a.e. on $\Omega.$ Then for all subdomains $G\subset \subset \Omega,$ the inequality

\[ \int_G f(\det Du(x))\,{\rm d}x \le \int_G f(\det Dv(x))\,{\rm d}x \]

holds for all $v\in W^{1,n}(G;\mathbb {R}^n)$ satisfying $v-u\in W^{1,n}_0(G;\mathbb {R}^n).$

Proof. Let $h(\det Du)=\mu$ be a constant a.e. on $\Omega.$ Since $f'=h$ is nondecreasing, it follows that $f$ is convex and thus $f(t)\ge f(t_0)+h(t_0)(t-t_0)$ for all $t,\, t_0\in \mathbb {R}.$ Let $v\in W^{1,n}(G;\mathbb {R}^n)$ satisfy $v-u\in W^{1,n}_0(G;\mathbb {R}^n).$ Then

\[ f(\det Dv)\ge f(\det Du) +\mu (\det Dv-\det Du) \quad \mbox{a.e. on}\ \Omega. \]

Integrating over $G,$ since $\int _G \det Dv=\int _G \det Du$, we have

\[ \int_G f(\det Dv ) \ge \int_G f(\det Du ) +\mu \int_G (\det Dv-\det Du) =\int_G f(\det Du). \]

2.3. Weak solutions in $W^{1,p}(\Omega ;\mathbb {R}^n)$ for $p\ge n$

It remains open that whether weak solutions $u\in W^{1,n}_{loc}(\Omega ;\mathbb {R}^n)$ of equation (2.1) must have a constant $h(\det Du)$ a.e. on $\Omega$ without assuming $h(\det Du)\in W^{1,1}_{loc}(\Omega ).$ In fact, despite the example of very weak solutions in example 3.4, we do not know whether a weak solution $u\in W^{1,n}_{loc}(\Omega ;\mathbb {R}^n)$ of (2.1) satisfying $h(\det Du)\in L^q_{loc}(\Omega )$ for some $q>1$ will have a constant $h(\det Du)$ a.e. on $\Omega.$

However, we have some partial results. The following result concerns certain homeomorphism weak solutions of equation (2.1).

Theorem 2.8 Assume $h$ is nondecreasing. Let $p>n$, $\tilde p={p}/({p-n}),$ and let $u\in W^{1,p}(\Omega ;\mathbb {R}^n)$ be a weak solution of (2.1) with $h(\det Du)\in L^{\tilde p}(\Omega )$ such that

(2.6)\begin{equation} \begin{cases} u \mbox{ is one-to-one on } \Omega,\\ u(\Omega) \ \mbox{is a domain in} \ \mathbb{R}^n,\\ v =u^{{-}1}\in C(u(\Omega);\mathbb{R}^n)\ \mbox{satisfies the } {Luzin} N-property: \\ |v(B)|=0\ \mbox{for all} \ B\subset u(\Omega)\ \mbox{with} \ |B|=0. \end{cases} \end{equation}

Then $h(\det Du)$ is a constant a.e. on $\Omega.$

Proof. Recall that $\operatorname {div}(\operatorname {cof} (Du))=0.$ Without loss of generality, we assume ${h(0)=0};$ thus $h(t)t=|h(t)t|\ge 0$ for all $t\in \mathbb {R}.$ From the assumption, we have

\[ h(\det Du)\operatorname{cof} Du\in L^{p'}(\Omega;\mathbb{M}^{n\times n});\quad p'= {p}/(p-1). \]

Let $\phi \in C^1_c(u(\Omega );\mathbb {R}^n).$ Then $\zeta (x)=\phi (u(x))\in W^{1,p}_0(\Omega ;\mathbb {R}^n)$ is a legitimate test function for (2.2). Since $u$ is one-to-one on $\Omega,$ we have $N(u|\Omega ;y) =1$ for all $y\in u(\Omega );$ thus, by the change of variable formula (2.5), we obtain

\begin{align*} 0& =\int_\Omega h(\det Du)\operatorname{cof} Du : D\zeta \,{\rm d}x\\ & =\int_\Omega h(\det Du(x))\operatorname{cof} Du(x) : D\phi(u(x))Du(x) \, {\rm d}x \\ & =\int_\Omega \operatorname{tr}(D\phi(u(x))) h(\det Du(x))\det Du(x) \, {\rm d}x \\ & =\int_\Omega (\operatorname{div} \phi)(u(x)) |h(\det Du(v(u(x)))| |\det Du(x) |\, {\rm d}x \\ & =\int_{u(\Omega)} \operatorname{div} \phi(y) |h(\det Du(v(y))| N(u|\Omega;y)\,{\rm d}y\\ & =\int_{u(\Omega)}\operatorname{div} \phi(y) |h(\det Du(v(y))| \,{\rm d}y. \end{align*}

This holding for all $\phi \in C^1_c(u(\Omega );\mathbb {R}^n)$ proves that $|h(\det Du(v(y))|$ is constant a.e. on $u(\Omega );$ hence $|h(\det Du)|=\lambda$ is a constant a.e. on $\Omega.$ If $\lambda =0$ then $h(\det Du)=0$ a.e. on $\Omega.$ We now assume $\lambda >0.$ Let

\[ \Omega_+{=}\{x\in\Omega: h(\det Du(x))=\lambda\},\quad \Omega_-{=}\{x\in\Omega: h(\det Du(x))={-}\lambda\}. \]

Then $|\Omega _+|+|\Omega _-|=|\Omega |.$ Since $\lambda >0$ and $h$ is nondecreasing, we have $\det Du\ge 0$ a.e. on $\Omega _+$ and $\det Du\le 0$ a.e. on $\Omega _-.$ We claim that either $|\Omega _+|=0$ or $|\Omega _+|=|\Omega |,$ which proves the theorem. To prove the claim, we observe that, for all $\zeta \in C^1_c(\Omega ;\mathbb {R}^n),$

\[ 0=\int_\Omega h(\det Du)\operatorname{cof} Du : D\zeta=\lambda \int_{\Omega_+} \operatorname{cof} Du : D\zeta -\lambda \int_{\Omega_-} \operatorname{cof} Du : D\zeta; \]

moreover,

\[ 0=\int_\Omega \operatorname{cof} Du : D\zeta = \int_{\Omega_+} \operatorname{cof} Du : D\zeta + \int_{\Omega_-} \operatorname{cof} Du : D\zeta, \]

and hence,

\[ \int_{\Omega_+} \operatorname{cof} Du : D\zeta \,{\rm d}x =0 \quad \forall\ \zeta\in C^1_c(\Omega;\mathbb{R}^n). \]

As above, we take $\zeta (x)=\phi (u(x))$ with arbitrary $\phi \in C^1_c(u(\Omega );\mathbb {R}^n)$ to obtain

\[ 0=\int_{\Omega_+} \operatorname{cof} Du : D\zeta \,{\rm d}x =\int_{u(\Omega_+)} \operatorname{div} \phi(y) \,{\rm d}y =\int_{u(\Omega)} \chi_{u(\Omega_+)}(y)\operatorname{div} \phi(y) \,{\rm d}y. \]

Again, this holding for all $\phi \in C^1_c(u(\Omega );\mathbb {R}^n)$ proves that $\chi _{u(\Omega _+)}$ is constant a.e. on $u(\Omega );$ hence, either $|u(\Omega _+)|=0$ or $|u(\Omega _+)|=|u(\Omega )|.$ If $|u(\Omega _+)|=0,$ then $\int _{\Omega _+} \det Du \,{\rm d}x= |u(\Omega _+)| =0;$ thus $\det Du=0$ a.e. on $\Omega _+,$ which proves $|\Omega _+|=0$ because, otherwise, we would have $\lambda =h(0)=0.$ Similarly, if $|u(\Omega _+)|=|u(\Omega )|$ then $|\Omega _+|=|\Omega |.$ This completes the proof.

Remark 2.9 A sufficient condition for the invertibility of Sobolev functions has been given in [1]. For example, suppose that $\Omega$ is a bounded Lipschitz domain and $u_0\in C(\bar \Omega ;\mathbb {R}^n)$ is such that $u_0$ is one-to-one on $\bar \Omega$ and $u_0(\Omega )$ satisfies the cone condition. Then, condition (2.6) is satisfied provided that

\[ \begin{cases} u|_{\partial\Omega}=u_0,\\ \det Du >0 \text{ a.e.}\,\text{on} \ \Omega,\\ \int_\Omega |\operatorname{cof} Du|^q(\det Du)^{1-q} \,{\rm d}x<\infty\ \mbox{for some}\ q>n. \end{cases} \]

See [9] for recent studies and more references in this direction.

The following result concerns the weak solutions with certain linear Dirichlet boundary conditions.

Proposition 2.10 Let $h(0)=0$, $p\ge n$, $\tilde p={p}/({p-n}),$ and $u\in W^{1,p}(\Omega ;\mathbb {R}^n)$ with $h(\det Du)\in L^{\tilde p}(\Omega )$ be a weak solution of (2.1) satisfying the Dirichlet boundary condition $u|_{\partial \Omega }=Ax,$ where $A\in \mathbb {M}^{n\times n}$ is given. Let

\[ \lambda=\int_\Omega h(\det Du)\det Du\,{\rm d}x, \quad B=\int_\Omega h(\det Du) \operatorname{cof} Du\,{\rm d}x. \]

Then $BA^T=\lambda I.$ Moreover, if $\det A=0$ and $h$ is one-to-one, then $\det Du=0$ a.e. on $\Omega,$ and thus $B=0.$

Proof. For $P\in \mathbb {M}^{n\times n},$ the function $\zeta (x)=P(u(x)-Ax)\in W^{1,p}_0(\Omega ;\mathbb {R}^n)$ is a legitimate test function for (2.2), which, from $D\zeta =PDu-PA$ and $\operatorname {cof} Du: PDu =( \operatorname {tr} P )\det Du,$ yields that

\[ (\operatorname{tr} P) \int_\Omega h(\det Du)\det Du \,{\rm d}x= \int_\Omega h(\det Du)\operatorname{cof} Du:PA\,{\rm d}x. \]

This is simply $(\lambda I-BA^T):P=0.$ Since $P\in \mathbb {M}^{n\times n}$ is arbitrary, we have $BA^T=\lambda I.$ If $\det A=0,$ then $\lambda =0.$ Furthermore, if $h$ is one-to-one, then $\lambda =0$ implies $\det Du=0$ a.e. on $\Omega$ and thus $B=0.$

Remark 2.11

1. (i) Assume $h(0)=0$ and $h$ is one-to-one. If $\det A\ne 0$, then $B=\mu h(\det A)\operatorname {cof} A,$ where $\mu ={\lambda }/{h(\det A)\det A}>0.$ It remains open whether $\mu =1.$ Note that if $\mu \ne 1$ then $\det Du$ cannot be a constant a.e. on $\Omega.$

2. (ii) There are many (very) weak solutions $u\in W^{1,p}(\Omega ;\mathbb {R}^n)$ of equation (2.1) satisfying $u|_{\partial \Omega }=Ax$ for some $p< n$ such that $\det Du$ is not constant a.e. on $\Omega.$ See example 3.4.

3. Radially symmetric solutions

Let $B=B_1(0)$ be the open unit ball in $\mathbb {R}^n.$ We consider the radially symmetric or radial functions

(3.1)\begin{equation} u(x)= \phi(|x|) x, \end{equation}

where $\phi \colon (0,\,1)\to \mathbb {R}$ is weakly differentiable. With $r=|x|$ and $\omega ={x}/{|x|}$, we have

(3.2)\begin{equation} \begin{cases} Du(x) =\phi(r) I + r\phi'(r)\, \omega\otimes \omega, \\ \det Du(x) = \phi(r)^n +r\phi'(r) \phi(r)^{n-1}, \\ \operatorname{cof} Du(x) = \alpha(r)I+\beta(r) \, \omega\otimes \omega, \end{cases} \end{equation}

for a.e. $x\in B,$ where $\alpha (r)=\phi ^{n-1}+r\phi ^{n-2}\phi '$ and $\beta (r)=-r\phi ^{n-2}\phi '.$

3.1. Some properties of radial functions

We study some properties of radial functions pertaining to equation (2.1).

Lemma 3.1 Let $p\ge 1$ and $v\in L^p_{loc}(B\setminus \{0\}).$ Define $\tilde v=M(v)\colon (0,\,1)\to \mathbb {R}$ by setting

(3.3)\begin{equation} \tilde v(r) =M(v)(r)= {\int \hspace{-11pt} - \hspace{-1pt}}_{S_r}v(x) \,{\rm d}\sigma_r=\frac{1}{\omega_n} \int_{S_1}v(r\omega) \,{\rm d}\sigma_1, \end{equation}

where $S_r=\partial B_r(0)$, ${\rm d}\sigma _r= {\rm d} \mathcal {H}^{n-1}$ denotes the $(n-1)$-Hausdorff measure on $S_r,$ and $\omega _n=\mathcal {H}^{n-1}(S_1).$ Then $\tilde v\in L^p_{loc}(0,\,1).$ Furthermore, if $v\in W^{1,p}_{loc}(B\setminus \{0\}),$ then $\tilde v\in W^{1,p}_{loc}(0,\,1)$ with

\[ \tilde v'(r)= {1}/{\omega_n} \int_{S_1}Dv(r\omega)\cdot \omega \,{\rm d}\sigma_1 =r^{{-}1}M(Dv\cdot x)(r) \quad a.e.\; r\in (0,1). \]

Since $W^{1,p}_{loc}(0,\,1)\subset C(0,\,1)$, $\tilde v$ can be identified as a continuous function in $(0,\,1)$ if $v\in W^{1,p}_{loc}(B\setminus \{0\}).$

Proof. Note that

\[ |\tilde v(r)| \le {\int \hspace{-11pt} - \hspace{-1pt}}_{S_r}|v| \,{\rm d}\sigma_r \le \left ( {\int \hspace{-11pt} - \hspace{-1pt}}_{S_r}|v|^p \,{\rm d}\sigma_r\right)^{1/p}. \]

Thus, for all $0< a< b<1$,

\[ \int_a^b \omega_nr^{n-1}|\tilde v|^p\,{\rm d}r\le \int_a^b \left ( \int_{S_r}|v|^p \,{\rm d}\sigma_r\right){\rm d}r=\int_{a<|x|< b} |v(x)|^p\,{\rm d}x<\infty. \]

This proves $\tilde v\in L^p_{loc}(0,\,1).$ Now assume $v\in W^{1,p}_{loc}(B\setminus \{0\})$ and let

\[ g(r)= {1}/{\omega_n} \int_{S_1}Dv(r\omega)\cdot \omega \,{\rm d}\sigma_1 =r^{{-}1}M(Dv\cdot x)(r) \in L^p_{loc}(0,1). \]

Let $0< a< b<1$ and $\eta \in C^\infty _c(a,\,b).$ Then

\begin{align*} \int_a^b \tilde v(r)\eta'(r)\,{\rm d}r& =\frac{1}{\omega_n} \int_a^b r^{1-n}\eta'(r) \Big( \int_{S_r} v \,{\rm d}\sigma_r\Big) {\rm d}r\\ & =\frac{1}{\omega_n} \int_{a<|x|< b} |x|^{1-n}\eta'(|x|)v(x)\,{\rm d}x\\ & =\frac{1}{\omega_n} \int_{a<|x|< b} D(\eta(|x|))\cdot (v|x|^{{-}n}x)\,{\rm d}x\\ & ={-}\frac{1}{\omega_n} \int_{a<|x|< b} \eta(|x|) \operatorname{div}(v|x|^{{-}n}x)\,{\rm d}x\\ & ={-}\frac{1}{\omega_n} \int_{a<|x|< b} \eta(|x|) Dv\cdot (|x|^{{-}n}x)\,{\rm d}x\\ & ={-}\frac{1}{\omega_n} \int_a^b \eta(r) r^{{-}n} \Big (\int_{S_r} Dv(x)\cdot x{\rm d}\sigma_r\Big )\,{\rm d}r ={-}\int_a^b g(r)\eta(r)\,{\rm d}r. \end{align*}

This proves $g=\tilde v'.$

Proposition 3.2 Assume $\phi \in W_{loc}^{1,1}(0,\,1)$ and $u(x)=\phi (|x|)x.$ Let $p\ge 1$ and $p'={p}/({p-1}).$ Suppose that $q(r)$ is a measurable function on $(0,\,1)$ such that

(3.4)\begin{equation} q(|x|)\operatorname{cof} Du\in L^p_{loc}(B\setminus \{0\}). \end{equation}

Then, for all $0< a< b<1$ and $v\in W_{loc}^{1,p'}(B;\mathbb {R}^n),$ it follows that

(3.5)\begin{equation} \int_{a<|x|< b} q(|x|)\operatorname{cof} Du:Dv \,{\rm d}x = \omega_n \int_a^b q(r)(r^{n-2}\phi^{n-1}\psi)' \,{\rm d}r, \end{equation}

where $\psi (r)=M(v\cdot x)(r)={r}/{\omega _n} \int _{S_1}v(r\omega )\cdot \omega \,{\rm d}\sigma _1.$

Proof. Note that $q(|x|)\operatorname {cof} Du:Dv\in L^1_{loc}(B\setminus \{0\}).$ Since $\operatorname {cof} Du: Dv =\alpha (r)\operatorname {div} v + \beta (r) Dv:(\omega \otimes \omega ),$ we have

(3.6)\begin{align} \int_{a<|x|< b} q(|x|)\operatorname{cof} Du:Dv \,{\rm d}x & =\int_a^b \Big( \int_{S_r} q(|x|)\operatorname{cof} Du:Dv \,{\rm d}\sigma_r\Big ){\rm d}r \nonumber\\ & = \int_a^b q(r) \Big (\alpha(r) \int_{S_r} \operatorname{div} v\,d \sigma_r\nonumber\\ & \quad + \beta(r) \int_{S_r}Dv:(\omega\otimes \omega) \,{\rm d}\sigma_r \Big ) {\rm d}r. \end{align}

We now compute the two spherical integrals. First, for all $a< t<1,$ by the divergence theorem,

\begin{align*} \int_a^t \int_{S_r} \operatorname{div} v\,{\rm d}\sigma_r \,{\rm d}r & =\int_{a<|x|< t}\operatorname{div} v\,{\rm d}x\\ & =\int_{S_t} v\cdot \frac{x}{t}\,{\rm d}\sigma_t-\int_{S_a} v\cdot \frac{x}{a}\,{\rm d}\sigma_a =\omega_n t^{n-2}\psi(t)-\omega_n a^{n-2}\psi(a). \end{align*}

Hence

\[ \int_{S_r} \operatorname{div} v\,{\rm d}\sigma_r = \omega_n (r^{n-2}\psi(r))' \quad a.e. \ r\in (0,1). \]

Second, since $D(v\cdot x) \cdot x =Dv : (x\otimes x) +v\cdot x,$ we have

\[ \psi'(r)= r^{{-}1} M(D(v\cdot x) \cdot x)= r^{{-}1} M(Dv : (x\otimes x)) + r^{{-}1} M(v\cdot x). \]

Thus $M(Dv : (x\otimes x)) =r \psi ' - \psi$ and hence

\begin{align*} \int_{S_r}Dv:(\omega\otimes \omega) \,{\rm d}\sigma_r & =\omega_n r^{n-3}M(Dv : (x\otimes x)) =\omega_n r^{n-2} \psi' -\omega_n r^{n-3}\psi\\ & = \omega_n (r^{n-2}\psi)' -(n-1)\omega_n r^{n-3}\psi. \end{align*}

Since $\alpha +\beta =\phi ^{n-1}$ and $\beta =-r\phi ^{n-2}\phi '$, elementary computations lead to

\begin{align*} & \alpha(r) \int_{S_r} \operatorname{div} v\,{\rm d}\sigma_r+ \beta(r) \int_{S_r}Dv:(\omega\otimes \omega) \,{\rm d}\sigma_r\\ & \quad = \omega_n \alpha (r^{n-2}\psi)' + \omega_n \beta [(r^{n-2}\psi)' -(n-1) r^{n-3}\psi]=\omega_n (\phi^{n-1}r^{n-2}\psi)' \end{align*}

for a.e. $r\in (0,\,1).$ Finally, (3.5) follows from (3.6).

Theorem 3.3 Assume $h\in C(\mathbb {R})$ is one-to-one, $p\ge {n}/({n-1})$ and $\phi \in W^{1,p}_{loc}(0,\,1).$ Let $u(x)=\phi (|x|)x$ be a weak solution of (2.1) such that

(3.7)\begin{equation} h(\det Du)\operatorname{cof} Du\in L_{loc}^{{p}/{(p-1)}}(B;\mathbb{M}^{n\times n}). \end{equation}

Then either $\phi \equiv 0,$ or

\[ \phi(r)=\left(\lambda + \frac{c}{r^n}\right)^{1/n}\ne 0\quad\forall\ 0< r<1, \]

where $\lambda$ and $c$ are constants. (When $n$ is even, we need $\lambda + {c}/({r^n})> 0$ in $(0,\,1)$ and there are two nonzero branches of the $n$th roots.)

Proof. Let $S=\{r\in (0,\,1):\phi (r)\ne 0\};$ then $S$ is open. If $S=\emptyset,$ then $\phi \equiv 0.$ Assume $S$ is nonempty. Let $(a,\,b)$ be a component of $S.$ Let $\eta (r)\in C_c^\infty (0,\,1)$ be any function with compact support contained in $(a,\,b).$ Define the radial function

\[ \zeta(x)=\begin{cases} \dfrac{\eta(r) }{r^{n}\phi(r)^{n-1}} \,x & \mbox{if} \ r=|x|\in (a,b),\\ 0 & \mbox{otherwise.}\end{cases} \]

Then $\zeta \in W^{1,p}(B;\mathbb {R}^n)$ with $\operatorname {supp} \zeta \subset \{a<|x|< b\}.$ Let $\psi =M(\zeta \cdot x);$ then $r^{n-2}\phi ^{n-1}\psi =\eta.$ Let $\det Du=\phi ^n +r\phi ' \phi ^{n-1}=:d(r).$ By assumption (3.7), $\zeta$ is a legitimate test function for equation (2.1); thus, by (3.5), we obtain that

\begin{align*} 0& = \int_B h(\det Du)\operatorname{cof} Du: D\zeta\,{\rm d}x\\ & = \int_a^b h(d(r)) (r^{n-2}\phi^{n-1}\psi)'\, {\rm d}r =\int_a^b h(d(r))\eta'(r){\rm d}r. \end{align*}

This holds for all $\eta \in C^\infty _c(a,\,b);$ thus $h(d(r))$ is constant a.e. in $(a,\,b)$. As $h$ is one-to-one, we have that $d(r)$ is constant a.e. in $(a,\,b).$ Assume $d(r) =\phi ^n +r\phi ' \phi ^{n-1}= \lambda$ in $(a,\,b).$ Solving the differential equation we obtain that

\[ \phi(r)\ne 0,\quad \phi(r)=\left(\lambda + \frac{c}{r^n}\right)^{1/n} \quad (a< r< b). \]

If one of $a$ and $b$ is inside $(0,\,1),$ then $\phi =0$ at this point; but in this case, $\phi \notin W^{1,q}_{loc}(0,\,1)$ for any $q\ge {n}/{n-1}.$ So $(a,\,b)=(0,\,1);$ this completes the proof.

3.2. Very weak solutions

We consider some examples of (very) weak solutions of (2.1) in $W^{1,p}(B;\mathbb {R}^n)$ with $p<{n}/{n-1}.$

Example 3.4 Let $n\ge 2$, $0< a\le b<1$ and $\lambda _1\ne \lambda _2.$ Let $u=\phi (|x|)x$, where

(3.8)\begin{equation} \phi (r)=\begin{cases} [\lambda_1 (r^n-a^n)]^{1/n}/{r} & (0< r \le a),\\ 0 & (a< r \le b),\\ [\lambda_2 (r^n-b^n)]^{1/n}/{r} & (b < r\le 1). \end{cases} \end{equation}

Then $u \in W^{1,p}(B;\mathbb {R}^n)$ for all $1\le p< {n}/({n-1})$ and $u$ is a weak solution of equation (2.1) in $B$ satisfying the Dirichlet boundary condition

\[ u|_{\partial B}=Ax=[\lambda_2 (1-b^n)]^{1/n}x, \]

but $\det Du$ is not a constant on $B.$ Moreover, if $\lambda _2=0$, then $u\chi _B$ is a weak solution of (2.1) on the whole $\mathbb {R}^n$; namely,

(3.9)\begin{equation} \int_B h(\det Du) \operatorname{cof} Du:D\zeta =0\quad\forall\ \zeta\in C^1(\mathbb{R}^n;\mathbb{R}^n). \end{equation}

Proof. If $\lambda _1=0$, then only $Du$ blows up at $r=|x|=b,$ with $|Du(x)|\approx |\phi '(r)|\approx |r-b|^{{1}/({n})-1}$ and $|\operatorname {cof} Du(x)|\approx |\phi (r)|^{n-2}|\phi '(r)|\approx |r-b|^{-{1}/{n}}$ near $r=b.$ Hence, in this case, $u \in W^{1,p}(B;\mathbb {R}^n)$ for $1\le p< {n}/({n-1})$ and $\operatorname {cof} Du\in L^q(B;\mathbb {M}^{n\times n})$ for all $1\le q< n.$

If $\lambda _1\ne 0$, then $u$ and $Du$ also blow up at $x=0$ and $Du$ blows up at $r=a$ and $r=b.$ In this case, the similar blow-up estimates show that $u \in W^{1,p}(B;\mathbb {R}^n)$ and $\operatorname {cof} Du\in L^p(B;\mathbb {M}^{n\times n})$ for $1\le p< {n}/({n-1}).$

Hence, in all cases, $u \in W^{1,p}(B;\mathbb {R}^n)$ and $h(\det Du) \operatorname {cof} Du \in L^p(B;\mathbb {M}^{n\times n})$ for all $1\le p< {n}/({n-1}).$ Given any $\zeta \in C^1(\mathbb {R}^n;\mathbb {R}^n),$ let $\psi (t)= M(\zeta \cdot x)(t)={t}/{\omega _n} \int _{S_1}\zeta (t\omega )\cdot \omega \,{\rm d}\sigma _1.$ Then

\[ \lim_{t\to 0^+} (t^{{-}1}\psi(t))= \lim_{t\to 0^+} \frac{1}{\omega_n} \int_{S_1}\zeta (t\omega)\cdot \omega \,{\rm d}\sigma_1=0. \]

By proposition 3.2, we have

\begin{align*} \int_B h(\det Du) \operatorname{cof} Du:D\zeta & =\lim_{t\to 0^+ } \int_{t<|x|<1} h(\det Du) \operatorname{cof} Du:D\zeta \\ & = h(\lambda_1) \lim_{t\to 0^+} \int_{t<|x|< a} \operatorname{cof} Du:D\zeta\\ & \quad + h(\lambda_2) \int_{b<|x|<1} \operatorname{cof} Du:D\zeta \\ & ={-}\omega_n h(\lambda_1) \lim_{t\to 0^+} t^{n-2}\phi(t)^{n-1}\psi(t)\\ & \quad +\omega_n h(\lambda_2) (t^{n-2}\phi(t)^{n-1}\psi(t))|_b^1\\ & =\omega_n h(\lambda_2) \phi(1)^{n-1}\psi(1). \end{align*}

If $\zeta \in C^1_c(B;\mathbb {R}^n)$, then $\psi (1)=0$; this proves that $u$ is a weak solution of (2.1). Moreover, if $\lambda _2=0$ then $\phi (1)=0;$ in this case, we obtain (3.9).

Example 3.5 Let $\Omega$ be any bounded domain in $\mathbb {R}^n$, $\{\bar B_{r_i}(c_i)\}_{i=1}^\infty$ be a family of disjoint-closed balls in $\Omega,$ and $u_i(x)= \phi _i(|x|)x$ be the radial function on $B=B_1(0),$ where $\phi _i(r)$ is defined by (3.8) with $\lambda _1=t_i\ne 0$, $a=a_i\in (0,\,1)$ and $\lambda _2=0.$ By choosing suitable $t_i$ and $a_i$, we assume

\[ \|u_i\|_{W^{1,p}(B)}+\|\operatorname{cof} Du_i\|_{L^p(B)} \le M\quad \forall\ i=1,2,\cdots \]

for some constants $M>0$ and $1\le p< {n}/({n-1}).$ Define

\[ u(x)=\begin{cases} r_i u_i(\frac{x-c_i}{r_i}) & x\in B_i=B_{r_i}(c_i),\\ 0 & x\in \Omega\setminus \cup_{i=1}^\infty B_i. \end{cases} \]

Then $u \in W^{1,p}(\Omega ;\mathbb {R}^n)$, $\operatorname {cof} Du\in L^p(\Omega ;\mathbb {M}^{n\times n})$ and $|\{x\in \Omega : \det Du(x)=t_i\}|\ge a_i^n |B_i|$ (with equality holding if $t_i\ne t_j$ for all $i\ne j$).

Moreover, $u$ is a weak solution of (2.1) on $\Omega ;$ in fact, $u\chi _\Omega$ is a weak solution of (2.1) on $\mathbb {R}^n.$ To see this, given any $\zeta \in C^1(\mathbb {R}^n;\mathbb {R}^n),$ we observe that

\begin{align*} \int_\Omega h(\det Du) \operatorname{cof} Du:D\zeta \,{\rm d}x & =\sum_{i=1}^\infty \int_{B_i} h(\det Du) \operatorname{cof} Du:D\zeta\,{\rm d}x \\ & =\sum_{i=1}^\infty r_i^n \int_{B} h(\det Du_i(z)) \operatorname{cof} Du_i(z):\\ & \qquad (D\zeta)(c_i+r_iz)\,{\rm d}z \\ & =\sum_{i=1}^\infty \int_{B} h(\det Du_i(z)) \operatorname{cof} Du_i(z):D\zeta_i(z)\,{\rm d}z =0, \end{align*}

where $\zeta _i(z) =r_i^{n-1} \zeta (c_i+r_iz)\in C^1(\mathbb {R}^n;\mathbb {R}^n).$ By (3.9), we have

\[ \int_{B} h(\det Du_i(z)) \operatorname{cof} Du_i(z):D\zeta_i(z)\,{\rm d}z=0\quad \forall\ i=1,2,\cdots. \]

Hence $\int _\Omega h(\det Du) \operatorname {cof} Du:D\zeta \,{\rm d}x=0$ for all $\zeta \in C^1(\mathbb {R}^n;\mathbb {R}^n).$

3.3. Non-quasimonotonicity

Quasimonotonicity is an important condition related to the existence and regularity of weak solutions of certain systems of partial differential equations; see [2, 6, 7, 10, 11, 21].

Definition 3.6 A function $\sigma \colon \mathbb {M}^{n\times n}\to \mathbb {M}^{n\times n}$ is said to be quasimonotone at $A\in \mathbb {M}^{n\times n}$ provided that

\[ \int_\Omega \sigma(A+ D\phi(x)):D\phi(x)\,{\rm d}x \ge 0\quad \forall\ \phi\in C_c^1(\Omega;\mathbb{R}^n). \]

(This condition is independent of the domain $\Omega.$)

We have the following result, which holds for the model case $h(t)=t;$ the result also holds for a more general class of functions including $h(t)=e^t$, but we do not intend to dwell on the generality. There are other examples of quasiconvex functions whose gradient map is not quasimonotone; see [2, 7].

Theorem 3.7 Let $n\ge 2$ and $h\in C^1(\mathbb {R})$ be such that

(3.10)\begin{equation} \lambda t^{k_1} \le h'(t)\le \Lambda(t^{k_2}+1)\quad \forall\ t\ge 0, \end{equation}

where $\Lambda >\lambda >0$, $k_1$ and $k_2$ are constants such that $0\le k_1\le k_2< k_1+1.$ Then $\sigma (A)=h(\det A)\operatorname {cof} A$ is not quasimonotone at $I\in \mathbb {M}^{n\times n}.$

Proof. Let $\Omega$ be the unit ball in $\mathbb {R}^n.$ We show that there exists a radial function $\phi (x)=\rho (|x|)x,\,$ where $\rho \in W^{1,\infty }(0,\,1)$ with $\rho (1)=0,$ such that

(3.11)\begin{equation} \int_\Omega \sigma(I+ D\phi(x)):D\phi(x)\,{\rm d}x<0. \end{equation}

Using the spherical coordinates, we compute that

\[ \int_\Omega \sigma(I+ D\phi(x)):D\phi(x)\,{\rm d}x =\omega_n \int_0^1 P(r)\,{\rm d}r, \]

where

\begin{align*} P(r)& =h\Big ((1+ \rho)^{n} + (1+ \rho)^{n-1} \rho' r\Big ) \Big ( n\rho (1+\rho)^{n-1} +(1+n\rho)(1+\rho)^{n-2} \rho' r\Big)r^{n-1}\\ & =h(A+B)(C+D), \end{align*}

with $A =(1+\rho )^n,\, B =(1+\rho )^{n-1}\rho ' r,\, C =n\rho (1+\rho )^{n-1}r^{n-1}$ and $D =(1+n\rho) (1+\rho )^{n-2}\rho 'r^n.$ We write $h(A+B)=h(A+B)-h(A)+h(A)=EB+h(A),$ where

\[ E= \int_0^1h'(A +tB )\,{\rm d}t. \]

Thus $P=EBC+EBD+h(A)C+h(A)D.$

Let $0< a<1$ be fixed and $b=a-\epsilon$ with $0<\epsilon < a$ sufficiently small. Define

(3.12)\begin{equation} \rho=\rho_\epsilon(r)=\begin{cases} -1, & 0\le r\le b,\\ \frac{n-1}{n\epsilon }(r-a)-\frac{1}{n}, & b\le r \le a,\\ \frac{1}{n(1-a)} (r-1), & a\le r\le 1. \end{cases} \end{equation}

(See Fig. 1.) Then, with $P(r)=P_\epsilon (r)$, we have

(3.13)\begin{equation} \int_0^1 P_\epsilon (r)\,{\rm d}r=\int_b^a P(r)\,{\rm d}r+\int_a^1 P(r)\,{\rm d}r,\quad \Big |\int_a^1 P(r)\,{\rm d}r \Big |\le M_1, \end{equation}

where $M_1$ (likewise, each of the $M_k$'s below) is a positive constant independent of $\epsilon.$ For all $b< r< a$ we have $\rho '=({n-1})/{n\epsilon }$, $n\rho +1=({n-1})/{ \epsilon } (r-a)$ and $\rho +1 =({n-1}/{n\epsilon } (r-b).$ Hence $0< A<1$ and $B> 0$ on $(b,\,a)$; moreover,

(3.14)\begin{equation} \Big |\int_b^a \Big ( h(A)C+h(A)D \Big )\,{\rm d}r \Big |\le M_2. \end{equation}

Figure 1. The graph of $\rho =\rho _\epsilon (r),$ where $0< a<1$ is fixed and $b=a-\epsilon$ with $\epsilon \in (0,\,a)$ sufficiently small.

Moreover,

\[ \lambda\int_0^1 (A+tB)^{k_1} \,{\rm d}t \le E\le \Lambda \left (1+\int_0^1(A+tB)^{k_2} \,{\rm d}t\right ). \]

Since

\[ \int_0^1 (A+t B)^k \,{\rm d}t = \epsilon^{{-}k} \int_0^1 \big (\epsilon A+ (1+\rho)^{n-1}\frac{n-1}{n} r t \big )^k \,{\rm d}t, \]

it follows that for all $k\ge 0$

\[ M_3 \epsilon^{{-}k} (1+\rho)^{k(n-1)}r^k \le \int_0^1 (A+t B)^k \,{\rm d}t \le M_4 \epsilon^{{-}k}. \]

Thus

(3.15)\begin{equation} \Big |\int_b^a EBC \,{\rm d}r \Big |\le M_5 (1+ \epsilon^{{-}k_2}). \end{equation}

Since $BD\le 0$ on $(b,\,a)$, we have

\begin{align*} & \int_b^a EBD\,{\rm d}r \le \lambda \int_b^a M_3 \epsilon^{{-}k_1} (1+\rho)^{k_1(n-1)}r^{k_1} BD\,{\rm d}r\\ & \quad =\lambda M_3 \epsilon^{{-}k_1} \int_b^a(1+\rho)^{k_1(n-1)+2n-3}(n\rho +1)\rho'^2 r^{k_1+n+1}\,{\rm d}r\\ & \quad \le \frac{ b^{k_1+n+1}M_6}{\epsilon^{2n+k_1n}} \int_b^a (r-b)^{k_1(n-1)+2n-3}(r-a) \,{\rm d}r ={-}M_7\epsilon^{{-}k_1-1}. \end{align*}

This, combined with (3.13)–(3.15), proves that

\[ \lim_{\epsilon\to 0^+} \left (\epsilon^{k_2} \int_0^1 P_\epsilon (r)\,{\rm d}r\right ) \le \lim_{\epsilon\to 0^+} (M_5 -M_7 \epsilon^{k_2-k_1-1}) ={-}\infty. \]

Consequently, $\int _0^1 P_\epsilon (r)\,{\rm d}r<0$ if $\epsilon \in (0,\,a)$ is sufficiently small; this establishes (3.11).

4. The two-dimensional case

The rest of the paper is devoted to the study of certain exact and certain approximate Lipschitz solutions of Euler–Lagrange equation (2.1) in the 2-D case. Throughout this section, upon replacing $h(t)$ by $h(t)-h(0)$, we shall always assume $h\in C(\mathbb {R})$ and $h(0)=0.$

It is well-known [8, 13] that $u\in W^{1,\infty }_{loc}(\Omega ;\mathbb {R}^2)$ is a Lipschitz weak solution of equation (2.1) if and only if there exists a function $v\in W^{1,\infty }_{loc}(\Omega ;\mathbb {R}^2)$ such that the function $U=(u,\,v)\colon \Omega \to \mathbb {R}^4$ is a solution of the first-order partial differential relation:

(4.1)\begin{equation} DU(x)\in K = \left \{\begin{bmatrix} A\\ h(\det A) J A\end{bmatrix} : A\in \mathbb{M}^{2\times 2}\right\} \quad \mbox{a.e. in} \ \Omega, \end{equation}

where $J=\begin{bmatrix}0 & -1\\1 & 0\end{bmatrix};$ note that $\operatorname {cof} A=-JAJ$ for all $A\in \mathbb {M}^{2\times 2}.$ In general, if $U=(u,\,v)\in W^{1,1}_{loc}(\Omega ;\mathbb {R}^4)$ is a solution of relation (4.1), then we have $h(\det Du)\operatorname {cof} Du=-Dv J$ a.e. on $\Omega$ and thus $u$ is a weak solution of (2.1). Let $W=(u,\,-Jv)$ for $U=(u,\,v)\colon \Omega \to \mathbb {R}^4.$ Then relation (4.1) is equivalent to the relation:

(4.2)\begin{equation} DW(x)\in \mathcal{K} = \left \{\begin{bmatrix} A\\ h(\det A) A\end{bmatrix} : A\in \mathbb{M}^{2\times 2}\right\} \quad \mbox{a.e. in } \Omega. \end{equation}

We focus on certain exact and approximate Lipschitz solutions to relation (4.2). Recall that in studying a general partial differential relation of the form

(4.3)\begin{equation} D\phi(x)\in \mathcal{S} \quad \mbox{a.e. in } \Omega, \end{equation}

where $\Omega \subset \mathbb {R}^n$, $\phi \colon \Omega \to \mathbb {R}^m$ and $\mathcal {S}\subset \mathbb {M}^{m\times n},$ various semi-convex hulls of the set $\mathcal {S}$ play an important role; we refer to [4, 8] for the definitions and further properties of these semi-convex hulls. In particular, the quasiconvex hull $\mathcal {S}^{qc}$ of $\mathcal {S}$ can be equivalently defined as follows.

Definition 4.1 A matrix $\xi \in \mathbb {M}^{m\times n}$ belongs to $\mathcal {S}^{qc}$ if and only if there exists a uniformly bounded sequence $\{\phi _j\}$ in $W^{1,\infty }(\Omega ;\mathbb {R}^m)$ such that $\phi _j|_{\partial \Omega }=\xi x$ and

(4.4)\begin{equation} \lim_{j\to \infty}\int_\Omega \operatorname{dist}(D\phi_j,\mathcal{S})\,{\rm d}x=0, \end{equation}

where $\Omega$ is any fixed bounded Lipschitz domain in $\mathbb {R}^n$ and $\operatorname {dist}(\eta,\,\mathcal {S})$ is the distance from $\eta \in \mathbb {M}^{m\times n}$ to the set $\mathcal {S}.$

Remark 4.2

1. (i) If $\{\phi _j\}$ is uniformly bounded in $W^{1,\infty }(\Omega ;\mathbb {R}^m)$ and satisfies (4.4), then it is easily shown that $\int _\Omega H(D\phi _j)\,{\rm d}x\to 0$ as $j\to \infty$ for all nonnegative continuous functions $H$ on $\mathbb {M}^{m\times n}$ that vanish on $\mathcal {S}.$

2. (ii) Any sequence $\{\phi _j\}$ in $W^{1,1}(\Omega ;\mathbb {R}^m)$ satisfying (4.4) is called an approximate sequence of relation (4.3). We say that relation (4.3) is stable if the limit of every weakly* convergent approximate sequence in $W^{1,\infty }(\Omega ;\mathbb {R}^m)$ is a solution of the relation. We say that (4.3) is rigid if every weakly* convergent approximate sequence in $W^{1,\infty }(\Omega ;\mathbb {R}^m)$ converges strongly in $W_{loc}^{1,p}(\Omega ;\mathbb {R}^m)$ for all $1\le p<\infty.$

3. (iii) It is known that the stability of (4.3) is equivalent to the equality $\mathcal {S}^{qc}=\mathcal {S},$ while the rigidity involves much stronger conditions on the set $\mathcal {S}$ that cannot be generally given but can only be studied individually for a given problem.

4.1. Some algebraic restrictions on $\mathcal {K}^{qc}$

Let $\mathcal {K}$ be the set defined in (4.2). In the model case when $h(t)=t$, it has been proved in [8] that the rank-one convex hull $\mathcal {K}^{rc}=\mathcal {K}$; therefore, the set $\mathcal {K}$ does not support any open structures of $T_N$-configurations [8, 18], which makes the construction of counterexamples in [13, 16, 20] impossible by using such a set $\mathcal {K}.$

The following result gives some algebraic restrictions on $\mathcal {K}^{qc}$ for the set $\mathcal {K}.$

Proposition 4.3 Assume $h$ is one-to-one. Let $\begin{bmatrix}A\\ {B}\end{bmatrix}\in \mathcal {K}^{qc}.$ Then ${B=\mu h(\det A) A}$ for some $\mu >0;$ in particular, $B=0$ if $\det A=0.$

Proof. Let $\{u_n\}$ and $\{v_n\}$ be uniformly bounded in $W^{1,\infty }(\Omega ;\mathbb {R}^2)$ such that

(4.5)\begin{equation} u_n|_{\partial\Omega}=Ax, \quad v_n|_{\partial\Omega}=Bx, \quad \lim_{n\to \infty}\int_\Omega \operatorname{dist} \left (\begin{bmatrix} Du_n \\ Dv_n\end{bmatrix}, \mathcal{K} \right) \,{\rm d}x =0. \end{equation}

In what follows, for any two vectors $\chi ^1$ and $\chi ^2$ in $\mathbb {R}^2$, we define $\chi ^1\wedge \chi ^2=\det X,$ where $X$ is the matrix in $\mathbb {M}^{2\times 2}$ having $\chi ^1$ and $\chi ^2$ as its first and second rows. For $\eta \in \mathbb {M}^{4\times 2},$ let $\eta ^i\in \mathbb {R}^2$ be its $i$th row vector for $1\le i\le 4.$ Consider functions: $H_1(\eta )=|h(\eta ^1\wedge \eta ^2) (\eta ^1\wedge \eta ^2) -(\eta ^1\wedge \eta ^4)|$, $H_2(\eta )=|h(\eta ^1\wedge \eta ^2) (\eta ^1\wedge \eta ^2) -(\eta ^3\wedge \eta ^2)|$, and $H_3(\eta )=|\eta ^1\wedge \eta ^3|+| \eta ^2\wedge \eta ^4|;$ they are all nonnegative continuous and vanish on the set $\mathcal {K}.$ By (4.5) and remark 4.2(i), we have

(4.6)\begin{align} & \lim_{n\to \infty}\int_\Omega |h(\det Du_n) Du_n - Dv_n | \,{\rm d}x=0,\nonumber\\ & \lim_{n\to \infty}\int_\Omega |h(\alpha_n^1 \wedge \alpha_n^2)(\alpha_n^1\wedge \alpha_n^2) -\alpha_n^1\wedge \beta_n^2| \,{\rm d}x=0, \nonumber\\ & \lim_{n\to \infty}\int_\Omega |h(\alpha_n^1 \wedge \alpha_n^2)(\alpha_n^1\wedge \alpha_n^2) -\beta_n^1\wedge \alpha_n^2| \,{\rm d}x=0,\nonumber\\ & \lim_{n\to \infty}\int_\Omega (|\alpha_n^1\wedge \beta_n^1|+ |\alpha_n^2\wedge \beta_n^2|) \,{\rm d}x=0, \end{align}

where $\alpha _n^i$ and $\beta _n^i$ are the $i$th row of $Du_n$ and $Dv_n,$ respectively, for $i=1,\,2.$ Let $\alpha ^i$ and $\beta ^i$ be the $i$th row of $A$ and $B,$ respectively, for $i=1,\,2.$ Then, by the boundary conditions in (4.5) and the null-Lagrangian property of $2\times 2$ minors, we have $\int _\Omega \alpha _n^i\wedge \beta _n^k \,{\rm d}x = (\alpha ^i\wedge \beta ^k) |\Omega |$ for $i,\,k=1,\,2.$ Thus, it follows from (4.6) that

(4.7)\begin{equation} \alpha^1\wedge \beta^1 = \alpha^2\wedge \beta^2 =0, \quad \beta^1\wedge \alpha^2= \alpha ^1\wedge \beta ^2 = \lambda, \end{equation}

where

\[ \lambda = \lim_{n\to \infty} {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega h(\alpha_n^1\wedge \alpha_n^2) (\alpha_n^1\wedge \alpha_n^2) \,{\rm d}x. \]

Case 1: $\lambda =0.$ Since $h(0)=0$ and $h$ is one-to-one, we have that either $h(t)t >0$ for all $t\ne 0$ or $h(t)t <0$ for all $t\ne 0.$ In this case,

\[ \lim_{n\to \infty} {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega |h(\alpha_n^1\wedge \alpha_n^2)(\alpha_n^1\wedge \alpha_n^2)|\,{\rm d}x =|\lambda|=0. \]

Via a subsequence of $n\to \infty,$ we have $h(\alpha _n^1\wedge \alpha _n^2)(\alpha _n^1\wedge \alpha _n^2)\to 0;$ thus $\alpha _n^1\wedge \alpha _n^2=\det Du_n \to 0$ a.e. on $\Omega.$ Hence $\det A={1}/{|\Omega |}\int _\Omega \det Du_n \to 0$ and $\det A=0.$ Moreover, since $h(\det Du_n)\to 0$ a.e. on $\Omega,$ we have

\[ |B| = \lim_{n\to \infty} \Big | {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega Dv_n \,{\rm d}x \Big | \le \lim_{n\to \infty} {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega |h(\det Du_n) Du_n - Dv_n | \,{\rm d}x =0. \]

So, in this case, we have $\det A=0$, $B=0,$ and thus $B=\mu h(\det A) A$ for all $\mu >0.$

Case 2: $\lambda \ne 0.$ In this case, by (4.7), both $\alpha ^1$ and $\alpha ^2$ are nonzero, and we have $\beta ^1=t\alpha ^1$ and $\beta ^2=s\alpha ^2$ for some constants $t,\,s\in \mathbb {R};$ thus $\alpha ^1\wedge \beta ^2=\beta ^1\wedge \alpha ^2=t\alpha ^1\wedge \alpha ^2=s\alpha ^1\wedge \alpha ^2= \lambda \ne 0.$ It follows that $\det A=\alpha ^1\wedge \alpha ^2\ne 0$ and $t=s= {\lambda }/{\det A}.$ Therefore, in this case, we have $B=tA=\mu h(\det A) A,$ where $\mu = {\lambda }/{h(\det A)\det A}>0,\,$ because the signs of $h(t)t$ and $\lambda$ are the same for all $t\ne 0.$

Finally, from the two cases above, we see that $\det A=0$ if and only if $\lambda =0.$ Thus $B=0$ if $\det A=0.$

The quasiconvex hull $\mathcal {K}^{qc}$ would be completely determined if, for given $\det A\ne 0$ and positive $\mu \ne 1$, we know whether there exist sequences $\{ u_n\}$ and $\{ v_n\}$ uniformly bounded in $W^{1,\infty }(\Omega ;\mathbb {R}^2)$ such that

(4.8)\begin{equation} \begin{cases} \displaystyle{\lim_{n\to \infty} \int_\Omega | h(\det D u_n) D u_n - D v_n|\,{\rm d}x =0,}\\ u_n(x)|_{\partial\Omega}= Ax,\quad v_n(x)|_{\partial\Omega}=\mu h(\det A) Ax. \end{cases} \end{equation}

However, a complete answer to this question is at present out of reach. In the final two remaining subsections, we discuss some related partial results.

4.2. A related Dirichlet problem

Assume $h$ is one-to-one. Let $\det A\ne 0$ and $\mu >0.$ Closely related to the approximation problem (4.8), we study the exact Dirichlet problem:

(4.9)\begin{equation} \begin{cases} h(\det D u) Du=D v \quad a.e. \; \Omega,\\ u(x)|_{\partial\Omega}=Ax, \quad v(x)|_{\partial\Omega}=\mu h(\det A) Ax. \end{cases} \end{equation}

The main question is whether (4.9) has a Lipschitz solution for positive $\mu \ne 1.$

Remark 4.4 Let $\Omega$ be the unit open disc in $\mathbb {R}^2.$ Given any $\mu >1,$ let $\lambda >1$ be the unique number such that $h(\lambda )=\mu h(1).$ Define the radial functions $u(x)= \phi (|x|) x$ and $v(x)= h(\lambda ) u (x),$ where

\[ \phi(r)=\begin{cases} 0 & \mbox{if} \ 0\le r\le \sqrt{\frac{\lambda-1}{\lambda }},\\ \sqrt{\lambda -\frac{\lambda -1}{r^2}} & \mbox{if} \ \sqrt{\frac{\lambda -1}{\lambda }}\le r\le 1. \end{cases} \]

It follows from example 3.4 that $(u,\,v)\in C(\bar \Omega ;\mathbb {R}^4)\cap W^{1,p}(\Omega ;\mathbb {R}^4)$ for all $1\le p<2$ and is a solution of (4.9) with $A=I,$ but $(u,\,v)\notin W^{1,p}(\Omega ;\mathbb {R}^4)$ for any $p\ge 2.$

However, it remains open whether problem (4.9) has a Lipschitz or even a $W^{1,2}(\Omega ;\mathbb {R}^4)$ solution $(u,\,v)$ for some positive $\mu \ne 1.$

We have the following partial result.

Theorem 4.5 Assume $h$ is one-to-one and $\det A \ne 0.$ Let $\mu >0$ and suppose that $(u,\,v)\colon \bar \Omega \to \mathbb {R}^4$ is a Lipschitz solution to problem (4.9) such that $(\det A) {\det Du} \ge 0$ a.e. in $\Omega.$ Then, $\det Du=\det A$ a.e. on $\Omega$, $\mu =1$, and $v=h(\det A) u$ on $\bar \Omega.$

Proof. Write $g=h(\det Du).$ Since $gDu=Dv$, we have

\[ g^2\det Du=\det Dv,\quad 2g\det Du= Dv:\operatorname{cof} Du \quad a.e.\ \Omega. \]

Integrating over $\Omega$ and using the null-Lagrangian property of $2\times 2$ minors, we have

\begin{align*} {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega \det Du & =\det A,\quad {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega g \det Du =\mu h(\det A)\det A,\\ {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega g^2 \det Du & =(\mu h(\det A))^2 \det A, \end{align*}

and thus

\begin{align*} & \int_\Omega (g-\mu h(\det A))^2(\det A) {\det Du} \,{\rm d}x\\ & \quad =(\det A) \int_\Omega \Big ( g^2 \det Du -2\mu h(\det A) g\det Du +(\mu h(\det A))^2 \det Du\Big )\,{\rm d}x =0. \end{align*}

Since $(\det A) {\det Du} \ge 0$ a.e. in $\Omega,$ it follows that $g =h(\det Du)=\mu h(\det A)$ a.e. on the set $E=\{x\in \Omega : (\det A) \det Du(x)> 0\}.$ As $h$ is one-to-one, we have $\det Du=\lambda \chi _E$, where $\lambda \ne 0$ is the unique number such that $h(\lambda )=\mu h(\det A).$ Thus $Dv = h(\lambda ) (Du) \chi _E$ and $\det Dv=(h(\lambda ))^2\lambda \chi _E.$ Let $\tilde v (x)=Pv(x),$ where $P=\mbox {diag}(1,\,{1}/{(h(\lambda ))^2\lambda })$ is a diagonal matrix. Then $\tilde v$ is Lipschitz on $\Omega$, $D\tilde v = h(\lambda ) P(Du) \chi _E$, and $\det D\tilde v= \chi _E;$ thus,

\[ |D\tilde v(x)|^2\le L\det D\tilde v(x) \quad \mbox{ a.e.}\ \text{on} \ \Omega, \]

where $L= \|D\tilde v\|^2_{L^\infty (\Omega )}$. Since $\det Du=\lambda \chi _E$, we have $\lambda |E| = \int _\Omega \det Du =|\Omega |\det A \ne 0;$ thus $|E|>0.$ Hence $\tilde v$ is not constant on $\Omega.$ This proves that $\tilde v$ is a non-constant $L$-quasiregular map on $\Omega.$ It is well-known (see [12, 15]) that any non-constant $L$-quasiregular mapping cannot have its Jacobian determinant equal to zero on a set of positive measure; hence $|\Omega \setminus E|=0,$ which implies that $\det Du=\lambda,$ $h(\lambda ) Du=Dv$ a.e. on $\Omega,$ and $\lambda ={1}/{|\Omega |}\int _\Omega \det Du =\det A.$ Thus, from $h(\lambda )=\mu h(\det A),$ we have $\mu = 1.$ Finally, since $Dv=h(\lambda ) Du$ a.e. on $\Omega$ and $v= h(\lambda )u$ on $\partial \Omega,$ it follows that $v=h(\lambda )u$ on $\bar \Omega.$

4.3. Stability of certain restricted subsets of $\mathcal {K}$

We now study some restricted subsets of $\mathcal {K}$ that are more suitable for problems in nonlinear elasticity. For each $\epsilon >0,$ let

(4.10)\begin{equation} \mathcal{K}_\epsilon = \left \{\begin{bmatrix} A\\ h(\det A) A\end{bmatrix} : A\in \mathbb{M}^{2\times 2},\ \det A\ge \epsilon\right\}. \end{equation}

Theorem 4.6 Let $h$ be one-to-one. Then $\mathcal {K}_\epsilon ^{qc}=\mathcal {K}_\epsilon$ for all $\epsilon >0.$

Proof. Let $\begin{bmatrix} A\\ {B}\end{bmatrix} \in \mathcal {K}_\epsilon ^{qc}.$ Then there exist sequences $\{u_n\}$ and $\{v_n\}$ uniformly bounded in $W^{1,\infty }(\Omega ;\mathbb {R}^2)$ such that

(4.11)\begin{equation} u_n|_{\partial\Omega}=Ax, \quad v_n|_{\partial\Omega}=Bx, \quad \lim_{n\to \infty}\int_\Omega \operatorname{dist} \left(\begin{bmatrix} Du_n \\ Dv_n\end{bmatrix}, \mathcal{K}_\epsilon\right) \,{\rm d}x =0. \end{equation}

Since $\mathcal {K}_\epsilon$ is closed, it follows that there exist measurable functions $A_n\colon \Omega \to \mathbb {M}^{2\times 2}$ with $\det A_n(x)\ge \epsilon$ a.e. $x\in \Omega$ such that

\[ \operatorname{dist} \left(\begin{bmatrix} Du_n \\ Dv_n\end{bmatrix}, \mathcal{K}_\epsilon\right) =\left[|Du_n-A_n|^2 +|Dv_n-h(\det A_n)A_n|^2\right]^{1/2}. \]

It is easily seen that $\{A_n\}$ is uniformly bounded in $L^\infty (\Omega ; \mathbb {M}^{2\times 2}).$ Thus, we have

(4.12)\begin{align} & \det A=\lim_{n\to \infty} {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega \det Du_n\,{\rm d}x =\lim_{n\to \infty} {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega \det A_n\,{\rm d}x \ge \epsilon,\nonumber\\ & \lim_{n\to \infty}\int_\Omega |h(\det Du_n) Du_n - Dv_n | \,{\rm d}x=0. \end{align}

By proposition 4.3, we have $B=tA$ for some number $t.$ Note that ${1}/{|\Omega |}\int _\Omega \det Du_n =\det A$ and, as in the proof of proposition 4.3,

\[ \lim_{n\to \infty} {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega h(\det Du_n) \det Du_n \,{\rm d}x = t\det A; \]

furthermore,

\[ \lim_{n\to \infty} {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega (h(\det Du_n))^2\det Du_n \,{\rm d}x = \lim_{n\to \infty} {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega \det Dv_n \,{\rm d}x =t^2 \det A. \]

Hence

\[ \lim_{n\to \infty} {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega (h(\det Du_n) -t)^2\det A_n \,{\rm d}x = \lim_{n\to \infty} {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega (h(\det Du_n) -t)^2\det Du_n \,{\rm d}x =0. \]

Since $\det A_n\ge \epsilon,$ we obtain

\[ \lim_{n\to \infty} {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega (h(\det Du_n) -t)^2 \,{\rm d}x =0, \]

Via a subsequence $n_j\to \infty$, we have $h(\det Du_{n_j}(x))\to t$ for a.e. $x\in \Omega.$ Since $h$ is continuous and one-to-one, this implies $\det Du_{n_j}(x)\to h^{-1}(t)$ for a.e. $x\in \Omega.$ Thus,

\[ \det A= {\int \hspace{-11pt} - \hspace{-1pt}}_\Omega \det Du_{n_j}(x)\,{\rm d}x \to h^{{-}1}(t) \]

and $t=h(\det A).$ This proves $B=h(\det A)A;$ thus $\begin{bmatrix} A\\ {B}\end{bmatrix} \in \mathcal {K}_\epsilon,$ as $\det A\ge \epsilon.$

Proposition 4.7 Let $h$ be one-to-one. Suppose that sequences $\{u_n\}$ and $\{v_n\}$ converge weakly* to $\bar u$ and $\bar v$ in $W^{1,\infty }(\Omega ;\mathbb {R}^2)$ as $n\to \infty,$ respectively, and satisfy

(4.13)\begin{equation} \lim_{n\to \infty}\int_\Omega \operatorname{dist} \left(\begin{bmatrix} Du_n \\ Dv_n\end{bmatrix}, \mathcal{K}_\epsilon\right) \,{\rm d}x =0. \end{equation}

Then $\begin{bmatrix} D\bar u (x) \\ D\bar v(x)\end{bmatrix} \in \mathcal {K}_\epsilon$ for a.e. $x\in \Omega ;$ moreover, $\det Du_n \to \det D\bar u$ strongly in $L^p(\Omega )$ for all $1\le p<\infty.$

Proof. Note that the stated inclusion follows from theorem 4.6 by a general theorem on quasiconvex hulls; however, we give a direct proof without using such a result. As in the proof of theorem 4.6, let $\{A_n\}$ be a uniformly bounded sequence in $L^\infty (\Omega ; \mathbb {M}^{2\times 2})$ with $\det A_n(x)\ge \epsilon$ for a.e. $x\in \Omega$ such that

(4.14)\begin{equation} \lim_{n\to \infty}\int_\Omega \left[|Du_n-A_n|^2 +|Dv_n-h(\det A_n)A_n|^2\right]^{1/2} {\rm d}x =0. \end{equation}

Thus, for all measurable sets $E\subset \Omega,$

\[ \int_E\det D\bar u \,{\rm d}x =\lim_{n\to\infty}\int_E \det Du_n\,{\rm d}x =\lim_{n\to\infty}\int_E \det A_n\,{\rm d}x \ge \epsilon |E|. \]

From this it follows that

\[ \det D\bar u(x)\ge \epsilon \quad \mbox{a.e.}\ x\in \Omega. \]

As before, let $\alpha ^i$ and $\beta ^i$ be the $i$th row of $D\bar u$ and $D\bar v,$ respectively, and let $\alpha _n^i$ and $\beta _n^i$ be the $i$th row of $Du_n$ and $Dv_n,$ respectively, for $i=1,\,2.$ Then all the limits listed in (4.6) above still hold. By the weak* convergence of minors, for all measurable sets $E\subset \Omega,$ we have

\[ \Big |\int_E \alpha^1\wedge \beta^1 \Big |=\Big | \lim_{n\to\infty}\int_E \alpha_n^1\wedge \beta_n^1 \Big |\le \lim_{n\to\infty}\int_\Omega |\alpha_n^1\wedge \beta_n^1| =0, \]

and similarly,

\begin{align*} \Big |\int_E \alpha^2\wedge \beta^2 \Big | & \le \lim_{n\to\infty}\int_\Omega |\alpha_n^2\wedge \beta_n^2| =0,\\ \Big |\int_E (\alpha^1\wedge \beta^2-\beta^1\wedge \alpha^2) \Big | & \le \lim_{n\to\infty}\int_\Omega |\alpha_n^1\wedge \beta_n^2-\beta_n^1\wedge \alpha_n^2| =0. \end{align*}

So $\alpha ^1\wedge \beta ^1=\alpha ^2\wedge \beta ^2=0$ and $\alpha ^1\wedge \beta ^2=\beta ^1\wedge \alpha ^2$ a.e. on $\Omega.$ Since $\alpha ^1\wedge \alpha ^2=\det D\bar u \ge \epsilon,$ it follows that $\beta ^1=g\alpha ^1$ and $\beta ^2=g\alpha ^2,$ where $g={\alpha ^1\wedge \beta ^2}/{\alpha ^1\wedge \alpha ^2}\in L^\infty (\Omega ).$ Again by (4.6) and the weak* convergence of $2\times 2$ minors, we have

\begin{align*} \lim_{n\to \infty}\int_\Omega g^2 \det Du_n\,{\rm d}x& =\int_\Omega g^2 \det D\bar u\,{\rm d}x,\\ \lim_{n\to \infty}\int_\Omega g h(\det Du_n) \det Du_n \,{\rm d}x & = \lim_{n\to \infty}\int_\Omega g \beta_n^1\wedge \alpha_n^2 \,{\rm d}x = \int_\Omega g^2\det D\bar u\,{\rm d}x,\\ \lim_{n\to \infty}\int_\Omega (h(\det Du_n))^2\det Du_n \,{\rm d}x & = \lim_{n\to \infty}\int_\Omega \det Dv_n \,{\rm d}x = \int_\Omega g^2\det D\bar u\,{\rm d}x. \end{align*}

Therefore,

\[ \lim_{n\to \infty}\int_\Omega (h(\det Du_n) -g)^2\det A_n \,{\rm d}x = \lim_{n\to \infty}\int_\Omega (h(\det Du_n) -g)^2\det Du_n \,{\rm d}x =0. \]

Since $\det A_n\ge \epsilon$, it follows that $h(\det Du_n) \to g$ strongly in $L^2(\Omega ).$ We assume, along a subsequence $n_j\to \infty,$ that $h(\det Du_{n_j}(x))\to g(x)$ for a.e. $x\in \Omega.$ Since $h$ is continuous and one-to-one, we have $\det Du_{n_j}(x)\to h^{-1}(g(x))$ for a.e. $x\in \Omega.$ But, since $\det Du_n\rightharpoonup \det D\bar u$ weakly* in $L^\infty (\Omega ),$ it follows that $h^{-1}(g(x))=\det D\bar u(x)$ a.e. $x\in \Omega,$ which proves $g=h(\det D\bar u)$ and hence $D\bar v= h(\det D\bar u)D\bar u.$ Finally, the strong convergence of $h(\det Du_n) \to h(\det D\bar u)$ in $L^2(\Omega )$ and the weak* convergence of $\{u_n\}$ and $\{v_n\}$ in $W^{1,\infty }(\Omega ;\mathbb {R}^2)$ establish the strong convergence of $\det Du_n\to \det D\bar u$ in $L^p(\Omega )$ for all $1\le p<\infty.$