Given an integer $k\ge 2$, let $\omega _k(n)$ denote the number of primes that divide n with multiplicity exactly k. We compute the density $e_{k,m}$ of those integers n for which $\omega _k(n)=m$ for every integer $m\ge 0$. We also show that the generating function $\sum _{m=0}^\infty e_{k,m}z^m$ is an entire function that can be written in the form $\prod _{p} \bigl (1+{(p-1)(z-1)}/{p^{k+1}} \bigr )$; from this representation we show how to both numerically calculate the $e_{k,m}$ to high precision and provide an asymptotic upper bound for the $e_{k,m}$. We further show how to generalize these results to all additive functions of the form $\sum _{j=2}^\infty a_j \omega _j(n)$; when $a_j=j-1$ this recovers a classical result of Rényi on the distribution of $\Omega (n)-\omega (n)$.

In this work we analyse bucket increasing tree families. We introduce two simple stochastic growth processes, generating random bucket increasing trees of size n, complementing the earlier result of Mahmoud and Smythe (1995, Theoret. Comput. Sci.144 221–249.) for bucket recursive trees. On the combinatorial side, we define multilabelled generalisations of the tree families d-ary increasing trees and generalised plane-oriented recursive trees. Additionally, we introduce a clustering process for ordinary increasing trees and relate it to bucket increasing trees. We discuss in detail the bucket size two and present a bijection between such bucket increasing tree families and certain families of graphs called increasing diamonds, providing an explanation for phenomena observed by Bodini et al. (2016, Lect. Notes Comput. Sci.9644 207–219.). Concerning structural properties of bucket increasing trees, we analyse the tree parameter $K_n$ . It counts the initial bucket size of the node containing label n in a tree of size n and is closely related to the distribution of node types. Additionally, we analyse the parameters descendants of label j and degree of the bucket containing label j, providing distributional decompositions, complementing and extending earlier results (Kuba and Panholzer (2010), Theoret. Comput. Sci.411(34–36) 3255–3273.).

The asymptotic behaviour of an occupation-time process associated with alternating renewal processes is investigated in the infinite mean cycle case. The limit theorems obtained extend some asymptotic results proved by Dynkin (1955), Lamperti (1958) and Erickson (1970) for the classical spent lifetime process. Some new phenomena are also presented.

A class of non-negative alternating regenerative processes is considered, where the process stays at zero random time (waiting period), then it jumps to a random positive level and hits zero after some random period (life period), depending on the evolution of the process. It is assumed that the waiting time and the lifetime belong to the domain of attraction of stable laws with parameters in the interval (½,1]. An integral representation for the distribution functions of the regenerative process is obtained, using the spent time distributions of the corresponding alternating renewal process. Given the asymptotic behaviour of the process in the regenerative cycle, different types of limiting distributions are proved, applying some new results for the corresponding renewal process and two limit theorems for the convergence in distribution.

This paper considers the joint limiting behavior of sums and maxima of stationary discrete-valued processes. The asymptotic behavior is a cross between a central limit theorem and asymptotic bounds for the distribution of the maxima. Some applications and simulations are also included.

For a random mapping model with a single attracting center (Stepanov (1971)) we study the relationship between the sizes of the central tree, the adjacent points, and the free points. Joint, marginal and conditional distributions are shown to be of well-known Lagrangian type. Exact and asymptotic moment properties are investigated with the aid of Riordan's (1968) Abel identities. In particular, the relationship between the size of the central tree and that of the central component is discussed in terms of a regression function.

The familiar limiting distributions of excess, age, and spread are derived on sample paths, where these distributions are fractions of time. Similar results are obtained for the distribution of remaining service at a queue. For stochastic processes, where specified limits exist as finite constants with probability 1, the derived sample-path results are shown to imply that the same limiting distributions hold, where in a stochastic setting, these distributions may be defined in a more conventional way.

We consider the random vector T = (T(0), ···, T(n)) with independent identically distributed coordinates such that Pr{T(i) = j} = Pj, j = 0, 1, ···, n, Σ 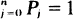. A realization of T can be viewed as a random graph GT with vertices {0, ···, n} and arcs {(0, T(0)), ···, (n, T(n))}. For each T we partition the vertex-set of GT into three disjoint groups and study the joint probability distribution of their cardinalities. Assuming that 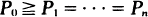we observe the asymptotics of this distribution, as n → ∞, for all possible values of P0. It turns out that in some cases these cardinalities are asymptotically independent and identically distributed.

In Part I (Hunter) a study of feedback queueing models was initiated. For such models the queue-length process embedded at all transition points was formulated as a Markov renewal process (MRP). This led to the observation that the queue-length processes embedded at any of the ‘arrival', ‘departure', ‘feedback', ‘input', ‘output' or ‘external' transition epochs are also MRP. Part I concentrated on the properties of the embedded discrete-time Markov chains. In this part we examine the semi-Markov processes associated with each of these embedded MRP and derive expressions for the stationary distributions associated with their irreducible subspaces. The special cases of birth-death queues with instantaneous state-dependent feedback, M/M/1/N and M/M/1 queues with instantaneous Bernoulli feedback are considered in detail. The results obtained complement those derived in Part II (Hunter) for birth-death queues without feedback.

Queueing systems which can be formulated as Markov renewal processes with basic transitions of three types, ‘arrivals', ‘departures' and ‘feedbacks' are examined. The filtering procedure developed for Markov renewal processes by Çinlar (1969) is applied to such queueing models to show that the queue-length processes embedded at any of the ‘arrival', ‘departure', ‘feedback', ‘input', ‘output' or ‘external' transition epochs are also Markov renewal. In this part we focus attention on the derivation of stationary and limiting distributions (when they exist) for each of the embedded discrete-time processes, the embedded Markov chains. These results are applied to birth–death queues with instantaneous state-dependent feedback including the special cases of M/M/1/N and M/M/1 queues with instantaneous Bernoulli feedback.

In this part we extend and particularise results developed by the author in Part I (pp. 349–375) for a class of queueing systems which can be formulated as Markov renewal processes. We examine those models where the basic transition consists of only two types: ‘arrivals' and ‘departures'. The ‘arrival lobby' and ‘departure lobby' queue-length processes are shown, using the results of Part I to be Markov renewal. Whereas the initial study focused attention on the behaviour of the embedded discrete-time Markov chains, in this paper we examine, in detail, the embedded continuous-time semi-Markov processes. The limiting distributions of the queue-length processes in both continuous and discrete time are derived and interrelationships between them are examined in the case of continuous-time birth–death queues including the M/M/1/M and M/M/1 variants. Results for discrete-time birth–death queues are also derived.

A random mapping of a countable set N into itself is studied. An explicit formula is found for the probability that a subset of N will not contain cycles of the mapping. This formula is applied then for a detailed asymptotic analysis of two special mappings.

A simple epidemic process in which the number of individuals who can become infected at any point in time is itself a random variable is described. The discrete asymptotic behaviour of such a process is discussed. In particular, the associated marginal distribution of the limiting process is considered.

For the general age-dependent branching process subject to an arbitrary pattern of immigration asymptotic distributions are studied in the supercritical and critical cases. In the supercritical case a new functional form is given for the limiting cumulant-generating function and in the critical case moment conditions are given under which a randomized gamma limit law prevails. The age-structure is also considered.

Some necessary and sufficient conditions are found for the existence of a proper non-degenerate limiting distribution for a Bellman-Harris age-dependent branching process with a compound renewal immigration component. A number of these results are applicable to the batch arrival GI/G/∞ queueing process. Some aspects of the situation when there is no such limiting distribution are considered. The situation when the immigration component is a nonhomogeneous compound Poisson process is briefly considered.

The limiting distributions of the actual waiting time and the virtual waiting time are determined for a single-server queue with Poisson input and general service times in the case where there are two types of services and no customer can stay in the system longer than an interval of length m.